Over the years, especially in PBL with mathematics, I have found that students greatly appreciate the authorship and ownership that comes with keeping a journal in my classroom.  In fact when I asked my students earlier this year, “When do you feel most confident in this class?” and here are some of the feedback responses they gave me:

“When I am about to hand in a journal.”
“When I am writing a journal entry because there are various concepts that initially don’t understand, and after discussions I make big discoveries and therefore it makes writing about it easier for me.”

There is something that I have come to appreciate about the way students grow to be able to show how they understand a concept.  Recently, I read a student’s journal entry and thought it was so amazing that I asked him if I could blog about it.  I thought that it really showed how he moved through his understanding of the concept and how he struggled with it to the end. In fact, he presented this problem in class thinking that he had gotten it right and wonderfully, kept going and learned something in the process.

The student – let’s call him Pete- was dealing with a problem that was towards the end of a thread that dealt with the concept of distance – distance between two points, distance between a point and a line, distance between two lines, etc.  This question was asking students to think about two different types of distance.  Here’s the problem:

“Plot all of the points that are 3 units away from the x axis and write an algebraic expression for those points.  Then plot all the points that are 3 units away from the point (5,4) and write an algebraic expression for those points.”

Up until now all we had discussed was writing expressions for Pythagorean distance between two points and writing equations for equations of lines.  We had also talked about the fact that the closest distance from a point to a line is the perpendicular distance.  So Pete was easily able to answer the first part of the question seeing that the set of all points that were 3 units away from the x axis were both the line y=3 and the line y=-3.  He drew a diagram discussing his concept of distance from a point to a line and how he visually (in his mind and physically on paper and at the board that day in class – connected them together).

However, in his journal he wrote about how the second part of the question seemed just as easy to him at first.  “I assumed I needed to do a straight line. I then saw ‘three units’, so I put a point on (5,1), and drew the line y=1.  If (5,1) was 3 away, I thought, shouldn’t all the points on the line be 3 away?” Here’s what his first diagram looked like:

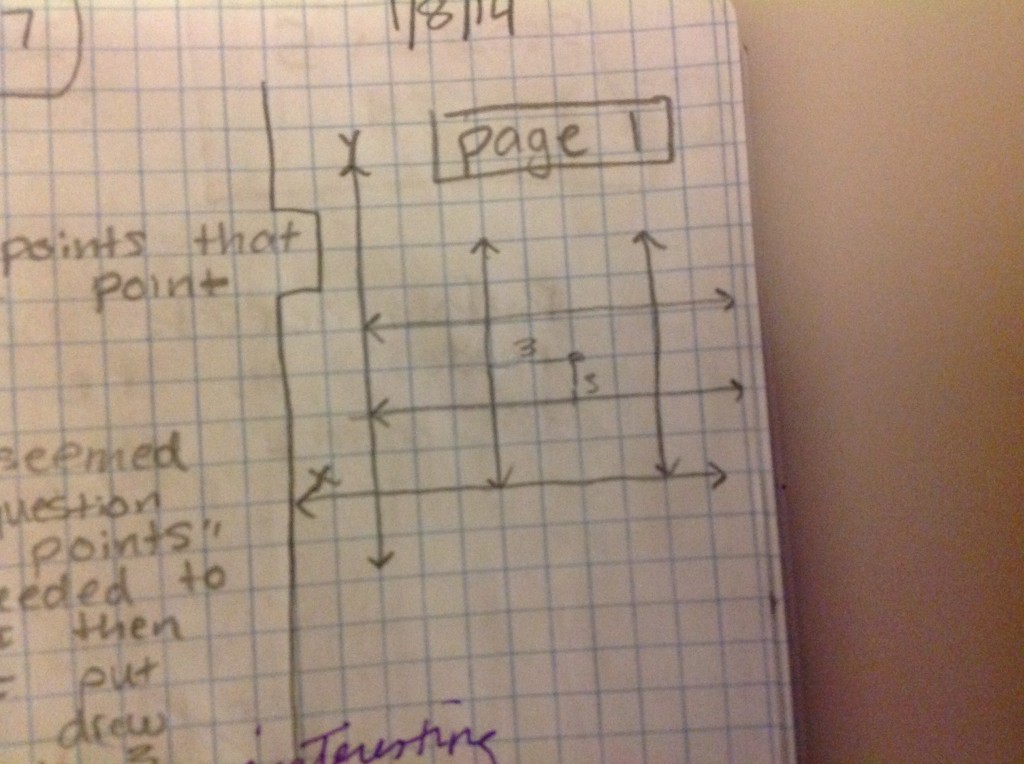
Pete had tried to use his understanding of distance being “three units” away from a point in the same way that being “three units” away from a line in the previous problem.  However, when he was at the board, another student told him they had thought of it another way and shared with Pete something Pete realized very soon…. “Only 1 point on each of the lines was actually 3.  The rest of the points were actually all further than 3 units from the point.”  Here’s his diagram of his realization of that:

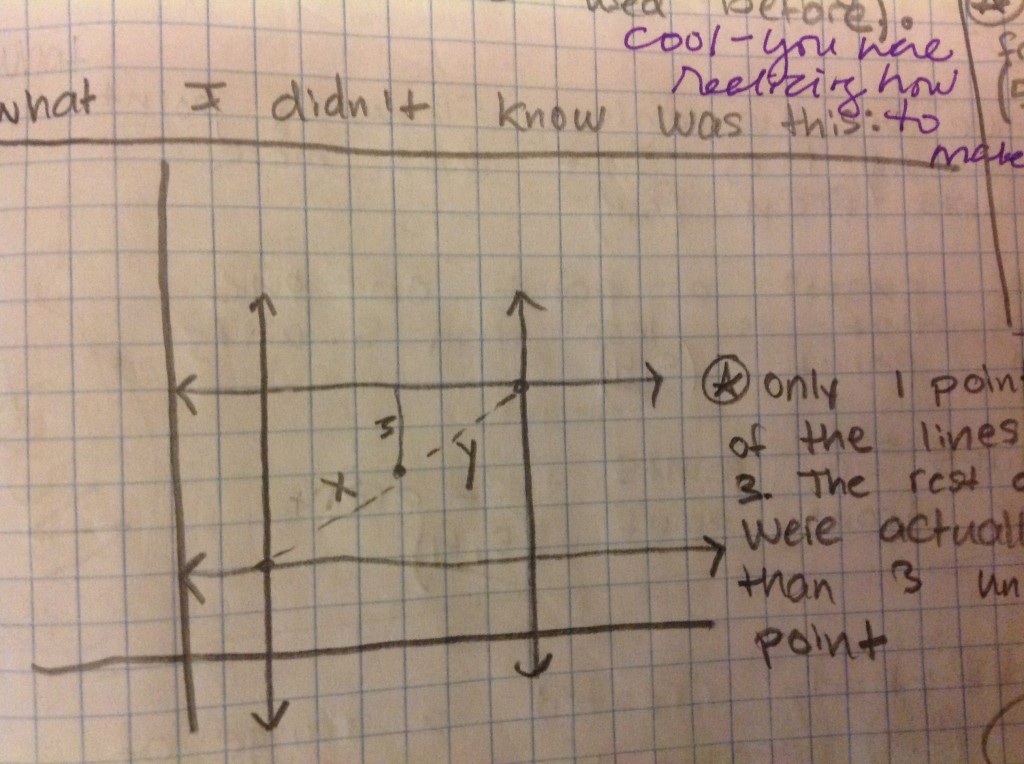
So now Pete is discussing how he is using his knowledge of Pythagorean distance and seeing that only the vertical and horizontal points are actually the required 3 units away.  Huh, how does he move forward now?  At the moment when he was in class, it took another classmate to say that ALL the points were supposed to be 3 units away and that he (that classmate) thought it would look more like a circle.  Pete was still determined to correct his own work (which I just love) then attempted this drawing:

 

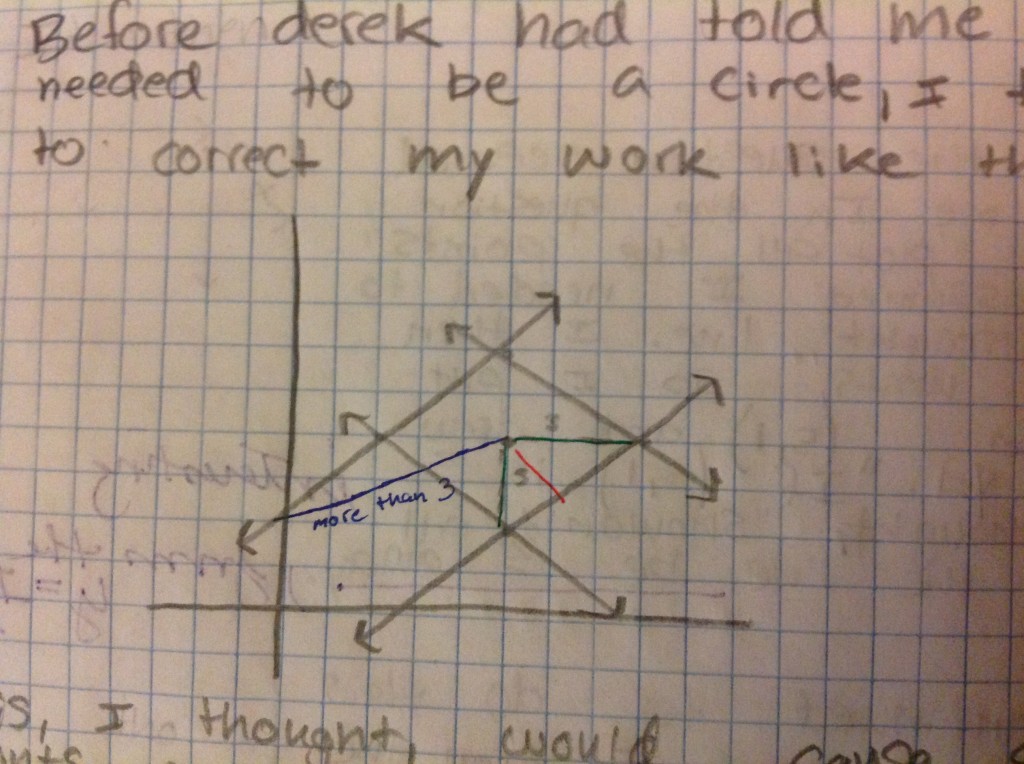
Pete writes:

“This, I thought, would cause all points on the line to be 3 units away from point (5,4).  However, I was again wrong.  The blue line on the diagram shows a point on one of my lines that was more than 3 units from (5,4). The red line shows a point on one of the lines that is less than three units from (5,4) [it would’ve been great if he went into more detail, but at this point I’m so psyched that he’s going into this much detail!]  The green lines are points that are 3 units away from point (5,4).  I have effectively created a range of lengths from (5,4) opposed to what the question was asking for which was 3 units from (5,4).”

This is some of the most insightful journal writing I’ve ever seen from a high school student.  Pete is moving through his understanding of what it means for a point to be 3 units away from another point (even when another student has imposed their understanding on him) and is trying to show me how he came to understand his classmate’s argument that it is a circle.  Realizing that there are points that are more than 3 units away (farther out than this diamond shape), 3 away (at the vertices) and closer than 3 (along the sides of the diamond)….well, that there was a range and not all constantly 3, showed him that his ideas was not correct.

Pete then draws this diagram:

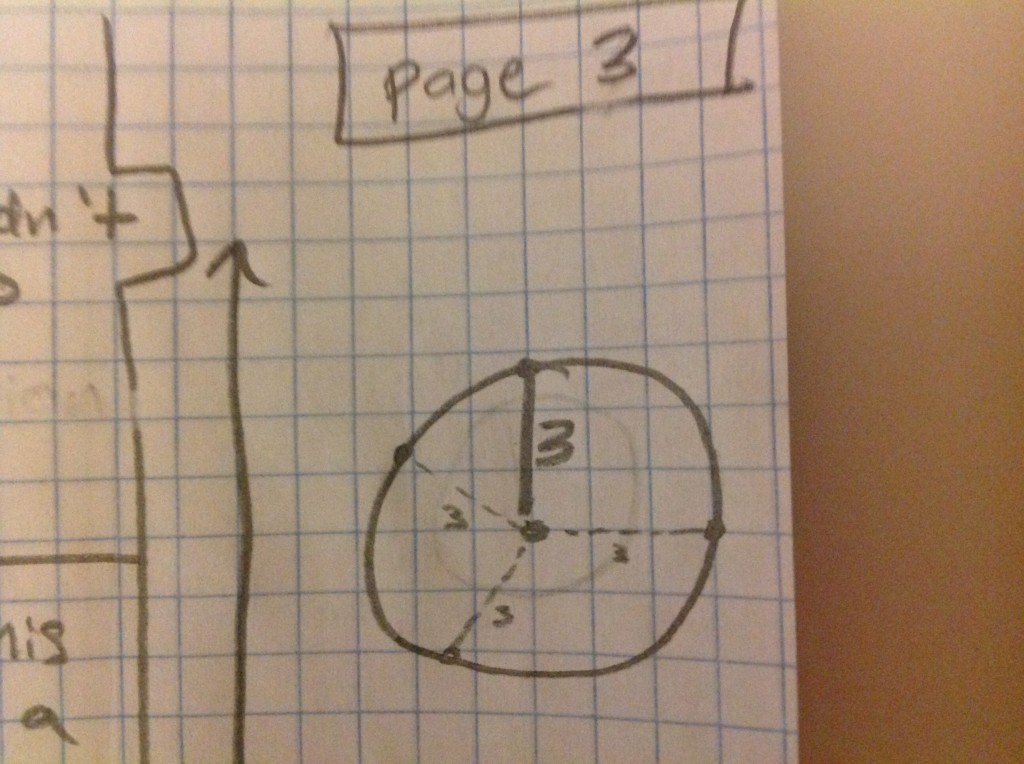
After understanding this, Pete writes:

“It made perfect sense!…Any point from the centerpoint of a circle to any point on the circle was the same length (the radius).  I immediately drew the connection.  3 was the radius and (5,4) was the center.  the distance between the middle point and any point on the circle was 3!”

Although Pete didn’t write about the discussion that ensued about the algebraic expression, I still felt like the goals of metacognitive journaling were reached with this entry.  Pete has articulated why he chose this problem to me, he started with his misunderstanding, how another student or the class discussion/experience had helped move him through his understanding and he could clearly write about how he now has a good understanding of the mathematical concept.  I was so proud of Pete.  Yes, it’s true, not every student gets to this point of writing mathematically by January of the school year, but the growth that occurs in each individual student is what is important – not necessarily the level of maturity in the writing.  However, I have learned not to prejudge or dismiss any student who starts off at a lower level because I believe they are all capable of growth.

If you are interested in looking at my grading rubric for journals or asking me questions about how I use them in the course, don’t hesitate to get in touch. (If you are looking for the grading rubric, make sure you scroll to the third page of that pdf).