Tag: Relational Pedagogy

Let’s Keep Online Learning Relational

About three years ago, I was invited to the great challenge of finding a way to teach Problem-Based Math online with a great school named Avenues: The World School, whose online school is called Avenues Online (AON). Little did I know then that COVID-19 would come in 2020 and the idea of online learning would be not only popular but necessary in this time of crisis.

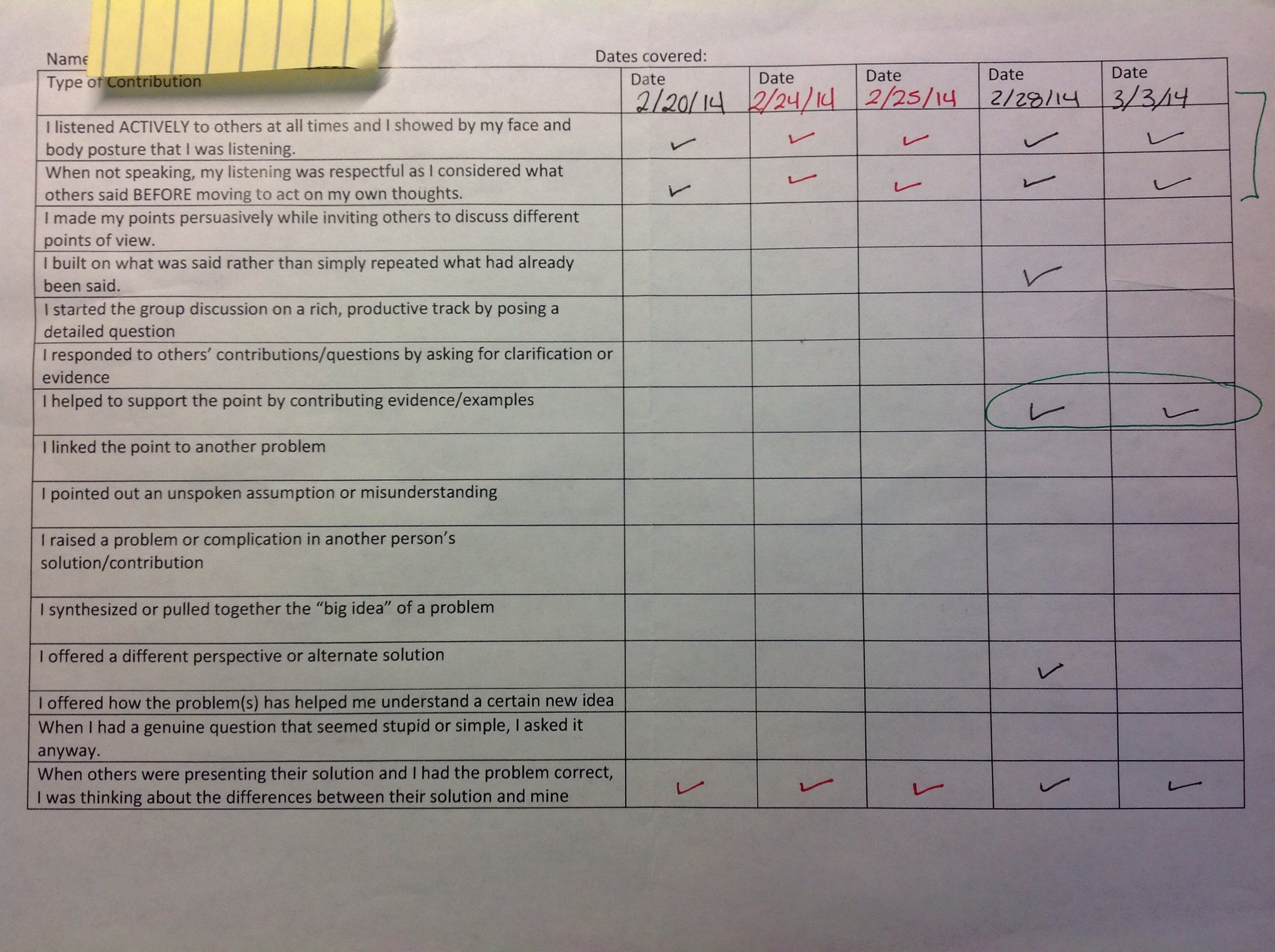
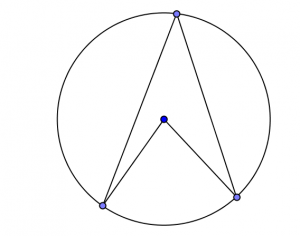
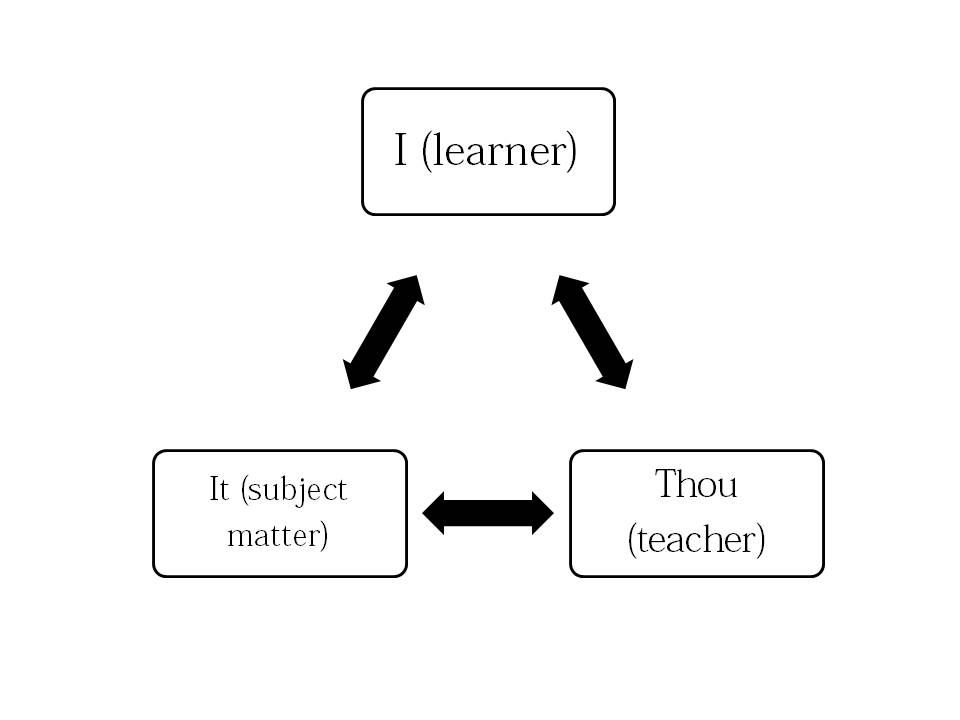
Awhile ago, I wrote a blogpost about teaching with a relational pedagogy with technology, as it is something that has been on my mind for a long time. When we started the pilot of AON, one of my first challenges was to find a way for students to interact that somehow mimicked the classroom process and community of discourse and interaction that a Relational PBL classroom has. In the past, I had experimented with the wonderful app called Voicethread that allowed students to have threaded discussions about problems with video, audio, text and drawing. I had students try to have conversations before we came to class about some problems they were grappling with. Here is an example of one of those voicethreads:

Of course, this was always followed by a class the next day, so we could follow up on students’s questions and comments. After I did this small experiment, I did a survey of the students in my classes:

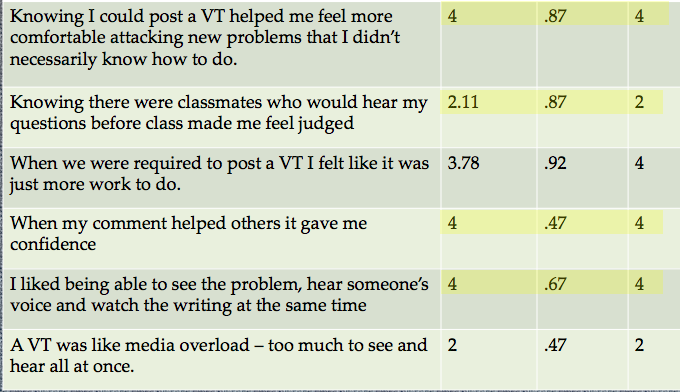
I think what I learned from this experiment was that using an app or interface that allowed for some type of interaction where students could hear, see and interact with each other helped them feel more heard, not judged, and more confident in their learning and discussion. They also did not feel like it was media overload, which was not surprising for this generation for sure.

This experiment helped inform the work I did with AON of course. There was no work that was F2F at all since this school is totally online. The coursework for other disciplines were split into World and STEAM, which are totally project-based (to learn more click the AON link above). But we kept math separate, in what we called the Math Inquiry Program, in order to make sure that the materials were consistently covered in a problem-based way.

The next question is, how do we get students in an online environment to have those relational interactions that were important to the students that used Voicethread? Clearly, it was seeing, hearing, interacting and being able to read each other’s work.

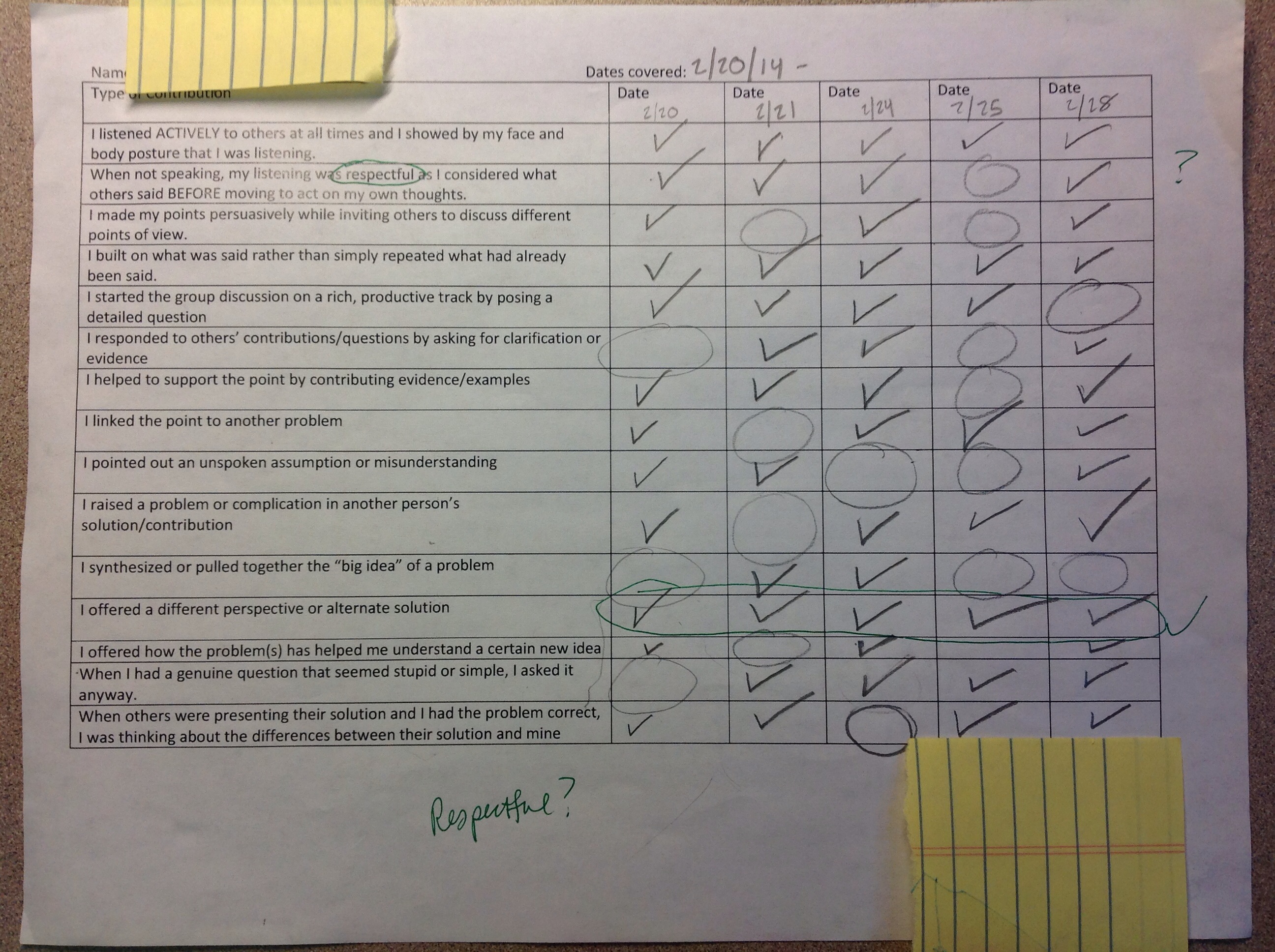
My ideas came to fruition with the amazing help of some exceptionally talented developers in our R&D team. We are lucky to have an interface now that allows for students to see, hear, write and watch each other write while discussing problems. The interface looks like this:

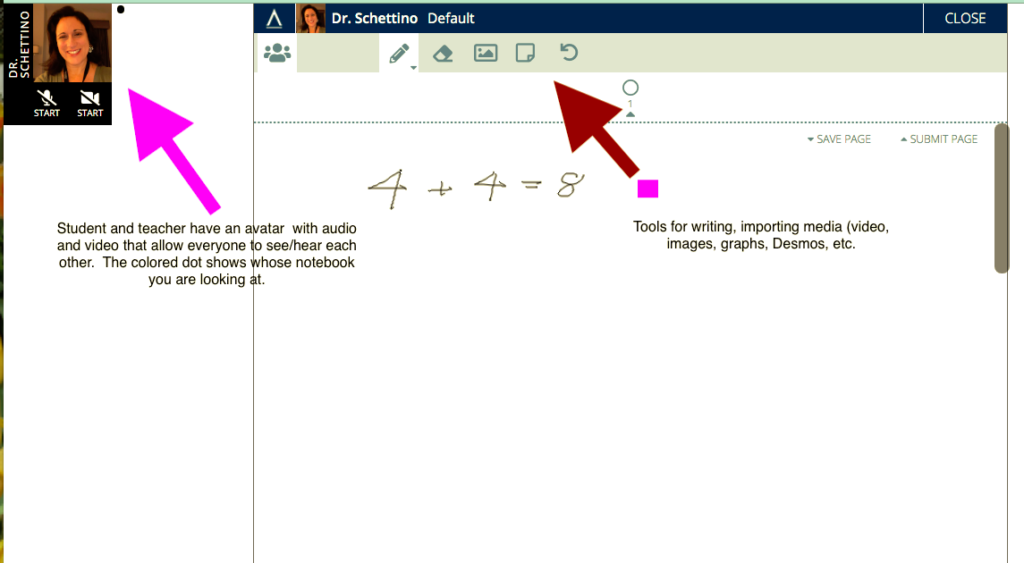
Each person in the class (cohort) as a “notebook” that shows up on a tab.

We have it to be very effective for interaction between students and teachers and to allow for collaborative problem solving. Is it perfect? Not quite, there is much that needs to be done, including teacher training and improvement in bandwidth needed for the video, audio and writing capabilities.

Here is a 5-minute video of a small cohort of 7th graders discussing a Mathalicious problem that you may be familiar with. It shows some of the ability of the interface and the ways in which students and teachers can interact in real-time.

The most important piece of teaching online with PBL is attempting to keep the relationships together – student-student, student-teacher and student-material. Keeping students involved in metcognitive activities – writing, making videos about their ideas, interacting with each other – this is what will pay off in the long run. It’s not something we are going to create in the next month or so, but if we keep in mind the relational aspects of learning, we may come out of this crisis with the strong connections we always had with our students.

Handouts – Front and Center

I always try to make it easy for people to find both my slides and handouts when I give a talk – so Here’s my powerpoint presentation from my talk entitled, “Change the Classroom, Not the Students: Creating Equity with PBL”  which I’m giving today at the NCTM Annual Conference in New Orleans – great to be here.  I also have 2 handouts which include my framework for a relational PBL class and the results of my qualitative dissertation – I’d love to hear any comments and questions and start a discussion with PBL teachers. (I do not include the videos I used in this public version of the powerpoint, sorry)

[slideshare id=33364598&doc=changetheclassroomschettino-140410070229-phpapp02]

Schettino Framework Handout

Schettino Sample Problems Handout NCTM2014

There are actually a few talks here today that I would highly recommend and seem to be related to this topic of creating a classroom that allows for discussion and interaction at the level of creating equity.  One of them is on Friday, and is entitled “The Hidden Message: Micromessaging and Mathematics” and it seems to be about managing the way we talk to each other in the classroom and making sure all voices are heard.  I’m definitely going to that one!  Unfortunately, Jo Boaler is presenting at the exact same time as me!  I don’t know if I should take that as a compliment that I was put as the same time or not 🙁

Well, hope everyone has a great time!  Enjoy the conference!

Having Students Be More Aware of their Contributions in PBL

One of the things I do at the middle of the term to have students reflect on the way that they talk about mathematics in class, is have them evaluate their work with my Student Self-Report on Class Contribution and I give them detailed feedback on their rankings of each type of question and what I think about their work so far.  But what I’ve found in the past is that it’s hard for them to remember specific examples of when they “helped to support the point by contributing evidence” or “raised a problem in another person’s solution” weeks or days after the fact.  Students have so much to think about during a class period that it really needs to be “in the moment” for them to be deliberately thinking about what they are doing and saying to each other.

So I had this idea that I would actually have them keep track of the types of questions and comments they made to each other over a five day period at the end of the term and then hand it in to me.  This way they would check off each category of question or comment at the moment when they did it.  So I made copies of this table of Student Analysis of Contribution and had them keep it on their desks while we were discussing topics or problems in class.  They had to have something to write with while they were talking and taking notes too.

Initially the students were very concerned about how they were going to tell the difference between each of the types of contributions.  But little by little, it became easier.  These categories were not arbitrary, they happened all the time, they just hadn’t really thought about it before.   For example, when a student presented a problem but had made an error and didn’t know it, another student usually “raised a complication in another person’s soltuion” or “pointed out an unspoken assumption or misunderstanding.”  These are important contributions that they were making every day about mathematics and are important critical thinking skills they didn’t even realize they were developing.  I just wanted to help them be more specific about realizing it.

The first day we did this I heard some students say something like, “Ooh, I just…(brief pause while looking at the table)… ‘started the group discussion on a rich, productive track by posing a detailed question.” with pride and excitement (and maybe a little sarcasm).  But after three days of doing it, and students seeing that they had places that they could check off, one student actually said, “I think that this table makes our conversations more interesting.”

Here are some samples of student feedback:

Quiet student who needs to work on all types of comments and questions

This first respondent is a student is very quiet, and she knows it.  She rarely speaks in class, but is actively listening.  I’ve spoken to her about why she does not ask questions or share her ideas in class and she says that she is afraid of being wrong in front of everyone.  My hope all year has been that seeing everyone else be wrong regularly would eventually show her how acceptable it was in class.  It was clear that using this table showed her that there are many different ways to contribute to class discussion.  When she presented her problems in class (which she will do when asked) she is very capable and knows that she is contributing evidence or examples, but she rarely questions others’ work.  I do remember the time when she “built on” what was said by asking a question about someone else’s solution.  She also sometimes asks for clarification of her own understanding, but I know that she’s capable of more.  I struggle with how to encourage her to get more out of class discussion, but at least now she knows how important a role she can play.

Outgoing student who lacks interaction from other kids

This is a really interesting student who is quite inquisitive and very comfortable with sharing his ideas.  When he has a question or comment, it’s very easy to get him to go to the board and naturally start writing another idea or possible solution method.  However, I noticed was missing in his table were checks in the two rows that had to do with “inviting others” into his thought process and also making comments on others’ solutions and ideas.  It made me wonder how much time in class he spends listening to other students talking or if he is just listening to his own ideas in his own mind.  I am working on trying to get him to collaborate more – with his creative mind it would behoove him to start interacting more with others.

Outgoing student who is unaware of his effect on others

This table belongs to a student who really has overestimated his contributions to class.  He definitely spends a lot of time talking (and hence I spend a lot of time managing his unrelated talking unfortunately) and thinks that talking – any kind of talking – is useful and contributing to class discussion.  One of the things I have talked to him about is the idea of active listening and how important not speaking can be.  He has not yet caught onto the idea of being respectful while listening, and still is considering his next talking move while others are trying to make their points.  It will be a difficult discussion, but a necessary one for this young man to grow in important ways.  At least I now have this chart to refer to when I have that discussion.

So was this exercise everything I had hoped?  Not really – but it definitely had some great highlights.  Class discussion was very exciting and interesting while students were aware and deliberate knowing the different types of ways in which they could contribute.  Knowing that they had to hand this table in to me in 5 days was putting the onus on them to show that they had or had not fulfilled what I had observed of them in class.  I do believe they learned a lot about what they were capable of.  I do believe I would do it again.

Does PBL teach Resilience?

I just read a great blogpost by a business writer, Gwen Moran, entitled, “SIx Habits of Resilient People.” When I think of people that I admire in my life for their resilience there was usually some circumstance in their life that led them to learn the quality of resilience because they had to. Even the examples that the author uses in this blogpost – being diagnosed with breast cancer, almost being murdered by a mugger, the inability to find a job – these tragedies that people have had to deal with can be turned into positive experiences by seeing them as ways in which we can learn and grow and find strength within ourselves.

But wouldn’t it be great if it didn’t take a negative experience like that to teach us how to be resilient? What if the small things that we did every day slowly taught us resilience instead of one huge experience that we had no choice but to face? Having to deal with small, undesirable circumstances on a daily basis, with the help and support of a caring learning community would be much more preferable, in my opinion, than surviving a mugging. (Not that one is more valuable than the other). But I just wonder – and I’m truly ruminating here, I have no idea – if it is possible to simulate the same type of learning experience on a slower, deeper scale by asking students to learn in a way that they might not like, that might make them uncomfortable, that asks more of them, on a regular basis.

I think you know what I’m getting at. Does PBL actually teach resilience (while also teaching so much more)? In my experience teaching with PBL the feedback I’ve received from students has been overwhelmingly positive in the end. But initially the comments are like this:

“This is so much harder.”
“Why don’t you just tell us what we need to know.”
“I need more practice of the same problems.”
“This type of learning just doesn’t work for me.”

Having students face learning in an uncomfortable atmosphere and face what is hard and unknown is difficult. Thinking for themselves and working together to find answers to problems that they pose as well as their peers pose is very different and unfamiliar. But does it teach the habits that Gwen Moran claim create resilient people? Let’s see. She claims that resilient people….

1.Build relationships – I think I can speak to this one with some expertise and say that at least if the PBL classroom is run with a relational pedagogy then it is very true that PBL teaches to build relationship. My dissertation research concluded nothing less. In discussing and sharing your ideas, it is almost impossible not to – you need relational trust and authority in order to share your knowledge with your classmates and teacher and this will only grow the more the system works for each student.

2. Reframe past hurts. – If we assume that real-life “hurts” are analogous to classroom mistakes, then I would say most definitely. PBL teaches you to reframe your mistakes. PBL is a constant cycle of attempting a problem->observing the flaw in your solution ->trying something else and starting all over again. This process of “reframing” the original method is the means by which students learn the the PBL classroom.

3. Accept failure – This may be the #1 thing that PBL teaches. I am constantly telling my students about how great it is to be wrong and make mistakes. You cannot have success without failing in this class. In fact, it is an essential part of learning. However, students in the US have been conditioned not to fail, so that reconditioning takes a very long time and is a difficult process.

4. Have multiple identities – In a traditional classroom, certain students fulfill  certain roles – there’s the class clown, the teacher’s pet, the “Hermione Grainger” who is constantly answering the teacher’s questions, etc. But what I’ve found happens in the PBL classroom is that even the student who finds him/herself always answering questions, will also find him/herself learning something from the person s/he thought didn’t know anything the next day. Those roles get broken down because the authority that once belonged only to certain people in the room has been dissolved and the assumption is that all voices have authority. All ideas are heard and discussed. PBL definitely teaches a student to have multiple identities while also teaching them a lot about themselves, and possibly humility, if done right.

5. Practice forgiveness – This might take some reinterpreting in terms of learning, but I do believe there are lessons of forgiveness in the PBL classroom. Students who expect themselves to learn everything the first time and when they don’t, feel stupid, need to forgive themselves and realize that learning is an ongoing process. Learning takes time and maybe needs more than one experience with a topic to see what the deeper meanings and understandings really are. Since PBL is not just a repetitive, rote teaching method, students need to learn how to be patient and forgiving of their own weaknesses as a learner and take time to see themselves as big picture learners.

6. Have a sense of purpose – This habit is about “big picture” purpose and looking at a plan. From the research that I did, I also found that PBL brings together many topics in mathematics, allowing students to see the “big picture” connections between topics much better than traditional teaching does. The decompartmentalization that occurs (as opposed to compartmentalizing topics into chapters in a textbook) is confusing at first because they are not used to it, but eventually students see how topics thread together. Just the other day in my geometry class we were doing a problem where they were asking to find as many points as possible that were 3 units away from (5,4) on the coordinate plane. A student in the class asked, “is this how we are going to get into circles?” The whole class was like “Oh my gosh, it is, isn’t it?” Bam, sense of purpose.

All in all, I feel that PBL meets Moran’s criteria of “resilience characteristics” in ways in which it allows students to practice these habits on a regular basis.  So not only does PBL help students learn collaboration, communication and creativity, but perhaps they will see the benefits over time in learning how to move forward from a setback – just a little.

 

The Downside of Naming “Feminine” Traits

I recently read this article from the Harvard Business Review stating that “Feminine” Values Can Give Tomorrow’s Leaders an Edge.   A study was done asking 64,000 people from over 13 countries all over the world for the traits, skills and competencies that were perceived to be appreciated in leaders in the world of business and leadership.  The conclusions (from statistical modeling) that the analysts came to were that tomorrow’s leaders must overwhelmingly learn to have what our culture has defined to be “feminine” traits.  Here’s the list of the survey said were the top 10 desired traits for modern leaders:

 

I don’t disagree with these traits, honestly, and as a feminist it actually excites me that the values that I work to foster in the classroom are being valued in the boardroom and society in general (Dewey would be proud too).  However, something that is troubling me is the ever-popular dichotomy that is being set up here that seems to always be at the heart of many issues that rise in our society.  Something I wrote about in my dissertation and any time I talk about Relational Pedagogy is the idea of breaking down this concept of masculine vs. feminine thinking, not only in mathematics or education, but in human relations altogether.

I will be the first person to motivate and encourage young women in the STEM fields or take a young boy who likes cooking and say, “you, go guy” and hand him an apron – but that is about individualism and allowing young people to be who they want to be and feel empowered.  In my classroom, allowing students to see multiple perspectives and have their voice heard whether they are male or female is entirely my top priority because they are individuals and their relationship with mathematics is unique.  For a long time in math education, the ideas in this study were how young girls were viewed – researchers thought that if we just saw how girls were different from boys that we could see why they weren’t “doing as well” as boys.  However, we saw that they were doing just as well.

So my problem with this study is not the fact that women will be empowered to become leaders in business – no, that’s really exciting to me.  In fact, maybe some men will see the potential in women and decide to hire more women in the future and this will create more jobs for women and this will in turn, create a more equitable workplace and more favorable working conditions, which will then create more exciting options for business situations because of the fact that different perspectives are being looked at with such different views being taken in problem solving in business.  That is extremely exciting to me!

However, my problem with this study is this.  In order to make such radical changes in how people view gender differences in our society we really need to stop making such huge oppositional statements.  In support of this view, Mendick (2005) stated

By aligning separate-ness with masculinity and connected-ness with femininity, these approaches feed the oppositional binary patterning of our thinking and in the final analysis reiterate it (p 163).

If we just continue to point out how “unfeminine” men are because they are less expressive and how “unmasculine” women because they can be undecisive all we are doing is perpetuating the oppositions that separate us instead of our humanness that can bring us together as learners and our vulnerability that can help us problem solve with our strengths and weaknesses that will make us stronger if we work together.

There was an article published in 2010, about how if you put more women in a group of people the “collective intelligence” increases – the group works better together.  I’m sure there’s some tipping point though that if the group has all women there are diminishing returns for this measure.  There has to come a time when we value the relationships in our learning, our work and our classrooms and as teachers foster all of these traits to the best of our abilities.

PBL – Students making Mathematical Connections

As someone who has used Problem-Based Learning for almost 20 years and sad to say has never been part of a full-fledged Project-Based Learning curriculum, what I know best is what I call PBL (Problem-Based Learning).  I know there is a lot of confusion out there is the blogosphere about what is what, and with which acronyms people use for each type of curriculum.  I did see that some people have been trying to use PrBL for one and PBL for the other, but I guess I don’t see how that clarifies – sorry.

So when I use the acronym PBL in my writing I mean Problem-Based Learning and my definition of Problem-Based Learning is very specific because it not only implies a type of curriculum but an intentional relational pedagogy that I believe is needed to support learning:

Problem-Based Learning (Schettino, 2011) – An approach to curriculum and pedagogy where student learning and content material are (co)-constructed by students and teachers through mostly contextually-based problems in a discussion-based classroom where student voice, experience, and prior knowledge are valued in a non-hierarchical environment utilizing a relational pedagogy.

Educational Psychologist and Cognitive Psychologists like Hmelo-Silver at Rutgers University have done a lot of research on how students learn through this type of scaffolded problem-based curriculum dependent on tapping into and accessing prior knowledge in order to move on and construct new knowledge.  There was a great pair of articles back in 2006/2007 where Kirschner, Sweller & Clark spoke out against problem- and inquiry-based methods of instruction and Hmelo, Duncan and Chinn responded in favor.  I highly recommend reading these research reports for anyone who is thinking of using PBL or any type of inquiry-based instruction (in math or any discipline).  It really helps you to understand the pros and cons and parent and administrator concerns.

However, after you are prepared and know the score, teachers always go back to their gut and know what works for their intuitive feeling on student learning as well.  For me, in PBL, I look at how their prior knowledge connects with how, why and what they are currently learning.  One of the best examples of this for me is a sequence of problems in the curriculum that I use which is an adaption from the Phillips Exeter Academy Math 2 materials.  I’ve added a few more scaffolding problems (see revised materials) in there in order to make some of the topics a bit fuller, but they did a wonderful job (which I was lucky enough to help with)and keep adding and editing every year. The sequence starts with a problem that could be any circumcenter problem in any textbook where students use their prior knowledge of how to find a circumcenter using perpendicular bisectors.

“Find the center of the circumscribed circle of the triangle with vertices (3,1), (1,3) and (-1,-3).”

Students can actually use any method they like – they can use the old reliable algebra by finding midpoints, opposite reciprocal slopes and write equations of lines and find the intersection points.  However, I’ve had some students just plot the points on GeoGebra and use the circumcenter tool.  The point of this problem is for them to just review the idea and recall what makes it the circumcenter.  In the discussion of this problem at least one students (usually more than one) notices that the triangle is a right triangle and says something like “oh yeah, when we did this before we said that when it’s an acute triangle the circumcenter is inside and when it’s an obtuse triangle the circumcenter is outside.  But when it’s a right triangle, the circumcenter is on the hypotenuse.”

Of course then the kid of did the problem on geogebra will say something like, “well it’s not just on the hypotenuse it’s at the midpoint.”

 

Dicussion will ensue about how we proved that the circumcenter of a right triangle has to be at the midpoint of the hypotenuse.

A day or so later, maybe on the next page there will be a problem that says something like

“Find the radius of the smallest circle that surrounds a 5 by 12 rectangle?”

Here the kids are puzzled because there is no mention of a circumcenter or triangle or coordinates, but many kids start by drawing a picture and thinking out loud about putting a circle around the rectangle and seeing they can find out how small a circle they can make and where the radius would be.  When working together oftentimes a student see a right triangle in the rectangle and makes the connection with the circumcenter.

A further scaffolded problem then follows:

“The line y=x+2 intersects the circle  in two points.  Call the third quadrant point R and the first quadrant point E and find their coordinates.  Let D be the point where the line through R and the center of the circle intersects the circle again.  The chord DR is an example of a diameter.   Show that RED is a right triangle.”

Inevitably students use their prior knowledge of opposite reciprocal slope or the Pythagorean theorem.  However, there may be one or two students who remember the circumcenter concept and say, “Hey the center of the circle is on one of the sides of the triangle.  Doesn’t that mean that it has to be a right triangle?”  and the creates quite a stir (and an awesome “light bulb” affect if I may say so myself).

A few pages later, we discuss what I like to call the “Star Trek Theorem” a.k.a. the Inscribed angle theorem (I have a little extra affection for those kids who know right away why I call it the Star Trek Theorem…)

I will always attempt to revisit the “RED” triangle problem after we discuss this theorem.  If I’m lucky a student will notice and say, “Hey that’s another reason it’s a right triangle – that angle opens up to a 180 degree arc, so it has to be 90.”  and then some kid will say “whoa, there’s so many reasons why that triangle has to be a right triangle”  and I will usually ask something like, “yeah, which one do you like the best?” and we’ll have a great debate about which of the justifications of why a triangle inscribed in a circle with a side that’s a diameter has to be right.  So who are the bigger geeks, their teacher who names a theorem after Star Trek or them?

References:

Kirschner, P. A., Sweller, J., & Clark, R. E. (2006). Why minimal guidance during instruction does not work: An analysis of the failure of constructivist, discovery, problem-based, experiential and inquiry-based teaching. Educational Psychologist, 41(2), 75-86.

 

Hmelo, C. E., Duncan., R.G., & Chinn, C. A. (2007). Scaffolding and achievement in problem-based and inquiry learning: A response to Kirschner, Sweller and Clark (2006). Educational Psychologist, 42(2), 99-107.

 

Why I disagree with Mr. Kahn

6 Comments

I have to say that I am not usually a controversial blogger – I’ll just put that out there right away.  However, I am so frustrated with the conversations, blog posts and articles that are zipping around the blogosphere about online learning, MOOCs and Khan Academy that I have to say something about it as a teacher, teacher educator and responsible learner, myself, about education theory.  I have taught online classes, taken online classes, used open source materials for my classes and definitely promote the idea of equal “world-class education for anyone, anywhere.”  However, I have yet to see how that quality education occurs online and especially the way that it is promoted in Salman Khan’s book, The One World Schoolhouse: Education Reimagined.

Now let’s just put something else out there right away – it might be that I am frustrated by the fact that he has no background experience in education (which he admits – “I had no teacher training”) and I am offended that he is speaking out of turn speaking as if he does.  For example, he says “There’s an old saying that ‘life is school.’”  Hmmm, I wonder who said that? And I’m not sure that’s really the right saying.  Or it could be that he is attacking the very discipline that I am working so hard to change – mathematics.  I totally agree that there is a lot that is wrong with the way mathematics is taught in the U.S.  But NOT going all “rogue” and working against the people who have already done some research on the subject and know a little about which they talk, might be a good place to start.  There are many things that Mr. Kahn discusses in his book that he seems to purport as novel ideas like Mastery Learning, Flipping the Classroom, etc. that are not his ideas.  So let’s pretend that the fact that he wrote a book of concepts that seem to be a compilation of educational reform ideas that have been around for a while is not what really annoys me.

What really gets my goat, if I seem to have his idea right, is that he is advocating for “a free world-class education, for anyone anywhere” but I’m not really seeing how this is going to happen.  He advocates for the use of the Khan Academy for mastery learning in the classroom (in a school system) where the students watch the videos and then come to class and do “projects” with each other in the “one room schoolhouse.”  I actually agree that this is a wonderful learning scenario that promotes creativity, independence in learning and individualized lessons for students of all ability levels.  Besides the huge government and system-wide testing restrictions that are currently in place and teachers’ current use of assessment, it would be very difficult (but not impossible) to change this system.  Kahn very naively writes a 5-page chapter on Tests and Testing, which again is nothing new, on the evils of standardized testing and why they don’t really tell you anything about students’ knowledge.  His “one room schoolhouse” is an idealistic utopia of learning for someone who has never been in the classroom and dealt with classroom management, assessment, review or planning of these open-ended projects.  I do believe that a great deal of teacher training would need to be reformed and reviewed in order for something like this to happen and before any school thinks of moving to a model like this they should think wisely about the ways in which teachers are ready to handle the change of the classroom culture and how they are ready to deal with it.  Students will still have questions about the material and will all be at different places in the content and the projects, which will probably demand more planning from the teachers (which again, is not a reason not to flip the classroom, but a necessity of which to be aware). I found what he put forth as the ideal classroom short-sighted and with many limitations.

Secondly, what about the “anyone, anywhere” Idea? Even if children in third-world countries have access to internet-ready computer to watch these videos, where are the teachers and schools to have them do the “world-class” learning with these group projects?  Where is their utopian learning environment?  I am confused about how watching videos online is giving them a “world-class” education (although I could see how it was free if Mr. Gates donated a bunch of computers and Internet access, etc.).  Mr. Kahn also realized that “teaching is a …skill – in fact, an art that is creative, intuitive, and highly personal…[which] had the very real potential to empower someone I cared about.”  Yes, Mr. Kahn, that’s what teaching is all about.  Teaching is about, as you said, “genuinely [sharing  your] thinking and express[ing] it in a conversational style, as if I was speaking to an equal who was fundamentally smart but just didn’t fully understand the material at hand.”  How is that supposed to happen for someone sitting alone watching a video?

In the NY Times article, The Trouble with Online Learning, Mark Edmunson wrote:

“Learning at its best is a collective enterprise, something we’ve known since Socrates. You can get knowledge from an Internet course if you’re highly motivated to learn. But in real courses the students and teachers come together and create an immediate and vital community of learning. A real course creates intellectual joy, at least in some. I don’t think an Internet course ever will. Internet learning promises to make intellectual life more sterile and abstract than it already is — and also, for teachers and for students alike, far more lonely.”

This is the heart of Relational Pedagogy, that the interhuman connection between people is what constructs knowledge and the trust, authority, and value of perspective that is shared and given to each other is just as important as the content that is exchanged – most especially in mathematics, it’s just taking us a lot longer to figure this out, Mr. Kahn.

The Role of Technology in Relational Pedagogy?

So I’ve been thinking a lot lately about technology and learning.  There’s so much in the news about MOOCs, using iPads, schools using technology, etc.  I am even part of a pilot program at my school right now where all of my students have iPads in my honors geometry class and we are trying to communicate at night using Voicethread and the iPads.  My hope was that having a way to share ideas during the evening would lessen the stress of homework problems that students are asked to grapple with in the PBL curriculum would give them more opportunities to throw out problem-solving ideas with each other before class starts so that we would spend less time in class debating different methods of solving the problem (although that’s what I love about class, right?).

But I’m asked as a teacher to find ways to integrate technology into my classroom – but to what end?  I want to find ways to use technology to solve problems, to explore ideas and to help improve students’ understanding of the mathematics.  Not necessarily help them communicate with each other, which is what I’m finding most of the apps out there are for right now – which I am open to – but they are removing a huge part of the learning triangle.  In fact, David Hawkins (1974) wrote about the I-thou-it reciprocal relationships in learning that simply must exist between the learner, the teacher and the subject matter.  He said that if one of the relationships is hindered or dysfunctional in some way, that learning is not optimal.

Hawkins (1974)

So if I interrupt that relational triangle between the students’ communication with the material (and with each other) and with me, using technology instead of discussion and the connection with all three, my fear is that learning is not optimal.  Perhaps the technology could enhance it, but for now I see that it is not truly happening.  My guess is that it has to take time for the students maybe to want for that to happen.

I also just read an article on Edutopia by a guy named Matt Levinson that was entitled “Where MOOCs Miss the Mark: The Student-Teacher Relationship” where it was stated that a lack of mentorship, close guidance or meaningful relationship between teachers and students is what is really lacking in these online courses. Even students who use Khan Academy lectures for “learning” sometimes comment that even though they don’t like sitting and listening to lectures in math class, they would “much [prefer] listening to her math teacher explain the same concepts because she likes this teacher and feels comfortable asking questions and going for extra help outside of class.”

Carol Rodgers (one of my most favorite people on earth) writes about teacher presence and the importance of it in the classroom.  I believe in mathematics class and especially the problem-based mathematics class it is truly essential because in order for students to take a risk with a method, they need to feel supported and safe in order to be open to new ideas and to discuss them with others.  With the open presence of a teacher and mentor, students are not “receiving knowledge” but creating it with others – creating it within those relationships that Hawkins was talking about – maybe with technology or without it.  But  for someone who just spent two years writing about the importance of relational pedagogy in PBL, I find it extremely difficult to assume that without those relationship the same exceptional amount of learning would go on.

To Kahn or not to Kahn in PBL

Recently there have been some discussions going around the Internet concerning Kahn Academy and other Internet-based “teaching tools” and their applicability or acceptability in terms of pedagogically sound classroom use. You can check out Dan Meyer’s blog or tweets about the MTT2k project, which I find pretty amusing actually, or Kate Nowak’s blog entry where she stated “Enduring learning requires productive struggle and time to noodle out unfamiliar problems, posed by a teacher who knows what you’re ready for, and can provide expert scaffolding. Lecture-only instruction focused on mastering procedures is an impoverished substitute for doing mathematics, and it doesn’t matter if that lecture is in person or in a video.” To that, I, of course, say, “here, here.” I spent some time going over the Kahn Academy website this past spring when my son was having some trouble studying for his science final exam and he was looking for some review materials and I actually thought it was something of a helpful resource for him. However, I’m not quite sure that it would’ve been a helpful way for him to have learned about genetics the first time around.

On the website, Kahn Academy has a great mission of having open-source curriculum for everyone, everywhere, which I am wholeheartedly in favor of. I believe that education needs to be the great equalizer and one of the best ways to do that is to actually allow everyone equal access to the same quality of education. However, they also seem to take pride in the fact that there are now “5th graders relentlessly tackling college-level math to earn Khan Academy badges” perhaps at the detriment of their understanding or even at the skills that they should be learning at their grade level (and I am definitely not against kids exploring interesting advanced topics or even discussing non-Euclidean geometry before they get to college, for example). So it’s important for there to be balance, as I always say, between content and process.

So overall, I would say, I have no problem with Kahn Academy’s (or any online institution of learning’s) pronouncements that they are helping to “spread the wealth” of education, but I do wonder about the quality of the instruction. They have some very, very smart people working there with very good goals about making education accessible, with which I totally agree and for that I commend them. However, there are lots of theories of education – both online and face-to-face that need to be considered in order to claim that any actual learning (whatever definition of that you are also claiming) is actually happening.

Before educators who are within F2F classrooms move to using online tools to “flip” classrooms in order to substitute for other methods of instruction and claim to be using Project-based or Problem-Based Learning, I encourage everyone to really explore the pedagogical methods of that online tool. Is it congruent to what you would do in the classroom? Does it actually help facilitate the type of learning you would want your students to experience? Does it ask the questions or help with the explorations that you would want them to grapple with themselves? Do they get to the confident explanation and security in the knowledge that they would in a discussion? If not, look for something else. Or even better, ask the questions or pose the problems yourself or get the students to ask each other.