So I heard that what everyone is saying about the new Star Wars Movie, The Force Awakens, is that “Everything Old is New Again” – go ahead google it, there are at least 5 or 6 blog posts or articles about how “BB-8 is the new R2D2” or “Jakku is the new Tattoine” or whatever. I actually don’t have a problem with J.J. Abrams reusing old themes, character tropes or storylines because I think that really great stories are timeless and have meaning and lessons that surpass the movie that you are watching. I still thought it was awesome.
This concept of everything old is new again really hit home to me today in my first period class when I was having the students do a classic problem that I probably first did in 1996 while I was under the tutelage of my own Yoda, Rick Parris (who I think wrote the problem, but if someone reading this knows differently, please let me know). The problem goes like this:
Pat and Chris were out in their rowboat one day and Chris spied a water lily. Knowing that Pat liked a mathematical challenge, Chris announced that, with the help of the plant, it was possible to calculate the depth of the water under the boat. When pulled taut, directly over its root, the top of the plant was originally 10 inches above the water surface. While Pat held the top of the plat, which remained rooted to the lake bottom, Chris gently rowed the boat five feet. This forced Pat’s hand to the water surface. Use this information to calculate the depth of the water.
What I usually do is have students get into groups and put them at the board and just let them go at it. Today was no exception – the first day back from winter break and they were tired and not really into it. At first they didn’t really know what to draw, how to go about making a diagram but slowly and surely they came up with some good pictures. Some of the common initial errors is not adjusting the units or mislabeling the lengths. However, one of the toughest things for students to see eventually is that the length of the root is the depth of the water (let’s call it x) plus the ten inches outside of the water’s surface. Most students end up solving this problem with the Pythagorean Theorem – I’ve been seeing it for almost 20 years done this way. Although I never tire of the excitement they get in their eyes when they realize that the hypotenuse is x+10 and the leg is x.
However, since everything old is new again, today I had a student who actually is usually a rather quiet kid in class, not confused, just quiet, but in a group of three students he had put his diagram on a coordinate plane instead of just drawing a diagram like everyone else did. This intrigued me. He initially wrote an equation on the board like so:
y= 1/6 (x – 0)+10
and I came over and asked him about it. He was telling me that he was trying to write the equation of one of the sides of the triangle and then I asked him how that was going to help to find the depth of the water. He thought about that for a while and looked at his partners. They didn’t seem to have any ideas for him or were actually following why he was writing equations at all. He immediately said something like, “Wait, I have another idea.” and proceeded to talk to his group about this:
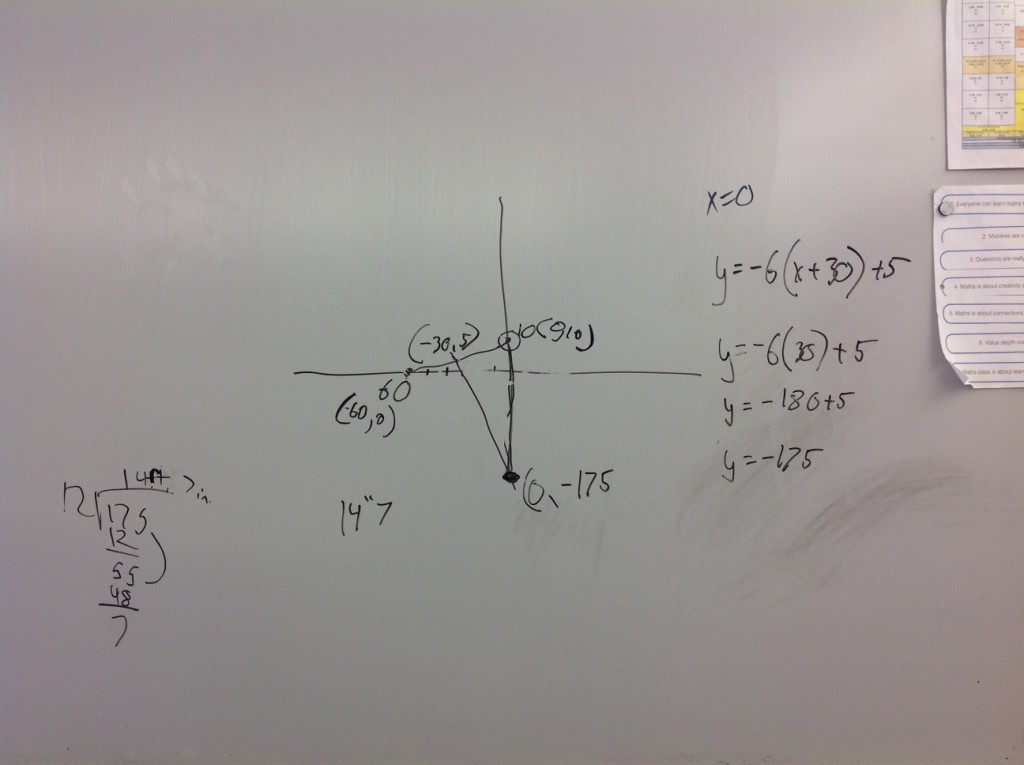
He had realized from his diagram that the two sides of the triangle would be equal and that if we wrote the equation of the perpendicular bisector of the base of the isosceles triangle and found its y-intercept he would find the depth of the water. He proceeded to find the midpoint of the base, then the slope of the base, took the opposite reciprocal and then evaluated the line at x=0 to find the y-intercept. I was pretty impressed – I had never seen a student take this perspective on this problem before.
This made my whole day – I was really dreading going back to work after vacation and honestly, first period was the best class of the day when this wonderful, new method was shown to me and this great experience of this student’s persistence refreshed my hope and interest in this problem. Perpendicular bisectors are the new Pythagorean Theorem!