Phew…exams given…check…exams graded…check…comments written…check…kids on bus…check. Now I can relax. Oh wait, don’t I leave tomorrow to drive to my sister’s for Thanksgiving?
Such is the life of a teacher, no? Just when you think you are on “vacation” there’s always something else to do. I had an exam on Saturday then worked the rest of Saturday and Sunday finishing up that grading and writing my comments that were due this morning at 9 am. But wait, I told some people I would write a blogpost about what my classroom is like, so I really wanted to do that too. That’s OK though, I think it’s important for me to reflect back on this fall term – what worked and what didn’t for my classes.
I have three sections of geometry this year that I teach with PBL and a calculus class that I would say is something of a hybrid because we do have a textbook (as an AP class I needed to do what the other teachers were doing), but I do many problems throughout the lessons.
In my geometry classes, the student have iPads on which they have GeoGebra, Desmos and Notability where they have a pdf of their text (the problems we use) and where they do all of their homework digitally. My class period for that course alternate between small group discussions in the Innovation Classroom in the library on Mondays and Thursdays and whole class discussions with student presentations of partial solutions (a la Jo Boaler or Harkness) on Tuesdays and Fridays. (We meet four times a week 3 45-minute periods and 1 70-minute period.) Because my curriculum is a whole-curriculum PBL model, we spend most of the time discussing the attempts that the students made at the problems from the night before. However, in class the discussion centers around seeing what the prior knowledge was that the presenter brought to the problem and making sure they understood what the question was asking.


If this didn’t happen we end up hearing from others that can add to the discussion by asking clarifying questions or connecting the question to another problem we have done (see Student Analysis of Contribution sheet).
One of the things that I had noticed this fall in the whole class discussion was that the students were focusing more on if the student doing the presentation was right immediately as opposed to the quality or attributes of the solution method. There was little curiosity about how they arrived at their solution, the process of problem solving or the process of using their prior knowledge. Unfortunately, it took me a while to figure this pattern out and I felt that it had also weeded itself into the small group discussion as well.
One day in the small group discussions, it became clear to me that the students were just looking for the one student who had the “right” answer and they thought they were “done” with the question. This spurred a huge conversation about what they were supposed to be doing in the conversation as a whole. I felt totally irresponsible in my teaching and that I had not done a good enough job in describing to them the types of conversations they were supposed to be having.
This raised so many questions for me:
- How did I fail to communicate what the objectives of discussing the problems was to the students?
- Why is this class so different from classes in the past (even my current period 7 class)?
- How can I change this now at this point in the year?
- How can I stress the importance of valuing the multiple perspectives again when they didn’t hear it the first time?
In my experience, sometimes when students are moving forward with the fixed mindset of getting to the right answer and moving on, it is very difficult to change that to a more inquiry-valued mindset that allows them to see how understanding a problem or method from a different view (graphical vs algebraic for example) will actually be helpful for them.
My plan right now is to start the winter term with an interesting problem next Tuesday.
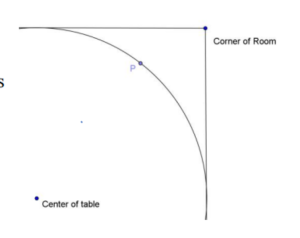
“A circular table is pushed into the corner of the room so that it touches both walls. A mark is made on the table that is exactly 18 inches from one wall and 25 inches from the other. What is the radius of the table?”
I have done this problem for many years with students and I have found the it works best when they are in groups. I usually give them the whole period to discuss it and I also give them this Problem Solving Framework that I adapted from Robert Kaplinsky’s wonderful one from his website. I am hoping to have a discussion before they do this problem about listening to each other’s ideas in order to maximize their productivity time in class together. We’ll see how it goes.
I’m sure you do this already, but what seems to have motivated my “regular” algebra2 class is the notion of the “best” mistake. I have overrun them with jo boalers videos about how their brain grows with struggle. I even had a student comment in a discussion, “my brain really grew on that one.” Lol.
Thanks Lea Anne – yes I have talked about the mistakes they make and growth mindset a great deal. But it’s never enough, is it? I think I might try some of those techniques like you are talking about where students write down their wrong answers on index cards and the teacher chooses one anonymously for students to critique (in a good way).
I could even have them project from their iPad anonymously if they think they have a “good” mistake too. thanks for the encouragement!
Thanks for the post! I can empathize with many of the frustrations you listed, mainly the “we’re done, let’s move on mentality”. I’m glad I’m not alone.
Yes, it’s really tough to work against. But it might be easy to forgive thinking of the culture of schools that most kids might come from? I get frustrated but hopefully it’s not frustration with them per se, but frustration with the whole system of learning, right? SO hard to get them to learn from a place of curiosity and interest if that is not innately there.
This is exactly how I feel about my class some days. I’m hoping to do better with explaining the expectations and benefits of looking for more than just the “right” way. This has certainly been hard.
It’s definitely become more of an uphill battle in the last 6-8 years. I’m not sure why – perhaps it’s a higher level of importance placed on the college process, and earlier? I know that 20 years ago I felt that it was easier.
We can only keep allowing students to see the intrinsic rewards. I’m going to write a blogpost about what happened next – which had some good results, luckily!
I think that something that I do that contributes to the problem that you describe is still overly emphasize correct work in their grade. My experience is that our students are very grade motivated, but I know that you already have numerous things that reward students for things other than “correct answer on timed test.” I mean, I am very much a beginner and you may have already resolved this tension, but it end of term grade feels very relevant to how we define the successful practice of mathematics to our students.
Thanks for the post. I appreciate the reminder that just as our students don’t fully resolve every question we pose to them, we do not always achieve our full aims with every class 🙂