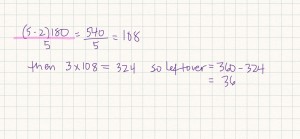I’d like to think of myself as a master teacher. I’ve always thought of myself as very aware of student perspectives in my classroom, but today after a weekend of being in bed with a bad cold, all I wanted to do was get through the problems and get back home – I admit it. I was not being very reflective and deliberate in my teaching. However, even with all that, something amazing happened today. Of course, I’d like to take all the credit, but I have to say I think the credit goes to the method of PBL, relational pedagogy and the students in my class. So here’s what happened.
We had gone through about half the problems and I think they could tell I was in a pretty bad mood. We got to one of my favorite geometry problems that starts an interesting strand of thinking that has to do with which polygons tessellate with others. One girl goes to the board (I’ll call her Robin) and she presents her solution to this problem:
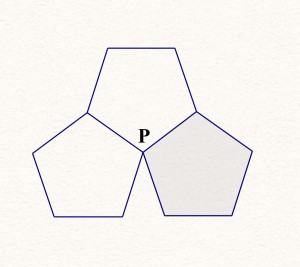
The diagram at the right shows three regular pentagons that share a common vertex at P. The three pentagons do not quite surround P. Find the size of the uncovered acute angle at P.
So Robin does what I expect (and in my fuzzy state of mind I am just happy that someone knows what’s going on). She writes on the board:
Her argument being that the three angles in the pentagons were congruent since they were all regular and the leftover part would be the difference between those three and 360. So, I was ready to move on. She was right, after all? Let’s go and do the next problem. But no, Tye speaks up and says, “Hey that’s what I got but that’s not what I did. That’s so cool I got the right answer.” So, as tired and sick as I was, I said, “What did you do Tye?” He says all I did was do 108 divided by 3 and it worked!” He was so proud of himself. I sat there and was like, OK, this isn’t going to fly, but I was so exhausted that ….but wait, another student says, “Hey that’s cool. You just take the angle measure and divide it by how many polygons you have.” I’m thinking, oh no, this is gonna get out of hand fast….
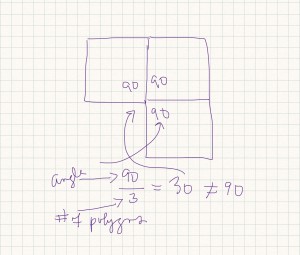
Then another student says, “wait a minute, lets see if it works with hexagons: ” So before they know it they realize that it can’t work with hexagons and Helen says, “but that’s because there are too many, you need something with room left over, like a square. What if you only use 3 squares? Is the angle leftover 90 divided by 3?”
So they soon have disproved the theory that if they just take the number of interior angle of the regular polygon and divide by how many polygons there are and divide them, they’ll get the leftover angle. But Tye is still adamant that he’s all proud he got the right answer. I am, however, still struggling with the fact that he can’t justify to himself why it works. I say,”Listen, why don’t you think about it some more and we’ll come back to it?” but guess what, they don’t let it go.
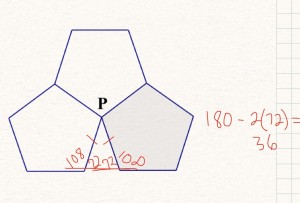
Luke says, “Well, what I did was just draw a triangle down there.”
He says that he knew that empty space was really an Isosceles triangle and because the base angles were supplementary to the interior angles of the pentagon, he could find the angle at the top. At this point, I’m like “will this ever end? Will I be able to get some Tylenol?” (I know can you believe me? what a role model…) A few other kids really liked what Luke did and said they did that too and thought they had been wrong, but now see that it was a valid method. I mean, could I ask for more? This was awesome stuff going on!
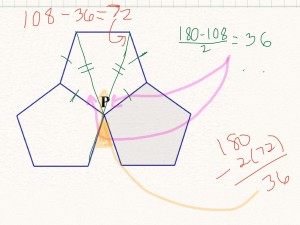
So at this point, we move on and do a few more problems, but then towards the end of class, I notice there are about three kids who aren’t really paying attention to the problem at hand. I couldn’t figure out what was going on because they are usually right in the thick of our discussions. So finally, one girls practically yells (and I mean, with arms flailing and everything) “I got it!” Alanna had been working on a justification for Tye’s idea of dividing the interior angle by three the whole class period, as had two other students. It was so interesting a problem to them that they just couldn’t stop. Alanna said, “I knew they were vertical angles, but I just couldn’t see how they could be the same.” She and the two other students had been playing with some isosceles triangles and vertical angles and come up with this solution:
By finding that the base angles of the isosceles triangles on the sides were both 36, and that the one in the middle was also 36, they had seen the reason why Tye could just divide 108 by 3. They knew it wasn’t just pure luck that it worked and it made them all so happy. It was so satisfying and I could just feel the excitement in the room.
It was so funny to me because everything that I did to try to discourage them from going to that place of curiosity or demanding the reason didn’t help. They went there anyway. Their voices were heard – again and again. The culture that we had set was there and no matter what I did now, at this point in the year, they knew what was expected of them – asking questions, not giving up, being inquisitive & creative. This class helped me realize that even on my bad days I need to see each student for who they are and be just as excited for each of them to realize their own potential. And if I don’t have the energy or strength to push them through, maybe, just maybe, they will.