I just got back from a great visit to Toronto (which was also my first visit to that wonderful city.)  I spoke at a conference and also did some work at beautiful girls’ school there that was interested in PBL.  It was the first time where two of my research interests intersected (Gender and PBL) and it was fascinating for all that were involved – quite wonderful and so much fun.

Many teachers talked to me there (and it comes up everywhere I go) about the fact that they are the only teacher, or one of the few, at their school that is interested in  trying out this different method of teaching, but need to keep up with the syllabus that their colleagues are using in order for students to be prepared for the common exam either at the end of the term, the end of the semester or even on a monthly basis.

This can be problematic when there are school districts that dictate down to the homework assignment or classroom activity that you need to be doing on a daily basis.  The free that classroom practitioners need to make decisions about what is best for the learning of their students is quite important.  However, it is still possible to integrate problem solving or methods of PBL into your classroom when you can get.

I talk about the Continuum of PBL when I give workshops to let people know that you don’t have to dive in head first if you want to try your hand at classroom discourse a little at a time.  Learning to facilitate discussion as a long-time direct instruction teacher is actually quite difficult to allowing students to have more authority can be tricky.  Here is the visual aid that I use when I discuss it:

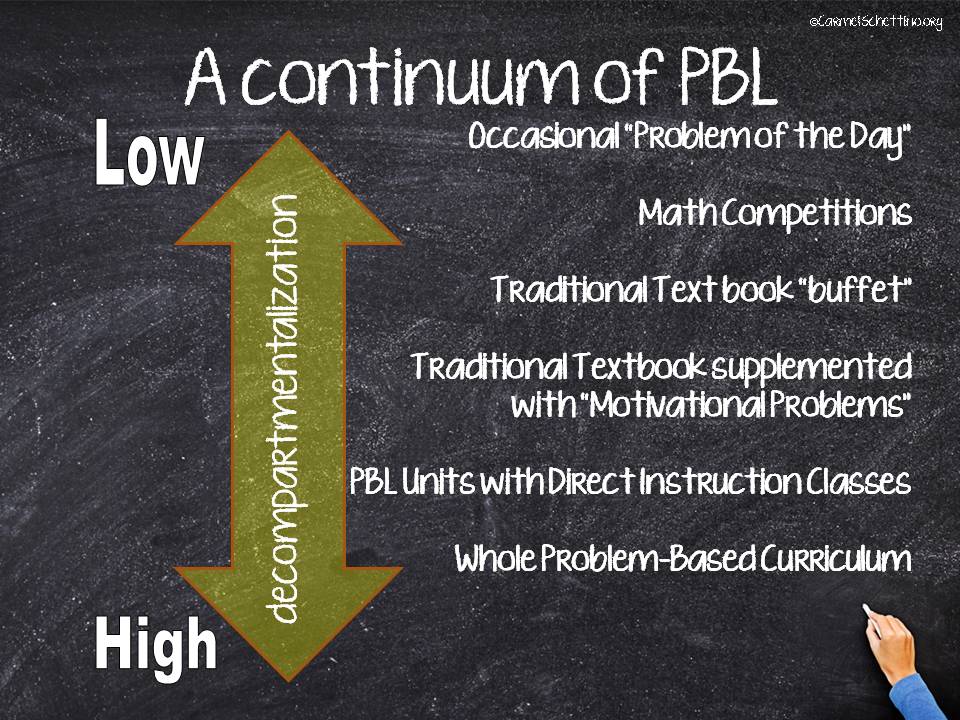
The arrow tells you the level of decompartmentalization of topics – in other words how the topics are blended together or not.  In a traditional mathematics curriculum, a textbook artificially separates mathematics into what I like to think of a “compartments” that in a very linear order and most students learn to believe that mathematics must be taught in that order.  Yes, some operations and skills must come before others, but conceptually a great deal of mathematics can be learned or thought about in no particular order.  It is all extremely and equally fascinating.  In a whole problem-based curriculum such as what the faculty at Phillips Exeter Academy has written there are no chapters that mark the ending of the content and the beginning of another since there truly no time when that content is no longer applicable to the new material that is being learned (yes, of course that is true in a text book as well, but the chapter alone have come to imply that to students).

Decompartmentalization can come at different levels.  At the lowest level,many  teachers use “Problems of the Day” that challenge students at the beginning of class with logic puzzles, topics they are not seeing regularly or interesting tidbits like soduku or other fun activities to get students’ minds working.  These create discussion and allow them to see problem solving in action.  However, there is little connection to the mathematics that is being learned in the class proper.

I won’t discuss every type of PBL on the continuum or this will turn into one of my hour long talks, but I will say that if you are interested in attempting to keep up with your colleagues who are following a traditional syllabus but you might want to use less direct instruction I have a link to my “Motivational Problems” page in order for you to have them start the conversation based on their prior knowledge of material.  The problems are listed by topic and you can have them move forward in class by presenting problems and then have them practice with problems in the textbook.  Anyone who tries this – I’d love to hear from you!

This was how I first started at my last school and it worked well for me.  You can read my article from 2003 in the Mathematics Teacher to learn what it was like.  But it definitely moved me in the right direction.  Keep pushing on!