Category: Problem based learning

Teaching Students to Become Better “Dancers”

So the other day I read a tweet by Justin Lanier that really sparked my interest.

 We all know the scenario in classroom discourse where a student asks a question – a really great question – and you know the answer, but you hedge and you say something like, “That’s a great question! I wonder what would happen if…”  So you reflect it back to the students so that they have something to think about for a little while longer, or maybe even ask a question like “Why would it be that way?” or “Why did you think or it like that?”  to try to get the student to think a bit more.  But what Justin, and the person who coined the phrase “authentic unhelpfulness” Jasmine Walker (@jaz_math), I believe were talking about was hedging because you really don’t know the answer – sincere interest in the uniqueness of the question – not because you’re so excited that student has helped you move the conversation forward, but because of your own excitement about the possibilities of the problem solving or the extension of the mathematics.

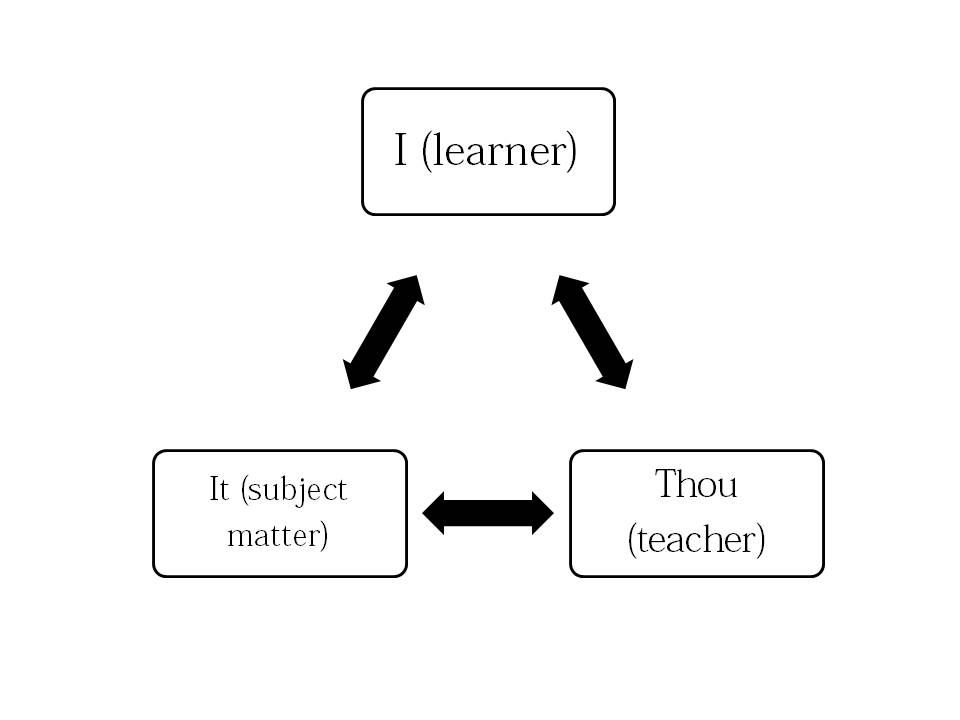
I think what got me so excited about this idea was how it connected to something that I was discussing earlier this summer with a group of teachers in my scaffolding in PBL workshop in late June.  In a PBL curriculum, the need to make sure that students have the right balance of scaffolded problems and their own agency is part of what Jo Boaler called the “Dance of Agency” in a paper she wrote in 2005 (see reference).  My understanding of this balance goes something like this:

(c) Schettino 2013

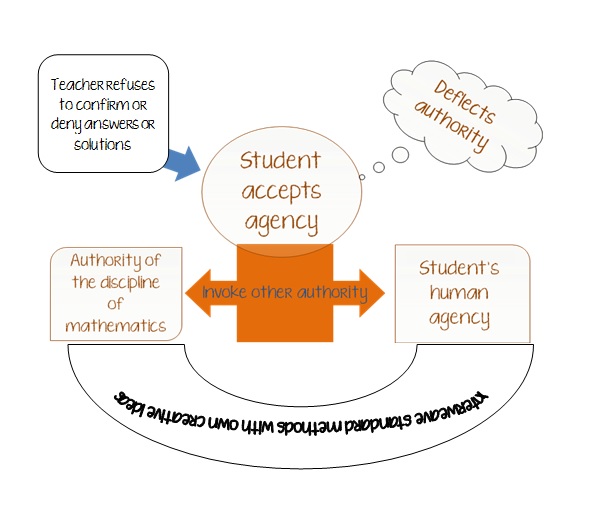
So initially, the student is confused (or frustrated) that the teacher refuses to answer the question although you are giving lots of support, advice and encouragement to follow their instincts.  The student has no choice but to accept the agency for his or her learning at that point because the teacher is not moving forward with any information.  But at that point usually what happens is that a student doesn’t feel like she has the authority (mathematical or otherwise) to be the agent of her own learning, so she deflects the authority to some other place.  She looks around in the classroom and uses her resources to invoke some other form of authority in problem solving.  What are her choices?

She’s got the discipline of mathematics – all of her prior knowledge from past experiences, she’s got textbooks, the Internet, her peers who know some math, other problems that the class has just done perhaps that she might be able to connect to the question at hand with previous methods that she might or might know how they work or when they were relevant – that discipline has had ways in which it has worked for her in the past and lots of resources that can help even if it may not be immediately obvious.

But she’s also got her own human agency which is most often expressed in the form of asking questions, seeing connections, drawing conclusions, thinking of new ideas, finding similarities and differences between experiences and thinking about what is relevant and what is not.  These pieces of the puzzle are not only important but a truly necessary function of the “dance of agency” and imperative to problem solving.

Interweaving both of these types of agency (and teaching kids to do this) have become more important than ever.  Yes, being able to use mathematical procedures is still important, but more important is the skill for students to be able to apply their own human agency to problem and know how and when to use which mathematical procedure, right?  This “dance” is so much more important to have every day in the classroom and if what initiates it is that deflection of authority then by all means deflect away – but the more we can “dance” with them, with “authentic unhelpfulness” and sincere deflection because we need to practice our own human agency, the more we are creating a true community of practice.

Boaler, J. (2005). Studying and Capturing the complexity of practice – the Case of the ‘Dance of Agency’

So How Do We Shift Gears?

OK, OK, I get the idea – not everything on the Internet is true and, for sure, not everything on the Internet is meaningful or helpful.  Since April of this year I have started following a bunch of people on Twitter (before that I really didn’t even know what it was or care) and thought that there were so many people out there that I wanted to learn from.  I would read other people’s blogs and try my best to think about what I had to learn from others. Mind you, I know I am definitely not the god of teaching, that’s for sure, but many of the things that are written out there – should I guess – with the hope of being “inspirational” or meaningful to others, I find less than helpful.

One site that I have really enjoyed reading which often has some great links and blogposts is Mindshift.  But they just tweeted this blog entry that cited an article about creating a business that fosters creativity.  OK, I see the connection to education, but honestly, it is a very different machine.  Kids and adolescents have a very different mindset than adults who are out there making money.  Not to mention the consequences of risk-taking in the classroom vs. risk-taking in the office have the potential for being very different.  (Assessment for grades has a different meaning possibly for a 13-year-old mind than brainstorming on the job, vs. assessment for a salary raise, etc for an adult who we hope can handle the pressure a little more.)

Then the blogger writes two short paragraphs at the end about how schools are just “incurious and risk averse” places.  Basically stating that schools don’t ever allow students to practice risk-taking or mistake making at all:

“Too few schools are incubators of curious and creative learners given their cultures of standardization, fear, and tradition. No doubt, external pressures exist that drive that culture. But if there ever was a time to shift gears, this is it. “

No doubt…sadly, our blogger, Will Richardson doesn’t really give us any advice on what to do about it….except, to do something about it. (Admittedly, he may have written something someplace else that I missed.)  And I don’t want to single out Mr. Richardson – I find tweets and blogs like this all day long – “Exploration, inquiry & problem solving are powerful learning mechanisms…” or “asking good questions and promoting discourse is an integral part of teaching and learning”…. Hmmm, well let me think about ways in which we can talk to teachers  in terms of mistake making and risk-taking:

  • Blogpost on making mistakes and classroom activity tied to Kathryn Schultz’ TED talk On Being Wrong
  • Discussion about article “Wrong is not always bad” with teachers
  • Modeling risk-taking in Problem-Solving in my course at ASG conference in June
  • Discussion of Relational Pedagogy to foster Risk-taking
  • Using a PBL curriculum to foster mistake-making and communication

I found that many teachers that I work with and who contact me are entirely dedicated to changing the culture of the mathematics classroom in the U.S. and making it (as Mr. Richardson writes) an “incubator of curious and creative learners.”  We need to make changes to our curriculum, our classroom relationships, our classroom culture and the authoritarian hierarchy that traditionally is prevalent in our mathematics classroom.  Students need to be able to feel safe enough, from judgment, alienation and failure to make those mistakes while learning.  We, as teachers, need to begin the discussion with each other about how to move forward with these initiatives and make sure that student voice is heard in the mathematics classroom as they question each other and us, the teachers, with true questions – ones we may not be able to answer.  These are the important aspects of creating curious learners who make mistakes and learn from them.  But we, as the adults in the room have a responsibility to let them feel safe in doing that.

I think teachers are aware of the fact that it’s time to “shift gears” – to make the classroom more conducive to students working together and taking chances.  There are so many subtleties to making this shift, however.  Students who need to shift, parents who are not used to that, assessment changes to be made – the list goes on and on.  I am doing what I can to help people with this conversation.  The pedagogy of relation (I believe) is at the heart of all of this – keeping in mind that in order for people to be vulnerable and make mistakes, we need to consider the interhuman aspect of learning.  In a classroom where this connection has for too long been typically so acceptably removed, it will take a lot of work to make this big “gear shift” but I’m up to it – bring it on!

A Total Win…with lots of understanding

Before I left for the Anjs S. Greer Math Conference last week, I read an amazing blog entry at the Math Ed Matters website by Dana Ernst and Angie Hodge that was talking about Inquiry-Based Learning and the mantra “Try, Fail, Understand, Win.”  The idea came from one of Prof. Ernst’s student course evaluations this past spring as his student summed up his learning experience in such an IBL course.  This blog post was so meaningful to me because for each of these four words, the authors wrote how we as teachers (and teacher educators) can take this student’s perspective towards our own work.  I decided to attempt to take this attitude going off to my own conference with two courses to give and three smaller talks.  It was sure to be a busy week.

And in fact, it really was.  I had very little time to sit and listen to others’ work, which I really was quite sad about.  However, in my own classes I was so impressed with the amount of enthusiasm and excitement my participants had for PBL and their own learning.  As I sat in front of my computer this morning reading the course evaluations and their tremendously helpful input, it finally occurred to me how truly powerful the experience had been for my participants.  Many of them became independent thinkers and knowers about PBL and feel so much more knowledgeable and prepared for the fall.    Part of the class time is spent in “mock PBL class” where I am the teacher/facilitator and they are the students doing problem presentations.  We then sit and talk about specific pedagogical questions and distinctions in classroom practice.  Some of the class time is spent in challenging problem solving which is where I also learn so much from the participant’s different perspectives. “We win when we realize there’s always something we can do better in the classroom” – as Ernst and Hodge write.

The now Infamous ‘French Garden’ Problem

I want to give a huge shout out to all of my participants from last week and encourage them to keep in touch with me.  Many of you wrote in your evaluations that you still have many questions about your practice and how to integrate your vision of PBL in your classroom.  I will always be only an email away and hope that you continue to question your practice throughout the year.

My plan is to try to write some blog posts at the end of the summer/beginning of the year in order to respond to some of the remaining questioning while you plan for the beginning of the school year such as:

  1. How to plan for week one – writing up a syllabus, creating acceptable rules
  2. Helping students who are new to PBL transition to it
  3. Assessment options – when to do what?
  4. Working hard to engage students who might not have the natural curiosity we assume

If you can think of anything else that you might find helpful, please post a comment or send me a message and I’d be happy to write about it too!  Thanks again for all of your feedback from the week and I look forward to further intellectual conversation about teaching and PBL.

Anja S. Greer Conference 2013

What a great time we had this week in my courses!  I am so excited by all of the folks that I met and the CwiC sessions of other leaders that I went to.  Pretty awesome stuff presented by Maria Hernandez from NCSSM, my great colleague Nils Ahbel, Tom Reardon, Ian Winokur, Dan Teague, Ken Collins and many others.  I was so busy that I didn’t get to see many other people’s sessions so I feel somewhat “out of it” unfortunately.

I want to thank everyone that came to my CwiC’s and remind them to be sure to go and pick up my materials on the server before they leave.

For my participants – here are the links to the course evaluations:

Moving Forward with PBL: Course Evaluation

Scaffolding and Developing a PBL Course:  Course Evaluation

PBL – Students making Mathematical Connections

As someone who has used Problem-Based Learning for almost 20 years and sad to say has never been part of a full-fledged Project-Based Learning curriculum, what I know best is what I call PBL (Problem-Based Learning).  I know there is a lot of confusion out there is the blogosphere about what is what, and with which acronyms people use for each type of curriculum.  I did see that some people have been trying to use PrBL for one and PBL for the other, but I guess I don’t see how that clarifies – sorry.

So when I use the acronym PBL in my writing I mean Problem-Based Learning and my definition of Problem-Based Learning is very specific because it not only implies a type of curriculum but an intentional relational pedagogy that I believe is needed to support learning:

Problem-Based Learning (Schettino, 2011) – An approach to curriculum and pedagogy where student learning and content material are (co)-constructed by students and teachers through mostly contextually-based problems in a discussion-based classroom where student voice, experience, and prior knowledge are valued in a non-hierarchical environment utilizing a relational pedagogy.

Educational Psychologist and Cognitive Psychologists like Hmelo-Silver at Rutgers University have done a lot of research on how students learn through this type of scaffolded problem-based curriculum dependent on tapping into and accessing prior knowledge in order to move on and construct new knowledge.  There was a great pair of articles back in 2006/2007 where Kirschner, Sweller & Clark spoke out against problem- and inquiry-based methods of instruction and Hmelo, Duncan and Chinn responded in favor.  I highly recommend reading these research reports for anyone who is thinking of using PBL or any type of inquiry-based instruction (in math or any discipline).  It really helps you to understand the pros and cons and parent and administrator concerns.

However, after you are prepared and know the score, teachers always go back to their gut and know what works for their intuitive feeling on student learning as well.  For me, in PBL, I look at how their prior knowledge connects with how, why and what they are currently learning.  One of the best examples of this for me is a sequence of problems in the curriculum that I use which is an adaption from the Phillips Exeter Academy Math 2 materials.  I’ve added a few more scaffolding problems (see revised materials) in there in order to make some of the topics a bit fuller, but they did a wonderful job (which I was lucky enough to help with)and keep adding and editing every year. The sequence starts with a problem that could be any circumcenter problem in any textbook where students use their prior knowledge of how to find a circumcenter using perpendicular bisectors.

“Find the center of the circumscribed circle of the triangle with vertices (3,1), (1,3) and (-1,-3).”

Students can actually use any method they like – they can use the old reliable algebra by finding midpoints, opposite reciprocal slopes and write equations of lines and find the intersection points.  However, I’ve had some students just plot the points on GeoGebra and use the circumcenter tool.  The point of this problem is for them to just review the idea and recall what makes it the circumcenter.  In the discussion of this problem at least one students (usually more than one) notices that the triangle is a right triangle and says something like “oh yeah, when we did this before we said that when it’s an acute triangle the circumcenter is inside and when it’s an obtuse triangle the circumcenter is outside.  But when it’s a right triangle, the circumcenter is on the hypotenuse.”

Of course then the kid of did the problem on geogebra will say something like, “well it’s not just on the hypotenuse it’s at the midpoint.”

 

Dicussion will ensue about how we proved that the circumcenter of a right triangle has to be at the midpoint of the hypotenuse.

A day or so later, maybe on the next page there will be a problem that says something like

“Find the radius of the smallest circle that surrounds a 5 by 12 rectangle?”

Here the kids are puzzled because there is no mention of a circumcenter or triangle or coordinates, but many kids start by drawing a picture and thinking out loud about putting a circle around the rectangle and seeing they can find out how small a circle they can make and where the radius would be.  When working together oftentimes a student see a right triangle in the rectangle and makes the connection with the circumcenter.

A further scaffolded problem then follows:

“The line y=x+2 intersects the circle  in two points.  Call the third quadrant point R and the first quadrant point E and find their coordinates.  Let D be the point where the line through R and the center of the circle intersects the circle again.  The chord DR is an example of a diameter.   Show that RED is a right triangle.”

Inevitably students use their prior knowledge of opposite reciprocal slope or the Pythagorean theorem.  However, there may be one or two students who remember the circumcenter concept and say, “Hey the center of the circle is on one of the sides of the triangle.  Doesn’t that mean that it has to be a right triangle?”  and the creates quite a stir (and an awesome “light bulb” affect if I may say so myself).

A few pages later, we discuss what I like to call the “Star Trek Theorem” a.k.a. the Inscribed angle theorem (I have a little extra affection for those kids who know right away why I call it the Star Trek Theorem…)

I will always attempt to revisit the “RED” triangle problem after we discuss this theorem.  If I’m lucky a student will notice and say, “Hey that’s another reason it’s a right triangle – that angle opens up to a 180 degree arc, so it has to be 90.”  and then some kid will say “whoa, there’s so many reasons why that triangle has to be a right triangle”  and I will usually ask something like, “yeah, which one do you like the best?” and we’ll have a great debate about which of the justifications of why a triangle inscribed in a circle with a side that’s a diameter has to be right.  So who are the bigger geeks, their teacher who names a theorem after Star Trek or them?

References:

Kirschner, P. A., Sweller, J., & Clark, R. E. (2006). Why minimal guidance during instruction does not work: An analysis of the failure of constructivist, discovery, problem-based, experiential and inquiry-based teaching. Educational Psychologist, 41(2), 75-86.

 

Hmelo, C. E., Duncan., R.G., & Chinn, C. A. (2007). Scaffolding and achievement in problem-based and inquiry learning: A response to Kirschner, Sweller and Clark (2006). Educational Psychologist, 42(2), 99-107.

 

Creating a Conspiracy in the PBL Classroom

Any Mad Men fans out there?  I just love some of the characters and the struggles they put themselves through.  In one episode from season 5, called “Signal 30,” Lane Pryce needs to take some clients out to dinner and Roger Sterling is giving him some advice on how to woo them to sign a contract with Sterling Cooper Draper Pryce.  Since he is not an account man, Lane is nervous about landing the account.

Roger:  And then it’s kind of like being on a date.

Lane:  Flattery, I suppose…

Roger:  Within reason, but I find it best to smile and sit there like you’ve got no place to go and just let ‘em talk.  Somewhere in the middle of the entrée, they’ll throw out something revealing and you want to wait ‘til dessert to pounce on it. You know, let him know you’ve got the same problem he has.  Whatever it is, and then you’re in a conspiracy – the basis of a quote “friendship”.  Then you whip out the form.

Lane:  What if I don’t have the same problem?

Roger:  It’ll probably be something like he drinks too much, he gambles…I once went on a five minute tear about how my mother loved my father more than me and I can assure you, that’s impossible.

Lane:  Very good then, and if for some reason he’s more reserved?

Roger:  You just reverse it – feed him your own personal morsel.

Lane:  Oh I see.

Roger: (getting up to leave) That’s it, get your answers, be nice to the waiter and don’t let him near the check.

My husband and I watched this episode about the same time I was having a great deal of resistance in my class to PBL.  I was talking to my husband about how to get students to buy into the notion of learning for the sake of learning where everywhere else in their lives what measures their learning are their grades.  Why would I expect anything different from them if this is the culture they were brought up in?  They depend on their grades to get them into a good college and if their grades are not up to a certain standard, they will not “measure up.”

I get this question all the time from other teachers – about  how to motivate students to find the love of learning and the interest in problems when they do not necessary know the solution methods to find them.  I usually tell them the same things – talking about the values of the class, grading class contribution with a viewable rubric,  grading their metacognitive journal writing, rewarding them with an interesting relationship with a great teacher…OK that might be pushing it.

However, this year is different.  I am having the hardest time trying to let them know what I want from them.  They do the homework, try their best, write down notes, but for some reason it feels different.  It’s almost as if there’s this wall between them and me and I don’t know how to get them to see my side.  I have had this problem with students in the past, but usually with a whole class.  Some of them blatantly are interrupting each other and others are obviously ignoring each other.

Then my husband says, “Maybe it’s like the conspiracy.”  I said, “What?” He said,” You know, what Roger was talking about on Mad Men.  Now, Roger Sterling is no saint (those of you who watch the show know this all too well) and I usually take what he says with a grain of salt.  I also would not ever consider taking advice from him, especially about teaching, but I allowed my husband to continue.  He said maybe what I had to do was build up the conspiracy that Roger was talking about.  I had a real problem with that because I am so committed to relational pedagogy that there’s no way I could lie to or mislead a student about their learning.  But that’s not what he really meant.

I suddenly realized that what had happened was I was teaching a curriculum that I didn’t even buy into.  I had just finished teaching them matrices and matrix operations with some problems that I had written, and it went very well.  However, in the end I did have to do Cramer’s Rule and determinants.  I tried motivating the problems about determinants with the area of a parallelogram, which kept them interested for a while, but in the end, with a 3×3 it was just here’s the way to do it.  I’m not sure that I could’ve expected them to have enough prior knowledge to derive the formula for finding a determinant of a 3×3.  As much as I tried to cover it up with problem-based learning, it was still a curriculum that is antiquated and not necessarily what I felt they should be doing and learning.  I couldn’t hide it any longer.

But we’re caught aren’t we?  Do we change the whole system – college prep curriculum, SAT required math, college expectations – and if so how do we do that? (see ahbel.com for a great article on this and a keynote address called Reflections on a 119 year old curricullum!)  Do we move beyond the required standardized testing material and allow our students to see mathematics the way we see it?  Yes, that’s the conspiracy – that’s what my husband was talking about.  When kids complain to me, I will “smile and sit there while they talk” knowing that I’m going to try to get something that we have in common.  “Do you hate solving a system of three equations with three unknowns with a determinant? Oh yeah, I did too in high school.  Wouldn’t it be great if we could do something else?  What else should we do?  Let me find some other problems that might be interesting.”  We have the same problem (literally and figuratively), now we’re on the same playing field having similar motivating factors.

And you know what?  I don’t think it would be the end of the world if they’re not revealing and you reversed it.  We are allowed to say to them that we don’t understand why we are still teaching this and these would be my reasons for taking it out of the curriculum – part of your own personal morsel.  It might actually bring you closer as a class and have you talking about how your hands are tied and we have to get through this “together.”

Yeah, there are little tricks that can be learned and carrots that can be used to get students to do what you want them to do, but in PBL, that’s not the point.  There is very little for them to mimic because it is based on their prior knowledge.  They are the ones who need to move the curriculum forward.  So in a nutshell,

  1. Take action – Get to problems in order for students to start feeling empowered and active in class.  Once they see that they are capable of a great deal on their own, it is amazing what they can accomplish.
  2. Create relationships – be sure that you are being reciprocal in your attempts at problems and valuing theirs.  The concept of Relational Trust and Authority are huge parts of a PBL pedagogy (Boaler, Bingham)
  3. But make sure that you are at least somewhat in control in the end because we are, at least for now, still responsible for making sure that some understanding of what we might consider unnecessary skills, for their next courses or future use.

As Roger said, “Get your answers, be nice to the waiter and don’t let ‘em near the check.”  Create that conspiracy.

 

 

 

The Role of Technology in Relational Pedagogy?

So I’ve been thinking a lot lately about technology and learning.  There’s so much in the news about MOOCs, using iPads, schools using technology, etc.  I am even part of a pilot program at my school right now where all of my students have iPads in my honors geometry class and we are trying to communicate at night using Voicethread and the iPads.  My hope was that having a way to share ideas during the evening would lessen the stress of homework problems that students are asked to grapple with in the PBL curriculum would give them more opportunities to throw out problem-solving ideas with each other before class starts so that we would spend less time in class debating different methods of solving the problem (although that’s what I love about class, right?).

But I’m asked as a teacher to find ways to integrate technology into my classroom – but to what end?  I want to find ways to use technology to solve problems, to explore ideas and to help improve students’ understanding of the mathematics.  Not necessarily help them communicate with each other, which is what I’m finding most of the apps out there are for right now – which I am open to – but they are removing a huge part of the learning triangle.  In fact, David Hawkins (1974) wrote about the I-thou-it reciprocal relationships in learning that simply must exist between the learner, the teacher and the subject matter.  He said that if one of the relationships is hindered or dysfunctional in some way, that learning is not optimal.

Hawkins (1974)

So if I interrupt that relational triangle between the students’ communication with the material (and with each other) and with me, using technology instead of discussion and the connection with all three, my fear is that learning is not optimal.  Perhaps the technology could enhance it, but for now I see that it is not truly happening.  My guess is that it has to take time for the students maybe to want for that to happen.

I also just read an article on Edutopia by a guy named Matt Levinson that was entitled “Where MOOCs Miss the Mark: The Student-Teacher Relationship” where it was stated that a lack of mentorship, close guidance or meaningful relationship between teachers and students is what is really lacking in these online courses. Even students who use Khan Academy lectures for “learning” sometimes comment that even though they don’t like sitting and listening to lectures in math class, they would “much [prefer] listening to her math teacher explain the same concepts because she likes this teacher and feels comfortable asking questions and going for extra help outside of class.”

Carol Rodgers (one of my most favorite people on earth) writes about teacher presence and the importance of it in the classroom.  I believe in mathematics class and especially the problem-based mathematics class it is truly essential because in order for students to take a risk with a method, they need to feel supported and safe in order to be open to new ideas and to discuss them with others.  With the open presence of a teacher and mentor, students are not “receiving knowledge” but creating it with others – creating it within those relationships that Hawkins was talking about – maybe with technology or without it.  But  for someone who just spent two years writing about the importance of relational pedagogy in PBL, I find it extremely difficult to assume that without those relationship the same exceptional amount of learning would go on.

Doing What You Can

I just got back from a great visit to Toronto (which was also my first visit to that wonderful city.)  I spoke at a conference and also did some work at beautiful girls’ school there that was interested in PBL.  It was the first time where two of my research interests intersected (Gender and PBL) and it was fascinating for all that were involved – quite wonderful and so much fun.

Many teachers talked to me there (and it comes up everywhere I go) about the fact that they are the only teacher, or one of the few, at their school that is interested in  trying out this different method of teaching, but need to keep up with the syllabus that their colleagues are using in order for students to be prepared for the common exam either at the end of the term, the end of the semester or even on a monthly basis.

This can be problematic when there are school districts that dictate down to the homework assignment or classroom activity that you need to be doing on a daily basis.  The free that classroom practitioners need to make decisions about what is best for the learning of their students is quite important.  However, it is still possible to integrate problem solving or methods of PBL into your classroom when you can get.

I talk about the Continuum of PBL when I give workshops to let people know that you don’t have to dive in head first if you want to try your hand at classroom discourse a little at a time.  Learning to facilitate discussion as a long-time direct instruction teacher is actually quite difficult to allowing students to have more authority can be tricky.  Here is the visual aid that I use when I discuss it:

“A Continuum of PBL” cschettino 2013

The arrow tells you the level of decompartmentalization of topics – in other words how the topics are blended together or not.  In a traditional mathematics curriculum, a textbook artificially separates mathematics into what I like to think of a “compartments” that in a very linear order and most students learn to believe that mathematics must be taught in that order.  Yes, some operations and skills must come before others, but conceptually a great deal of mathematics can be learned or thought about in no particular order.  It is all extremely and equally fascinating.  In a whole problem-based curriculum such as what the faculty at Phillips Exeter Academy has written there are no chapters that mark the ending of the content and the beginning of another since there truly no time when that content is no longer applicable to the new material that is being learned (yes, of course that is true in a text book as well, but the chapter alone have come to imply that to students).

Decompartmentalization can come at different levels.  At the lowest level,many  teachers use “Problems of the Day” that challenge students at the beginning of class with logic puzzles, topics they are not seeing regularly or interesting tidbits like soduku or other fun activities to get students’ minds working.  These create discussion and allow them to see problem solving in action.  However, there is little connection to the mathematics that is being learned in the class proper.

I won’t discuss every type of PBL on the continuum or this will turn into one of my hour long talks, but I will say that if you are interested in attempting to keep up with your colleagues who are following a traditional syllabus but you might want to use less direct instruction I have a link to my “Motivational Problems” page in order for you to have them start the conversation based on their prior knowledge of material.  The problems are listed by topic and you can have them move forward in class by presenting problems and then have them practice with problems in the textbook.  Anyone who tries this – I’d love to hear from you!

This was how I first started at my last school and it worked well for me.  You can read my article from 2003 in the Mathematics Teacher to learn what it was like.  But it definitely moved me in the right direction.  Keep pushing on!

A New Year: Setting Up the Dialogue

As the new school year approaches, I’m re-editing, once again, my PBL text that has been a “work in progress” for about seven years now. Every year my colleagues and I at my old school would take the input from our department and the students in the course and improve upon the work. This is what the teachers at Phillips Exeter do every year to their original materials as well. I think the idea of the problem sets being organic and dynamic is really the only way to think about problem-based learning – to believe that you can learn as much from the students and how they view the problem as they can learn from the problems themselves. In fact, while cleaning out some old folders this summer I ran across this quote, which I believe, is from Freire:

“The problem-posing method does not dichotomize the activity of the teacher-student: she is not ‘cognitive’ at one point and ‘narrative’ at another. She is always ‘cognitive,’ whether preparing a project or engaging in dialogue with the students. He does not regard cognizable objects as his private property, but as the object of reflection by himself and the students. The students – no longer docile listeners – are now critical co-investigators in dialogue with the teacher. The teacher presents the material to the students for their consideration, and re-considers his earlier considerations as the students express their own. “

Pretty amazing the way he’s got it right there, I think. That once you put that problem out there, it is no longer yours, but everyone’s to work with and the students need to be part of the responsibility for the learning. It is presented to them “for their consideration” you must reconsider your earlier consideration once they express theirs. That’s the deal you make when you use PBL – that you will do those reconsiderations. It’s part of the pact.

However, the kids need to be part of the pact too. Wait, let me back up. So, I’m sitting at my computer typing and my son, who is going into ninth grade geometry this fall, asks me what I’m doing. I tell him and editing my geometry textbook for my class for the fall. He asks me if he’ll be using a book like that – that’s not a “normal” textbook. I tell him, I don’t think so – I think his school uses a traditional geometry textbook that pretty much will give him direct instruction in the classroom much to my dismay. And he says to me with a sigh of relief, “Phew…well, that’s just fine with me.”

Of course, I’m thinking…whoa, hold the phone. Have I failed as a parent? Have I not instilled any intellectual curiosity in my son at all that he wouldn’t want to have some type of investigation going on in his mathematics classroom? I also had a very interesting experience starting at a new school this past year that traditionally had mathematics classrooms that were taught with direct instruction. It definitely took some time for students to get used to the idea of a teacher that did things a little differently. Student expectations for being “given” knowledge were extremely high and my expectations for them to construct knowledge were extremely high. It was an interesting situation.

Anderson (2005) found that many teachers who taught with PBL-type pedagogies found reluctance and resistance in students for lots of reasons. Even though they enjoyed the classroom more and even learned better in the long run, there a few downsides. Because of the habits of mind that students have formed in traditional classrooms they do not feel they are being “successful” unless the known authority figure (a.k.a. the teacher) is telling them they are right or wrong. The typical “received knower” that many students are in American classrooms today have “grown accustomed to learning in a classroom that required little from [them] in terms of engagement with mathematics” and they find it difficult for themselves to take responsibility and control for their learning in the way PBL asks them to. What can even happen sometimes is that these kids who are resistant can glom onto a student that seems to take on the attributes of the teacher or authority figure (in their perception) and a small group can become a microcosm of the traditional classroom if a teacher is not careful.

However, from working with teachers for many years and my own personal experience, students are actually very adaptable. Spending time in the classroom with this type of learning, students learn to adjust their own expectations and realize how much of a give and take there is – how much support to expect and learn that they are pleasantly surprised by what they can accomplish on their own.

I’d like to think that even my own son would be proud of himself if give the opportunity and I might give him some problems outside of class this year just to see what he does with them. However, it is just that pact that I was referring to before that the students have to buy into. If they don’t do their share and express their considerations on the problems, there is no dialogue to reflect on, there is no sharing that has gone on. And there enlies the rub – you are back to square one with direct instruction. So I’ve told myself that at the beginning of the year this year, I’m making that part perfectly clear that they have just as much say in the dialogue about what we’re learning and I hope they get the point – at least faster than they did last year!

What’s the “P” in PBL?

One of the issues I talk about a lot with people who are interested in Problem-Based Learning is the “continuum” of integration that I use to tell people how they can implement it in their classroom. How do you want to incorporate the teaching with problems in your classroom? Magdalene Lampert wrote a wonderful book called Teaching Problems and the Problems with Teaching in which she chronicled her journey of teaching a fifth grade classroom for a year with problems (it’s an awesome book, BTW). The way in which you use the problems, the pedagogy you use, and the classroom community you set (the lack of hierarchy, the authority you allow the kids to have, the safety of the risk-taking, etc.) is all hugely important parts of the PBL environment. I found another “continuum” created by someone name Peter Skillen and a colleague named Brenda Sherry. Mr. Skillen, a lifelong educator from Toronto, Canada, doesn’t claim to be an expert in PBL, but has extensive experience in the world of education and has great ideas. Check out his blog if you have time.

He created a wonderful Continua to Consider for Effective PBL which I believe is definitely worth sharing. Although his “P” in PBL is Project, I believe his Continua (since there is more than one scale) is just as applicable to Problem-Based Learning. It reminds me a great deal of what I use in terms of implementation. He also has stated that anyone who would like to add categories should feel free, and I might actually work with that. His categories to consider are

Trust
Questioning
Collaboration
Content
Knowledge
Purpose

These are amazing to start off with and I would probably add a few more to those including authority (although, I think this is what he’s getting at with trust and locus of control) and perhaps also change the “collaboration” one a bit. It is pretty tricky – this idea of interdependent, independent or dependent learning – dependent on what? The teacher, other students, a textbook? Very complex ideas at stake here. Different types of PBL are being considered and in different frameworks. But what he and his colleague have put together is amazing start to an important discussion.

In fact, it’s really important to decide what you mean my “PBL” is? Even on the public shared website for the American Education Research Association Special Interest Group for PBL there is a “Statement on Nomenclature” about what PBL might be interpreted as meaning. There is an acceptance that there is more than one, and in fact many, meanings for the acronym “PBL” and what one person thinks it is may not be the same as another. I am very open to the understanding that when some contacts me about their own school’s interest in using PBL, I have many questions for them before we start talking about implementation.

Not to belabor the great article by David Jonassen that was published in the Interdiscipliary Journal of Problem-Based Learning, (see my other blogpost Worked Examples in PBL) but I really like the distinction he makes between Project-Based and Problem-Based Learning. What it comes down to for him really is the authenticity of the problem. It’s not really about how many, what kind, or how big the problems are that you have the students do. What it is about how did you plan (or not plan) the problems. He is calling it the difference between “emergent authenticity” and “preauthentication.” (definitions by Jonassen (2011). Supporting Problem Solving in PBL. Interdisciplinary Journal of Problem Based Learning 5 (2)).

Emergent Authenticity is when “problems occur during practice within a disciplinary field by engaging in activities germane to the field.” In other words, this is more like when you pose a problem to the students that is something that a mathematician might encounter in real life and an answer is truly unknown (like in real life!!) and they are engaging in that activity of not really knowing that answer and grappling with finding the tools and resources that they need to move forward to find a solution. That is when the authenticity of the problem (or project) is actually emerging as authentic.

Preauthentication is “analyzing activity systems and attempting to simulate an authentic problem in a learning environment.” In high school mathematics classes, this when the teacher knows they want their students to learn something specific from engaging in a specific type of problem or series of problems (mostly like what I do in my curriculum, honestly) and they “set up” a problem-solving situation, but make the kids think that it’s novel. The learning experience has already been analyzed by the teacher and the teacher is giving the students the authority to do the problem at their own pace and draw conclusions, struggle on their own. However, there is some control because it is really only a “simulation” and the teacher actually has more information that can be helpful in terms of learning outcomes, etc. The authenticity has already been “preauthenticated” so that it simulates the experiences of a mathematician as much as possible, but still has the learning outcomes, goals or desired content objectives that might need to be fulfilled.

Which is better? I don’t believe there is a “better”. I believe there is what works for your school, pedagogical beliefs, student audience, teaching style, etc. All of these wonderful categories are what must be considered when you and your department start on the journey towards incorporating PBL into your curriculum. There are many great choices to be made, but it is a long journey and cooperation with lots of reflection are definitely needed. So much to consider.