Category: Exeter

Documents for CwiC Sessions at Anja Greer MST Conference 2016

Instead of passing out photocopies, I tried to think of a way that participants could access the “hand-outs” virtually while attending a session.  What I’ve done in the past a conferences is have them just access them on their tablet devices.  You can also go and access copies on the Conference Server if you do not have a device with you (you should be able to use your phone too).

These link to This is an Adobe Acrobat Documentpdf documents that I will refer to in the presentation about “Assessment in PBL”

Information on Spring Term Project and Spring Term Project Varignon 2015 (this document includes rubric)
Keeping a Journal for Math Class
Revised Problem Set Grading Rubric new
Rubric for Sliceform project and Sliceforms Information Packet
Weekly-Learning-Reflection-Sheet

Page at my website with Rubrics and other guides for Assessment

One of the Original “Makers”

Apologies to any faithful readers out there – I have had a heck of a summer – way too much going on.  Usually during the summer, I keep up with my blog much more because I am doing such interesting readings and teaching conferences, etc. (although I’m running a conference for the first time in my life!) However, this summer I was dealing with one of my biggest losses – the passing of my father after his 8 year battle with breast cancer.  I thought I would honor him by writing a post talking about a problem that I wrote a few years ago, well actually a series of problems that utilized his work when teachers of algebra I asked me how I taught the concept of slope.  So dad, this one’s for you.

In 1986, my dad, Francesco (Frank) Schettino, was asked to work on the renovations for the centennial project for the Statue of Liberty.  He was a structural steel detailer (also known as a draftsman) but he was really good at his job.  Everywhere we went with my dad when I was younger, he would stop and comment about the way buildings were built or if the structure of some stairs, windows or door frames was out of wack.  He could tell you if something was going to fall down in 10 years, just by looking at it.  At his wake last week, one of the project managers from a steel construction company that he worked on jobs for told me that they would save the interesting, most challenging jobs for him because they knew he would love it and do it right.
photo (1)I remember sitting with my dad at his huge drafting desk and seeing the drawings of the spiral stairs in the Statue of Liberty.  He talked to me about the trigonometry and the geometry of the circles that were necessary for the widths that were regulated for the number of people that they needed to walk up and down the stairs.  This all blew my mind at the time – that he needed to consider all of this.  So to be able to write problems that introduce slope to students about this was just a bit simpler to me.

If you take a look at my motivational problems on slope and equations of lines I believe it’s numbers 2 and 3 that refer to his work (excuse the small typo).  Over the years I’ve meant to go back and edit these a number of times.  If you are someone who has taken my course at the Anja S. Greer Math, Science and Technology Conference at Exeter, you are probably familiar with this series of questions because we have discussed these at length and talked about how students have reacted to them (and how different adult teacher-students have as well).  We have assumed no prior knowledge of slope (especially the formula) or the terminology at all.

Some questions that have come up: (with both students and the teacher-students I’ve worked with)

1. What does a graphical representation of “stairs” mean to students?
2. What does “steeper” mean and what causes stairs to be steep?
3.  Why are we given the “average” horizontal run for the spiral stairs? Would another measurement be better?
4. Why does the problem ask for the rise/run ratios?  Is there a better way to measure steepness?
5. (from a teacher perspective) why introduce the term “slope” in #3? can we just keep calling it steepness?

These are such rich and interesting questions. The questions of scaffolding terminology and when and how to introduce concepts are always the most difficult.  Those we grapple with specifically for our own students.  I always err on the side of allowing them to keep calling it steepness as long as they want, but as soon as we need to start generalizing to the abstract idea of the equation of the line or coming up with how to calculate that “steepness” a common language of mathematics will be necessary.  This is also where I take a lesson from my dad in terms of my teaching.  His great parenting style was to listen to me and my sisters and see where we were at – how much did we know about a certain situation and how we were going to handle it.  If he felt like we knew what we were doing, he might wait and see how it turned out instead of jumping in and giving advice.  However, if he was really worried about what was going to happen, he wouldn’t hesitate to say something like “Well, I don’t know…”  His subtle concern but growing wisdom always let us know that there was something wrong in our logic but that he also trusted us to think things through – but we knew that he was always there to support and guide.  There’s definitely been a bit of his influence in my career and maybe now in yours too.

Inspirational colleagues? Wow…

OK, so I’m not really doing the full blog challenge – This weekend was nutso and blogging everyday is really tough – enough with the excuses.  But this question, “Who was or is your most inspirational colleague and why?” just really struck me at my core.  There have been so many, probably for all of us in education, it would be extremely difficult to pinpoint just one who was MOST inspirational.  I continue to be inspired rather regularly by my past professor (now friend) Carol Rodgers (SUNY) who is just one of the most amazing writers, Dewey Scholars and researchers and reflective practice I have ever met.  She is an amazing teacher mentor and has taught me a great deal. Ron Lancaster (OISE – Toronto) continues to show me how to be a true teacher of teachers every time I see him.  Nils Ahbel (Deerfield) and Maria Hernandez (NCSSM) and two of the most passionate mathematics educators I have ever met and every time I speak with them about my practice, I learn something new – period.  If you all ever get a chance to hear any of them speak, I highly recommend it.

I’ve already written about my inspiration and admiration for Rick Parris and the amazing life he led as a an educator, so I won’t go into that again, but I do feel that if I had to name someone who was not only inspiring, a major role model, caring, patient and kind, and truly changed my life, it would have to be Anja Greer.  If there is anyone to whom I have to attribute my work and lifelong love of teaching mathematics with problem-based learning, it would be Anja, mostly because I would not have had the opportunities and the courage to have taken the risks and to work with people who intimidated the heck out of me when I was only 26 years old.  She was a woman at school that had a very male-dominated history and she always spoke up for the students that were underserved and underrepresented.  She gave of herself in every way and gave me a job opportunity in 1996 that changed my life.

In the classroom, she was a teacher, mentor, innovator and amazing administrator.  To watch her handle a room full of very opinionated and argumentative mathematics faculty was amazing – never losing her grace and determination.  She took her time finding the words that she wanted to say and to this day, when I feel that I am pressured to quickly say something I think of her, take a breath, and rethink my words in my head.

The day I met Anja she frankly explained that she had to put a wig on in order to take me to campus because the students hadn’t seen her with her hair so short.  You see, she was battling cancer at the time that she was serving as department chair, implementing a new curriculum and hiring 4 new teachers that year.  The courage she had to “put on that wig” and move through her days for the next few years inspired me so much.  My son was born the year she lost her battle to cancer and she still had the compassion to let me know how happy she was for me that January.

I am so grateful for Anja’s influence on my life and I continue, in her memory, to teach annually at the conference that was named for her.  If I can even remotely come close to influencing another teacher in the way she has for me, I will have just started to repay her.

30-Year-Old Wisdom, Not Recent Rhetoric

Recently, the Exeter Bulletin published an amazing Memorial Minute in honor of Rick Parris just this past week which I believe was wonderfully written.  In it they use a quote that Rick stated back in 1984 which shows his wisdom and insight into student learning of mathematics and the basis of my interest in PBL.

“My interest in such problems is due in part to the pleasure I get from working them myself, but it also stems from my belief that the only students who really learn mathematics well are the ones who develop the staying power and imagination that it takes to be problem-solvers. Such students will have thus learned that being accomplished in mathematics is not simply a matter of learning enough formulas to pass tests; that creative, original thought requires living with some questions for extended periods of time, and that academic adventure can be found in the pursuit and discovery of patterns, more so than in the mere mastery of known formulas.”

In this one paragraph is the whole of what you can find in so many blogposts, writings of so-called “experts” and “thought-leaders” in education nowadays.  I’m not so sure that the recent trend of promoting curiosity, innovation, creativity, perseverance and ‘grit’ are such original ideas.  It just has taken a long time to catch on in any type of mainstream educational jargon.

Rick Parris knew the truth over 30 years ago and led the charge in curriculum writing, pedagogical study, and leadership in student learning.  Always humble, but deeply interested in discussion, he would never shy away from the chance to discuss teaching mathematics and so I was lucky enough to have him as a colleague in the early years of my career.  He helped form my teaching philosophy and I owe a huge debt to the wisdom he imparted to me.  I seek to help students “live with some questions” every day in my classroom and I join them daily on the “academic adventure” of problem-based learning.  I can only hope that the mathematics community and society as a whole in the U.S. can catch up with his wisdom and we can eventually change the way we view learning mathematics.

A Total Win…with lots of understanding

Before I left for the Anjs S. Greer Math Conference last week, I read an amazing blog entry at the Math Ed Matters website by Dana Ernst and Angie Hodge that was talking about Inquiry-Based Learning and the mantra “Try, Fail, Understand, Win.”  The idea came from one of Prof. Ernst’s student course evaluations this past spring as his student summed up his learning experience in such an IBL course.  This blog post was so meaningful to me because for each of these four words, the authors wrote how we as teachers (and teacher educators) can take this student’s perspective towards our own work.  I decided to attempt to take this attitude going off to my own conference with two courses to give and three smaller talks.  It was sure to be a busy week.

And in fact, it really was.  I had very little time to sit and listen to others’ work, which I really was quite sad about.  However, in my own classes I was so impressed with the amount of enthusiasm and excitement my participants had for PBL and their own learning.  As I sat in front of my computer this morning reading the course evaluations and their tremendously helpful input, it finally occurred to me how truly powerful the experience had been for my participants.  Many of them became independent thinkers and knowers about PBL and feel so much more knowledgeable and prepared for the fall.    Part of the class time is spent in “mock PBL class” where I am the teacher/facilitator and they are the students doing problem presentations.  We then sit and talk about specific pedagogical questions and distinctions in classroom practice.  Some of the class time is spent in challenging problem solving which is where I also learn so much from the participant’s different perspectives. “We win when we realize there’s always something we can do better in the classroom” – as Ernst and Hodge write.

The now Infamous ‘French Garden’ Problem

I want to give a huge shout out to all of my participants from last week and encourage them to keep in touch with me.  Many of you wrote in your evaluations that you still have many questions about your practice and how to integrate your vision of PBL in your classroom.  I will always be only an email away and hope that you continue to question your practice throughout the year.

My plan is to try to write some blog posts at the end of the summer/beginning of the year in order to respond to some of the remaining questioning while you plan for the beginning of the school year such as:

  1. How to plan for week one – writing up a syllabus, creating acceptable rules
  2. Helping students who are new to PBL transition to it
  3. Assessment options – when to do what?
  4. Working hard to engage students who might not have the natural curiosity we assume

If you can think of anything else that you might find helpful, please post a comment or send me a message and I’d be happy to write about it too!  Thanks again for all of your feedback from the week and I look forward to further intellectual conversation about teaching and PBL.

Anja S. Greer Conference 2013

What a great time we had this week in my courses!  I am so excited by all of the folks that I met and the CwiC sessions of other leaders that I went to.  Pretty awesome stuff presented by Maria Hernandez from NCSSM, my great colleague Nils Ahbel, Tom Reardon, Ian Winokur, Dan Teague, Ken Collins and many others.  I was so busy that I didn’t get to see many other people’s sessions so I feel somewhat “out of it” unfortunately.

I want to thank everyone that came to my CwiC’s and remind them to be sure to go and pick up my materials on the server before they leave.

For my participants – here are the links to the course evaluations:

Moving Forward with PBL: Course Evaluation

Scaffolding and Developing a PBL Course:  Course Evaluation

Linking Theory to Practice: A Shout-Out to ‘savedabol’

This past January, I gave a key-note address at the ISOMA conference in Toronto and posted my slides from that talk on my academia.edu site that I thought would be a good place for me to easily give other people access to my work. (along with my website).  Academia.edu is great because it gives you lots of information about the stats of surfers who come and look at your information.  All of a sudden I saw that this powerpoint had more than something like 400 views and I couldn’t believe it.  I had to see who was searching and looking at this slideshow.

I quickly realized that someone had seen it, liked it and posted something about it on reddit.  There were only a few comments but one of them went something like this:

“I think the single worst part of being a teacher is sitting through PowerPoints like this, while some earnest non-classroom pedagogue tells us the bleeding obvious.”

Whooo – that one stung…my first instinct was to try and find out who that person was and defend myself to the ends of the earth.  Anyone who calls me a non-classroom pedagogue deserves to be righted…but then I kept reading…and someone with the alias ‘savedabol’ wrote this:

‘Carmel Schettino (the author) led a seminar I took at the Exeter math conference last summer. She is incredible. I can assure you that she is not a non-classroom pedagogue. She has been in the classroom nonstop for at least 20 years (that I know of). She is particularly scholarly when it comes to PBL and other ed topics, but that doesn’t make her irrelevant to what we do every day. Near the end she gives some great resources.’

I can’t tell you how affirmed I felt by ‘savedabol’ and I want to just let them know how nice that was of them to share their thoughts about my work with them.  I have been in the classroom non-stop since 1990 (except for two terms of maternity leave and one term of a sabbatical when I was a full-time student myself) and I pride myself in researching as much as possible about what I do.

I do wish that the first poster had had the chance to hear me speak instead of jumping to the conclusions they had – and it definitely got me thinking about something that was discussed last year at the PME-NA conference in October 2012.  I was one of maybe just a few people in the special category of math teacher/educator/researcher/doctoral students at this research conference where many of the math research folks were talking about ways in which they could breach the great divide of the theory people (them) and the practice people (us).

For many years I have lived this double life of both theory and practice and I have to say, I love it.  Having just finished up my Ph.D. and teaching full time was probably one of the toughest things I’ve had to do in my life, but having my mind constantly in both arenas has only helped me be a better teacher and a better researcher.

Jo Boaler is a great researcher at Stanford University who is doing great work in outreach between theory and practice this summer by offering a free online course called “How to Learn Math.”  It’s a course for k-12 teachers that is grounded in the most recent research in math education.  What a great idea!  She is sharing some of her wisdom freely online with k-12 teachers who want to spend some time learning about new ideas themselves.  I know I’m in.

In August 2008, the NCTM put together a special Research Agenda Project to work on recommendations for just this cause and you can see their report here.  One of the major recommendations that came out of their work was to not only emphasize the need for communication between researchers and practitioners, but in my view to help them realize that this communication would benefit both parties equally.  We all have something to share with each other and I know that I appreciate every classroom practitioners’ experiences.  I learn something from every teacher that ends up in my workshop every summer and often end up using many of their ideas as they do mine.

So let’s keep supporting each other both in real life and virtually, and realize that often times, the “bleeding obvious” is something that needs to be stated and discussed over and over again to be sure that we are still talking about it with the right people.

PBL – Students making Mathematical Connections

As someone who has used Problem-Based Learning for almost 20 years and sad to say has never been part of a full-fledged Project-Based Learning curriculum, what I know best is what I call PBL (Problem-Based Learning).  I know there is a lot of confusion out there is the blogosphere about what is what, and with which acronyms people use for each type of curriculum.  I did see that some people have been trying to use PrBL for one and PBL for the other, but I guess I don’t see how that clarifies – sorry.

So when I use the acronym PBL in my writing I mean Problem-Based Learning and my definition of Problem-Based Learning is very specific because it not only implies a type of curriculum but an intentional relational pedagogy that I believe is needed to support learning:

Problem-Based Learning (Schettino, 2011) – An approach to curriculum and pedagogy where student learning and content material are (co)-constructed by students and teachers through mostly contextually-based problems in a discussion-based classroom where student voice, experience, and prior knowledge are valued in a non-hierarchical environment utilizing a relational pedagogy.

Educational Psychologist and Cognitive Psychologists like Hmelo-Silver at Rutgers University have done a lot of research on how students learn through this type of scaffolded problem-based curriculum dependent on tapping into and accessing prior knowledge in order to move on and construct new knowledge.  There was a great pair of articles back in 2006/2007 where Kirschner, Sweller & Clark spoke out against problem- and inquiry-based methods of instruction and Hmelo, Duncan and Chinn responded in favor.  I highly recommend reading these research reports for anyone who is thinking of using PBL or any type of inquiry-based instruction (in math or any discipline).  It really helps you to understand the pros and cons and parent and administrator concerns.

However, after you are prepared and know the score, teachers always go back to their gut and know what works for their intuitive feeling on student learning as well.  For me, in PBL, I look at how their prior knowledge connects with how, why and what they are currently learning.  One of the best examples of this for me is a sequence of problems in the curriculum that I use which is an adaption from the Phillips Exeter Academy Math 2 materials.  I’ve added a few more scaffolding problems (see revised materials) in there in order to make some of the topics a bit fuller, but they did a wonderful job (which I was lucky enough to help with)and keep adding and editing every year. The sequence starts with a problem that could be any circumcenter problem in any textbook where students use their prior knowledge of how to find a circumcenter using perpendicular bisectors.

“Find the center of the circumscribed circle of the triangle with vertices (3,1), (1,3) and (-1,-3).”

Students can actually use any method they like – they can use the old reliable algebra by finding midpoints, opposite reciprocal slopes and write equations of lines and find the intersection points.  However, I’ve had some students just plot the points on GeoGebra and use the circumcenter tool.  The point of this problem is for them to just review the idea and recall what makes it the circumcenter.  In the discussion of this problem at least one students (usually more than one) notices that the triangle is a right triangle and says something like “oh yeah, when we did this before we said that when it’s an acute triangle the circumcenter is inside and when it’s an obtuse triangle the circumcenter is outside.  But when it’s a right triangle, the circumcenter is on the hypotenuse.”

Of course then the kid of did the problem on geogebra will say something like, “well it’s not just on the hypotenuse it’s at the midpoint.”

 

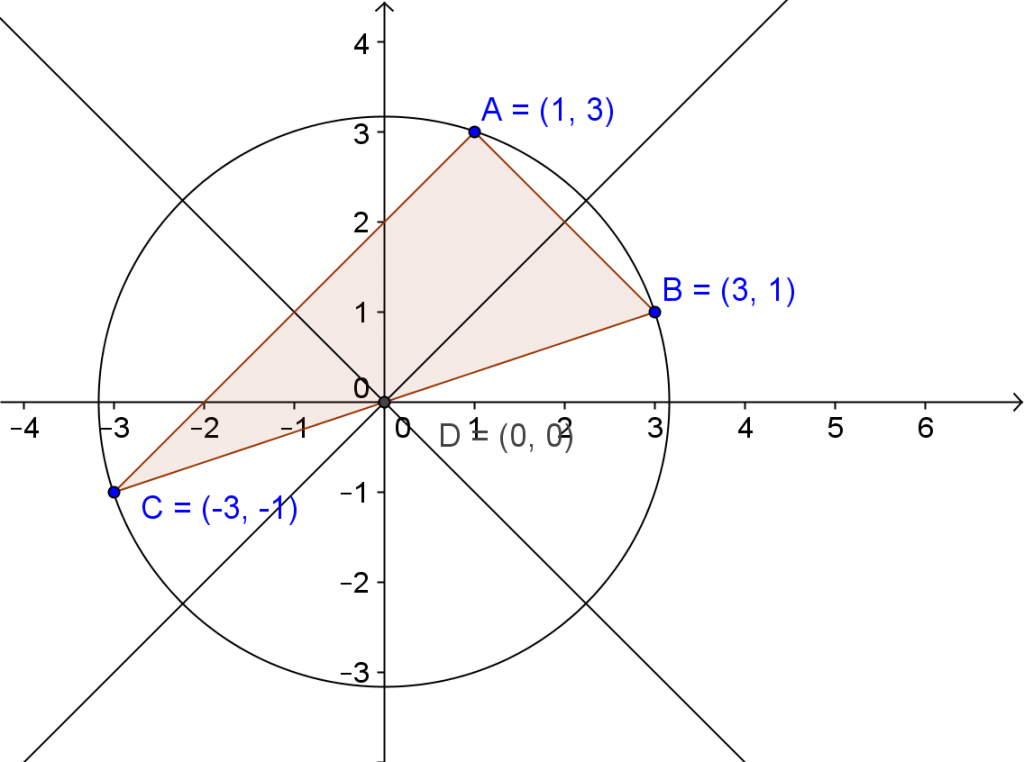
Dicussion will ensue about how we proved that the circumcenter of a right triangle has to be at the midpoint of the hypotenuse.

A day or so later, maybe on the next page there will be a problem that says something like

“Find the radius of the smallest circle that surrounds a 5 by 12 rectangle?”

Here the kids are puzzled because there is no mention of a circumcenter or triangle or coordinates, but many kids start by drawing a picture and thinking out loud about putting a circle around the rectangle and seeing they can find out how small a circle they can make and where the radius would be.  When working together oftentimes a student see a right triangle in the rectangle and makes the connection with the circumcenter.

A further scaffolded problem then follows:

“The line y=x+2 intersects the circle  in two points.  Call the third quadrant point R and the first quadrant point E and find their coordinates.  Let D be the point where the line through R and the center of the circle intersects the circle again.  The chord DR is an example of a diameter.   Show that RED is a right triangle.”

Inevitably students use their prior knowledge of opposite reciprocal slope or the Pythagorean theorem.  However, there may be one or two students who remember the circumcenter concept and say, “Hey the center of the circle is on one of the sides of the triangle.  Doesn’t that mean that it has to be a right triangle?”  and the creates quite a stir (and an awesome “light bulb” affect if I may say so myself).

A few pages later, we discuss what I like to call the “Star Trek Theorem” a.k.a. the Inscribed angle theorem (I have a little extra affection for those kids who know right away why I call it the Star Trek Theorem…)

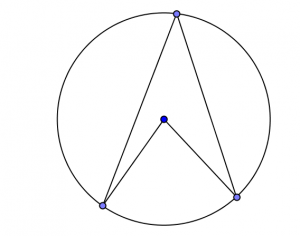
I will always attempt to revisit the “RED” triangle problem after we discuss this theorem.  If I’m lucky a student will notice and say, “Hey that’s another reason it’s a right triangle – that angle opens up to a 180 degree arc, so it has to be 90.”  and then some kid will say “whoa, there’s so many reasons why that triangle has to be a right triangle”  and I will usually ask something like, “yeah, which one do you like the best?” and we’ll have a great debate about which of the justifications of why a triangle inscribed in a circle with a side that’s a diameter has to be right.  So who are the bigger geeks, their teacher who names a theorem after Star Trek or them?

References:

Kirschner, P. A., Sweller, J., & Clark, R. E. (2006). Why minimal guidance during instruction does not work: An analysis of the failure of constructivist, discovery, problem-based, experiential and inquiry-based teaching. Educational Psychologist, 41(2), 75-86.

 

Hmelo, C. E., Duncan., R.G., & Chinn, C. A. (2007). Scaffolding and achievement in problem-based and inquiry learning: A response to Kirschner, Sweller and Clark (2006). Educational Psychologist, 42(2), 99-107.

 

An infinite amount of thanks…

Everyone has those mentors in their life who have impacted their work or career in ways that have truly changed who they are.  In my instance, the person I am going to write about not only has impacted my life and career, but because he taught me so much about great teaching, in particular PBL, he has impacted all of the students and teachers I have worked over my twenty year career so far.  So I feel justified in taking a short break from writing strictly about professional educational work musings and just finding a moment to say thanks for the life and work of Rick Parris.

Even if you never met Rick in his time teaching at Phillips Exeter Academy, or used his wonderful opensource Peanut software for windows machines, or downloaded the faculty-authored materials that he was integral in writing by the mathematics department at PEA – if you have worked with me at all, you have been affected by Rick’s work.  Rick Parris had to be one of the most brilliant, efficient, insightful  mathematicians I’ve ever been lucky enough to work with.  He saw things in a problem that I definitely never would be able to see in a million years.  I was so extremely intimidated by him when I first started working in the same department that I would go for days confused about a problem instead of go up and ask him.  But what I soon found was that not only was he one of the most brilliant mathematicians, I’ve ever met, but he was one of the best teachers too.  Now, there is a rare combination – finding someone who has the insightful intelligence to be able to have a Ph.D. in mathematics but to also be so sensitive to others’ understanding of the subject and the patience and passion to want them to love it as much as he did.

I remember finally having the courage to go and ask him a question about a problem in the 41C materials on fall afternoon (mostly because I knew I had to understand it) and he looked at me, with what I thought was a look of disdain or horror that one of his colleagues wouldn’t understand a problem that he wrote.  And just as I was going to run in shame, he said something like, “that is such an interesting way to look at that” and I was amazed at how good that felt.  He entertained my ideas and although I felt like he was initially just appeasing me, I soon realized that he was truly and sincerely intrigued.  Our relationship as colleagues and interested problem solvers grew, even after I left PEA.  He allowed me to keep in touch constantly asking him questions and posing them over email.  He taught me so much about writing great problems, encouraging students to ask great questions and making sure that they always felt like they were they most interesting questions ever.

This past summer, the last time I saw Rick, we were talking about the game of Set (you know that really fun card game with the colors, shapes and numbers).  We were just posing really fun questions like “What’s the maximum number of sets you can get in a 12 card deal?”  We found these types of questions intriguing and even after we parted company we continued emailing with email subject lines like “a baker’s dozen of sets”, “set lore” and “the game of set redux.”  He always treated me like a real mathematician even though he was the one who I saw as my inspiration and motivation in that area.

Rick taught me about how to scaffold problems (not too much) so that students would see their way through a topic and find out exciting ideas of mathematics on their own.  I loved to watch him teach, probably observing his classes three or four times a year in order to gain insight into his questioning methods.  He made a point of trying to hear from every student in the class at least once a class.  I don’t know if he ever knew how much of an impact he had on my teaching and philosophy of learning.  I am so grateful.

So how do you say thank you to someone who pushed you in a direction that changed your life?  I guess I have just to recommit myself to learning about and researching the best practices of inquiry and problem-based learning in secondary mathematics education.  I do believe that the world needs to know about the contributions of this man and the department at PEA because without them and the model that they have created, I’m not sure that many of the schools today that utilize their curriculum would be where they are.  I give thanks to Rick and consider myself extremely lucky to have worked with him and shared his enthusiasm for problems.

Thank you! Thank you!

What an amazing week I’ve spent in Exeter at the conference. I have met some truly wonderful people, who I feel lucky to call my colleagues in mathematics education. I’ll share some great highlights here.

Monday night we heard Steven Strogatz speak – author of the Calculus of Friendship and honored professor of Applied mathematics at Cornell University. What a touching story (and speaker!) about mentoring, teaching and the reciprocity of learning – I highly recommend the book to any math teacher as it truly captures why we do what we do. There was not a dry eye in the place. At one point I was able to get him to sign my book and he even inquired about what PBL was all about.

Yesterday afternoon I held a Special Interest Group for teachers and others who wanted to discuss issues related to gender equity for girls in STEM fields. I had been surprised to see that the conference director had organized it to be a double session (two hours long), but said, what the heck, we can always end early if people start leaving. Boy, was I surprised when about 20 people came at the beginning and stayed for the whole two hours. We had an amazing and passionate conversation that ranged from questions about best practices for teaching girls in the classroom to sharing personal stories and experiences from science and math education from the participants. I was most surprised to hear from the youngest women educators that biased practices still go on in academia to dissuade women from moving on in advanced degrees.

Everyone’s contributions were so important and I want to thank everyone that came to that session. I learned so much from that discussion, it might have been the highlight of the week for me. (Especially seeing the male teachers that came!) I would reiterate my statement of how important it is to share with our colleagues the message of encouraging girls and merely stating that the problem still exists out there. Creating mentoring programs in our schools is another great idea, as well as showing by role modeling that mathematical women can be strong and feminine.

This may have been the best PEA ASG conference I’ve attended and want to thank all of my own mentors that were here – so many people to be grateful for. Probably the most important is the woman after whom the conference was named – Anjs S. Greer who hired me at Exeter in 1995 – she changed my life and helped make me the educator I am today. I am forever in her debt and continue her work.

Enjoy your summer and thanks again for a great week!