Category: Assessment

What I get out of Student Writing

I have been using journaling in math class since 1996 – which was a really important year in my teaching career for lots of reasons, but it was definitely because I was introduced to the idea of math journals.  Since then I’ve done many different iterations for what my expectations are.  Even this year I did something new where I allowed students to write about errors they made on assessments in order to attempt to compare their assessment problems to what they did on homework in the hope of reflecting on the work pre-assessment for future problem sets.

However, a lot of students still use their journal almost like a problem-solving conversation with me, especially after we have already gone over a problem and they still don’t understand a method.  Here is one I ran across just the other day in my lower-level geometry class and thought it just perfectly expressed some of the goals I am hoping to accomplish with journaling.

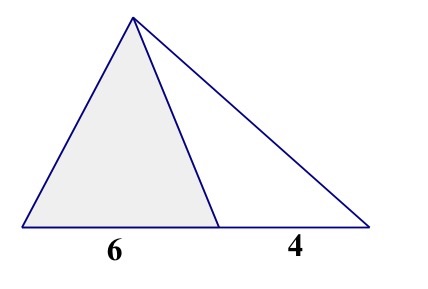
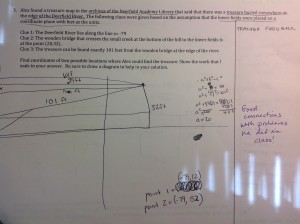
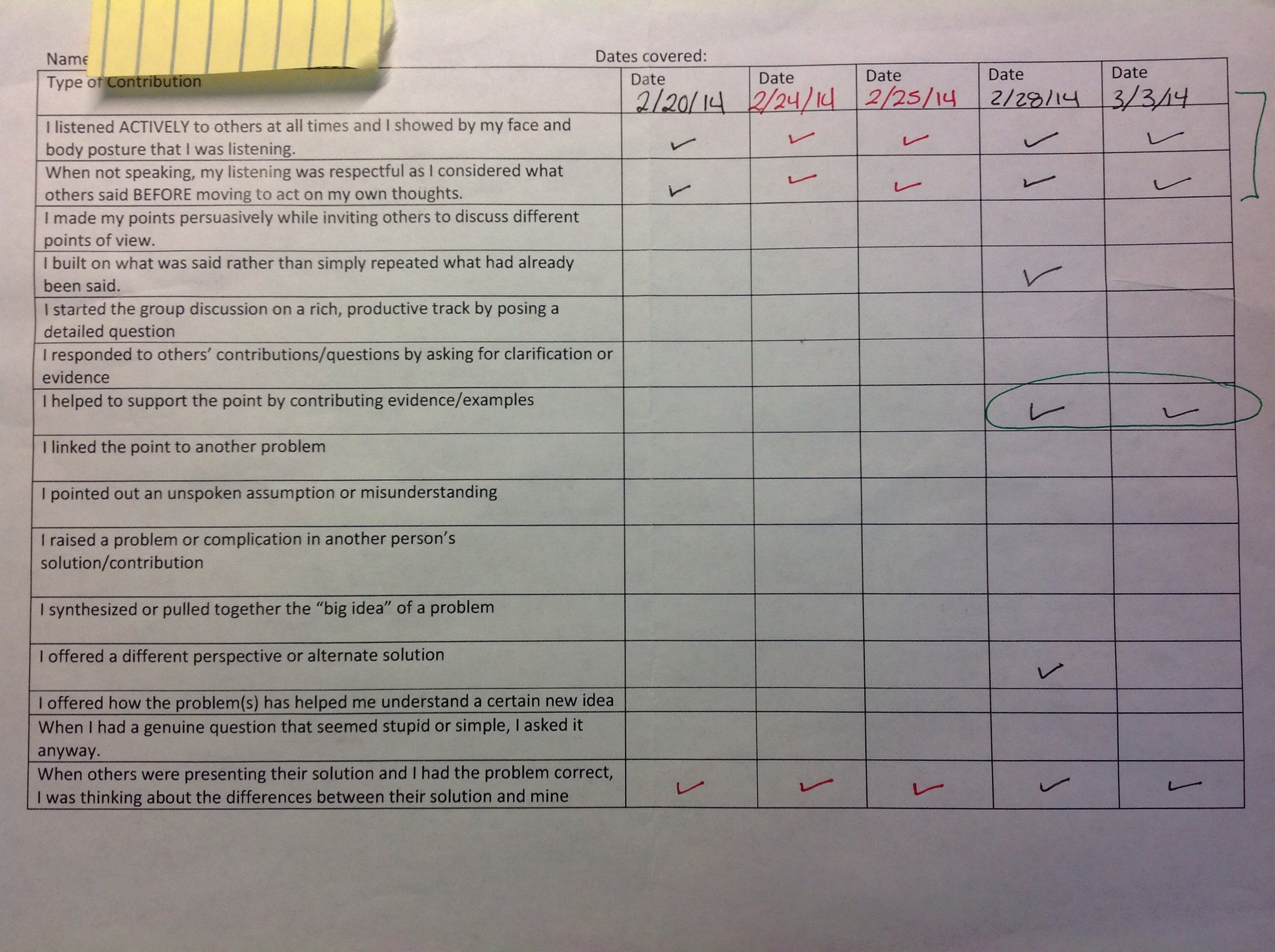
I’ll call this student Cindy and we had just introduced the theorems about parallel lines through a geogebra lab and this had been the first problem they looked at that took the concepts out of the context of the lines and threw it into a triangle.  For many students this might be an easy transfer of skills (including the algebra, other theorems, etc.) but for the kids I have – not necessarily.  Here is what Cindy wrote:file_001-1

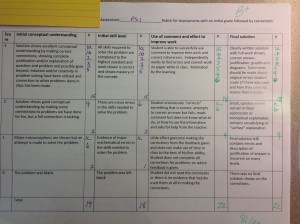
The first thing that Cindy does in her journaling is make her own thinking explicit (which I love).  She is stepping me through her thinking and the questions that arose for her.  This is actually a major step for many students who are confused – are they able to even know what they are confused about?

She writes: “I know the problem probably deals with the parallel line theories that we dealt with.” and then lists the types of angles we studied and then with a big “OR” says “maybe it has something to do with the sum of the angles of parallelograms and triangles?”  Little does she know that what she is doing is practicing synthesizing different pieces of prior knowledge – is it overwhelming to her? – possibly, but she went there and that’s so great!  I wanted her to know that I was excited that that she even thought about the sum of the angles so I gave her some feedback about those ideas.

She wrote down what she knew about the sums of the angles which we had also studied.

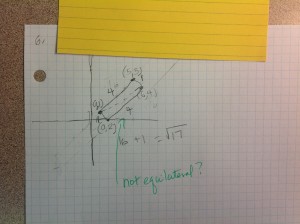
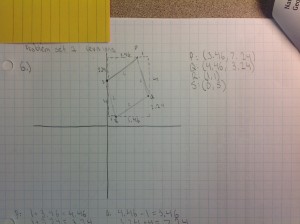
She writes her first equation to think about: “5x-5/=180” using one of the angles in the top triangle.  I would’ve loved to know where that was coming from.  What made her write that?  She then notes that “but it wouldn’t work because if x is the measure of the angle than the equation should be set to 180”

There is so much that this tells me about her confusion.  She is not understanding what the expression 5x-5 is supposed to be representing in the diagram I think, or she isn’t connecting what x is “not representing” (the angle) and the whole expression is representing too.  She also is confused about between the sum of the whole triangle’s angles and just that one angle.

She then looks at the two expressions she is given, 5x-5 and 4x+10 and I think makes a guess that they are corresponding angles – she doesn’t give any reason why they are corresponding.  She just asks the question.  But the cool thing is she says “Let’s try it.”  I love that.  Why not – I am always encouraging them to go with their ideas and the fact that she tries it is wonderful.  The funny thing is she does end up getting the same value for the two angles so she asks: “Does this mean that this is correct?” and then “What do I do for “6y-4?” and still has not connected many of the ideas line the fact that these angles are a linear pair and that’s where the 180 comes into play, or even why the angles were corresponding in the first place.  So many questions that she still has, although I am encouraged by her thinking and risk-taking.

This journal entry allowed me to have a great follow-up conversation with Cindy and she was able to talk to me about these misconceptions.  I’m not sure I would’ve had this opportunity to clarify these with her if she had not written this journal entry and then she would not have done so well on the problem set the following week.  I just love it!  Let me know if you use journals and if you feel the same clarifying or communicative way about them too.

See my website for lots of sample entries and also other blogposts and resources about journaling if you are interested.

 

Journals: Paper vs Digital: The Pros and Cons

5 Comments

I was totally honored the other day when I saw some tweets from TMC16 from @0mod3 and @Borschtwithanna

 

And yes it’s true, I’ve been writing and practicing the use of metacognitive journaling for very long time – probably since 1996 ever since I read Joan Countryman’s book about mathematical journaling and heard about it in many workshops that summer.  I wrote a rubric (make sure you scroll to the 3rd page) while I was at the Klingenstein Summer Institute for New Teachers (that’s how long ago it was) and since then I’ve been refining that rubric based on feedback from students and teachers. A few years ago, I finally refined a document called How to Keep a Journal for Math Class to a degree that I really like it now.  However, please know that lots of math teachers do journaling differently and without the metacognitive twist. I do believe that metacognitive writing is essential to the PBL classroom (read more here)

So this morning, I was asked this question on twitter

 

Which is something that many people often ask so I thought I’d respond with a more in-depth answer.

Here are the pros, I’ve found over the years of having students journal digitally:

Speed/complexity: Students are used to typing, using spell-check, inserting pictures, graphics and naturally including documents, links and thinking in the complex way that digital media allows them to.  It allows their journal to be more rich in content and sometimes connect problems to each other if their journal is say on a google doc that can connect to other html docs.  If they create, for example, iBooks or Explain Everything videos, there is even a lot more richness that can be embedded in the file as well – their creativity is endless.

Grading/Feedback: I found grading in Notability or on Google docs or some other digital platform really nice that allowed you to add comments with a click or audio extremely easy and quick.  I did not receive feedback from the students very often about how the feedback helped them though.  If you use an LMS like Canvas that integrates a rubric or integrates connection to Google it’s even nicer because you can have those grades go right from your assignment book to your gradebook.

I love having kids use digital platforms for writing/creating in mathematics when it is for a project or big problem that I want them to include many pieces of evidence, graphs, geogebra files and put it together nicely in a presentation or portfolio.  Not necessarily for their biweekly journals. Some guys who make use of digital journals in interesting ways are @GibsonEdu and @FrasiermathPBL at the Khabele School in Austin TX.

Here are the cons, in my mind of using digital journals: (which might be the “pros” of paper journals) – which is the side I have come down on.

the “real” writing factor: there is some research about the actual physical process of writing and the time it takes for kids to process their thoughts.  I do believe that when i want kids to be metacognitive about their learning and also want them to be thoughtful and take the time think about their initial error, think about what happened in class discussion to clear up their misunderstanding and also then what new understanding they came to.  That’s a lot of thinking. So I want them to take the time to write all that down.  Sometimes typing (like what I’m doing right now!) is a fast process and I’m not sure I do my best writing this way.

practice in hand-writing problem solving: this is re-enacting doing homework and sitting for assessments (in my class at least) and I want them to do this more regularly.  If in your class kids take assessments digitally or do homework nightly digitally then maybe they should do their journal digitally as well. This also give me practice in reading their handwriting, getting to hear their voice through their handwriting and seeing what it looks like on a regular basis.  In a time crunch on an assessment it honestly helps me know what they are thinking.

Conversational Feedback: I feel that when I hand write my feedback to them I can draw a smilely face or arrows or circle something that I want to emphasize more easily than when it is on something digitally (this is also true in a digital ink program – so that is something to consider, like Notability for example). I give feedback (see some journal examples on my blog) that is very specific about their writing and want the to improve not only in the math aspect of their writing but in how they are looking at their learning.  I want them to respond and I want to respond in the hope that we are starting a mathematical conversation about the problem.  I have received more questions about the feedback in the paper journals (like “what did you mean by this?”) than on the electronic feedback – not sure why.

Portability: I find that small composition graph paper notebook is extremely portable and easy for me to carry home to grade.  The students bring them to their assessments and there is nothing else in the notebook (no homework at all and no access to the internet) so I am not worried about academic honestly.

There are probably more but this is it in a nutshell – please add your comments below or tweet me to let me know your thoughts!

 

Documents for CwiC Sessions at Anja Greer MST Conference 2016

Instead of passing out photocopies, I tried to think of a way that participants could access the “hand-outs” virtually while attending a session.  What I’ve done in the past a conferences is have them just access them on their tablet devices.  You can also go and access copies on the Conference Server if you do not have a device with you (you should be able to use your phone too).

These link to This is an Adobe Acrobat Documentpdf documents that I will refer to in the presentation about “Assessment in PBL”

Information on Spring Term Project and Spring Term Project Varignon 2015 (this document includes rubric)
Keeping a Journal for Math Class
Revised Problem Set Grading Rubric new
Rubric for Sliceform project and Sliceforms Information Packet
Weekly-Learning-Reflection-Sheet

Page at my website with Rubrics and other guides for Assessment

Adventures in Feedback Assessment

On an assessment students did for me today I gave this question:

An aging father left a triangular plot of land to his two children. When the children saw how the land was to be divided in two parts (Triangle ADC and Triangle BDC), one child felt that the division of the land was not fair, while the other was fine with it. What do you think and why? Support your justification with mathematical evidence.

 So this student had a hard time with this question. Since there was no height given and the bases were different, she was unable to think about how to compare the areas. She was however able to say that it would be a fair split if the areas were the same. So since I am doing this work this year with giving feedback first and then grades (see past blogpost “Why teachers don’t give feedback before grades and why they should”) I wrote this feedback on the problem set: 
 I am trying to get her to remember a problem we did in class where there was a similar problem we did with an acute triangle and obtuse triangle that shared the same height:

The area of the shaded triangle is 15. Find the area of the unshaded triangle.

This idea of where the height of obtuse triangles are is a really tough one for some geometry students. But more than that the idea of sharing a height and what effect that has on the area is also difficult.

We will see tomorrow if this student is able to take my feedback and see what whether the division of the land is fair.

By the way, here’s a response that another student had:


Just in case you can’t read it:

“Because the height is the same, it’s the ratio of the bases that would determine which child would get the most land. I think the division of land was not fair, because the heights are the same so therefore the bases are determining the area of the plot. If x=5 then child one would get A=20, child 2 would get 12.5 and that makes the original plot of land 37.5. This means child 2 has a third of the land (12.5:25) (part:part) and half of child 1’s) Even without x=5, the child 2 would only get a third of the land.”

We’ll see what happens!

Someday I’ll get this assessment thing right… (Part 2 of giving feedback before grades)

3 Comments

So, all assessments are back to the students, tears have been dried and we are now onto our next problem set (what we are calling these assessments).  What we’ve learned is that the rubric allowed us to easily see when a student had good conceptual understanding but perhaps lower skill levels (what we are used to calling “careless mistakes” or worse). We could also quickly see which problems many students had issue with once we compared the rubrics because, for example, problem number 6 was showing up quite often in the 1 row of the conceptual column.  This information was really valuable to us.  However, one thing we didn’t do was take pictures of all of this information to see if we could have a record of the student growth over the whole year. Perhaps an electronic method of grading – a shared google sheet for each student or something to that effect  might be helpful in the future – but not this day (as Aragorn says) – way too much going on right now.

We also changed the rubric a bit for a few reasons.  First, we found that when students completed the problem to our expectations on the initial attempt we felt that they should just receive 3’s for the other two categories automatically.  We considered not scoring them in this category but numerically felt that it was actually putting students who correctly completed a problem at a disadvantage (giving them fewer overall points in the end). Second, we also changed the idea that if you did not write anything on the revisions you earned 0 points for the revisions columns.  Many students told me afterwards that they felt like they just ran out of time on the revisions and actually had read the feedback.  This was unfortunate to me since we had spent so long writing up the feedback in the hope that the learning experience would continue while doing revisions.

Here is the new version of the rubric: Revised Problem Set Grading Rubric new

What we decided to do was to try the revisions this time without the “explanation” part of writing.  I think it will keep the students focused on reading the comments and attempting a new solution.  I was frankly surprised at how many students stuck to the honor pledge and really did not talk to each other (as they still got the problem wrong the second time around – with feedback).  Truly impressive self-control from the students in my classes and how they were sincerely trying to use the experience as a learning opportunity.

I do think the second assessment will go more smoothly as I am better at doing the feedback and the rubric grading.  The students are now familiar with what we are looking for and how we will count the revisions and their work during that time.  Overall, I am excited about the response we’ve received from the kids and hope that this second time is a little less time-consuming.  If not, maybe I’ll just pull my hair out but I’ll probably keep doing this!

 

 

 

Why Teachers Don’t Give Feedback instead of Grades, and Why We Should

7 Comments

First in a series of posts about my experiences with “Feedback Before Grades”

Holy Mackerel is all I have to say – Ok, well, no I have plenty more to say – but after about a week and a half of holing myself up with my colleague, Kristen McVaugh, (big shout-out to Ms McVaugh who is not only teaching PBL for the first time but was willing to dive into this amazing journey of alternative assessment with me this year too), I am totally exhausted, almost blind as a bat, partially jaded and crazy – but mostly ready for a drink.  This little looped video of Nathaniel Rateliff and the Night Sweats pretty much sums it up…

So here was our well-intentioned plan:  we wanted to start the year off with a different type of assessment.  I put out my feelers on twitter and asked around if anyone had a rubric for grading assessments where the teacher first gave only feedback and then allowed students to do revisions and then once the revisions were done the students received a grade. Kristen and I knew a few things:

  1. we wanted to make sure the revisions were done in class
  2. we wanted to make sure the revisions were the students’ own work (tough one)
  3. we wanted to give students feedback that they needed to interpret as helpful so that we weren’t giving them the answer – so that it was still assessing their knowledge the second time around
  4. we wanted to make sure that students were actually learning during the assessment
  5. we wanted students to view the assessment as a learning experience
  6. we wanted students to be rewarded for both conceptual knowledge and their skills in the problem solving too

So we created this rubric Initial Draft of Rubric for Grading.  It allowed us to look at the initial conceptual understanding the student came to the problem set with and also the initial skill level. Kristen and I spent hours and hours writing feedback on the students’ papers regarding their errors, good work and what revisions needed to be done in a back-handed sort of way.

Here are some examples:

Student 1 Initial Work
Student 2s initial work
Student 3 initial work

 

Some kids’ work warranted more writing and some warranted less.  Of course if it was wonderful we just wrote something like, excellent work and perhaps wrote and extension question.  The hard part was filling out the rubric.  So for example, I’ll take Student 3’s work on problem 6 which is the last one above. Here is the rubric filled out for him:

Student 3’s Rubric

You will notice that I put problem 6 as a 1 for conceptual understanding and a 2 for skill level (in purple). In this problem students were asked to find a non-square quadrilateral with side lengths of sqrt(17).  Student 3 was definitely able to find vertices of a quadrilateral, but he was unable to use the PT to find common lengths of sides.  I gave him feedback that looking at sqrt(17) as a hypotenuse of a right triangle (as we had done in class) would help a bit and even wrote the PT with 17 as the hypotenuse in the hope of stimulating his memory when he did the revisions.

The day of the revisions Student 3 was only capable of producing this:

Student 3 revisions

He followed my direction and used 4 and 1 (which are two integers that give a hypotenuse of 17, but did not complete the problem by getting all side lengths the same. In fact, conceptually he kind of missed the boat on the fact that the sqrt(17) was supposed to be the side of the quadrilateral altogether.

 

One success story was Student 2.  She also did this problem incorrectly at first by realizing that you could use 4 and 1 as the sides of a right triangle with sqrt(17) as the hypotenuse but never found the coordinates of the vertices for me. I gave her feedback saying there might be an easier way to do this because she needed vertices.  However, she was able to produce this:

Student 2s revision
Student 2s revision

Although she did not give me integer-valued coordinates (which was not required) and she approximated which officially would not really give sqrt(17) lengths it came pretty darn close! I was impressed with the ingenuity and risk-taking that she used and the conceptual knowledge plus the skill-level. Yes, most other kids just used some combination of 1’s and 4’s all the way around but she followed her own thought pattern and did it this way.  Kudos to student 2 in my book.

Next time I will talk about some of the lessons we learned, other artifacts from the kids’ work and what we are changing for next time! Oh yeah and some great martini recipes!

Late night thoughts on Assessing Prior Knowledge

2 Comments

So it’s 11:50 pm on a Tuesday night, so what?  I can still think critically, right?  It was the last day of classes and I had an amazing day, but then all of a sudden Twitter started gearing up and lots of discussions began and my mind started racing.  I had planned on writing a blogpost about a student’s awesome inquiry project (which, it ends up, took me about 2 hours to figure out a way to make an iBook on my iPad into a video to try to post on my blog, so that will have to wait), but then I read a great post by Andrew Shauver (@hs_math_physics)

Mr. Shauver writes about the pros and cons of direct instruction vs. inquiry learning but has a great balanced viewpoint towards both of them. In this post, he is discussing the how and when teachers should or can use either method of instruction.  It is important, Shauver states to remember that “inquiry can work provided that students possess the appropriate background knowledge.”

I would totally agree, but I’m just wondering how we assess that – does it really work to lecture for a day and then say they now possess the appropriate background knowledge?  Do we lecture for two days and then give them a quiz and now we know they possess it?  I wonder how we know?  At some point, don’t we have to look at each student as an individual and think about what they are capable of bringing to a mathematical task?  We should set up the problems so that there is some sort of triggering of prior knowledge, communication between peers, resources available for them to recall the information?

Joseph Mellor makes a great point that in PBL most of the time you might plan a certain outcome from a problem, or set of problems, but the triggering didn’t work, or the kids didn’t have the prior knowledge that you thought.  He says that he is often either pleasantly surprised by their ability to move forward or surprised at how much they lack. In PBL, we depend on the students’ ability to communicate with each other, ask deep questions and take risks – often admitting when they don’t remember prior knowledge – hopefully to no suffering on their part. This can be a big hurdle to overcome and can often lead to further scaffolding, a deeper look at the writing of the problem sequence, fine tuning the awareness of their true prior knowledge (not just what the previous teacher said they “learned”) or yes, maybe a little direct instruction in some creative ways.  However, I do believe that given the opportunity a lot of students can be pleasantly surprising.  What do you think?

Spring has Sprung – and so has the French Garden!

So the spring term means two things for my Honors Geometry kids – the technology inquiry project and looking at the French Garden Problem.  So for those of you who are not familiar with both of those I’ll try to quickly fill you in while I talk about how they just happen to so coolly (is that an adverb?  if not I just made it up) overlapped this week.

My Spring Term Technology Inquiry Project is something I came up with three years ago when I really wanted a way to push my honors geometry students into thinking originally while at the same time assessing their knowledge of using technology.  I did a presentation last year at the Anja S. Greer Conference on Math, Science and Technology and the audience loved it.  Basically, I give students an inquiry question (one that I attribute to my good friend Tom Reardon) that they have to work on with technology and then they have to come up with their own inquiry question (which is, of course, the fun part) and explore that with technology and/or any other methods they wish.  I have received some pretty awesome projects in the past two years and I don’t think I am going to be disappointed this year either.

The French Gardener Problem is famously used in my PBL courses at the MST Conference as well.  Everyone who has taken my course knows the fun and interesting conversations we have had about the many ways to solve it and the extensions that have been created by many of my friends – an ongoing conversation exists somewhere in the Blogosphere about the numerous solutions – In fact Tom sent me a link just last fall to a more technological solution at Chris Harrow’s blog. (We’re such geeks).  Great math people like Phillip Mallinson and Ron Lancaster have also been drawn in by the attractive guile of the The French Gardener Problem.  In this problem, the main question is what fraction of the area of the whole square is the octagon that is formed inside (what is the patio for the garden)?

So the other night, after we had worked on this question in class for a couple of days and the students had meet with me in order for me to approve their original inquiry question, a student stops by to discuss his question.  John starts off with, “I can’t think of anything really. What I had wanted to do, someone else already claimed.” (I’m not letting them do a question that someone else has already decided to look into.  So John sits in my study and thinks for a while. I told him that this part of the project was supposed to be the fun part.  I gave him some thoughts about extending some problems that he liked.  He said he had liked the French Garden Problem and thought it was really cool.  So I went back to some of my work and he started playing with GeoGebra.  Before I knew it he starts murmuring to himself, “Cool, cool….Cool! It’s an octagon too!”  I’m thinking to myself, what has he done now?  I go over to his computer and he’s created this diagram:

John's Original Inquiry Question
John’s Original Inquiry Question

I’m asking him, “What did you do? How did you get that?”  He says that he just started playing with the square and doing different things to it and ended up reflecting equilateral triangles into the square instead of connecting the vertices with the midpoints as in the original French Garden Problem.  Then he started seeing how much of the area this octagon was and it ended up that it was……you don’t think I’m going to tell you, do you?

Anyway, it just made my night, to see the difference in John when he came by and the by the time he left.  He was elated – like he had discovered the Pythagorean Theorem or something.  I just love this project and I would encourage anyone else to do the same thing.  Leave a comment if you end up doing it because I love to hear about any improvements I could make.

Having Students Be More Aware of their Contributions in PBL

One of the things I do at the middle of the term to have students reflect on the way that they talk about mathematics in class, is have them evaluate their work with my Student Self-Report on Class Contribution and I give them detailed feedback on their rankings of each type of question and what I think about their work so far.  But what I’ve found in the past is that it’s hard for them to remember specific examples of when they “helped to support the point by contributing evidence” or “raised a problem in another person’s solution” weeks or days after the fact.  Students have so much to think about during a class period that it really needs to be “in the moment” for them to be deliberately thinking about what they are doing and saying to each other.

So I had this idea that I would actually have them keep track of the types of questions and comments they made to each other over a five day period at the end of the term and then hand it in to me.  This way they would check off each category of question or comment at the moment when they did it.  So I made copies of this table of Student Analysis of Contribution and had them keep it on their desks while we were discussing topics or problems in class.  They had to have something to write with while they were talking and taking notes too.

Initially the students were very concerned about how they were going to tell the difference between each of the types of contributions.  But little by little, it became easier.  These categories were not arbitrary, they happened all the time, they just hadn’t really thought about it before.   For example, when a student presented a problem but had made an error and didn’t know it, another student usually “raised a complication in another person’s soltuion” or “pointed out an unspoken assumption or misunderstanding.”  These are important contributions that they were making every day about mathematics and are important critical thinking skills they didn’t even realize they were developing.  I just wanted to help them be more specific about realizing it.

The first day we did this I heard some students say something like, “Ooh, I just…(brief pause while looking at the table)… ‘started the group discussion on a rich, productive track by posing a detailed question.” with pride and excitement (and maybe a little sarcasm).  But after three days of doing it, and students seeing that they had places that they could check off, one student actually said, “I think that this table makes our conversations more interesting.”

Here are some samples of student feedback:

Quiet student who needs to work on all types of comments and questions

This first respondent is a student is very quiet, and she knows it.  She rarely speaks in class, but is actively listening.  I’ve spoken to her about why she does not ask questions or share her ideas in class and she says that she is afraid of being wrong in front of everyone.  My hope all year has been that seeing everyone else be wrong regularly would eventually show her how acceptable it was in class.  It was clear that using this table showed her that there are many different ways to contribute to class discussion.  When she presented her problems in class (which she will do when asked) she is very capable and knows that she is contributing evidence or examples, but she rarely questions others’ work.  I do remember the time when she “built on” what was said by asking a question about someone else’s solution.  She also sometimes asks for clarification of her own understanding, but I know that she’s capable of more.  I struggle with how to encourage her to get more out of class discussion, but at least now she knows how important a role she can play.

Outgoing student who lacks interaction from other kids

This is a really interesting student who is quite inquisitive and very comfortable with sharing his ideas.  When he has a question or comment, it’s very easy to get him to go to the board and naturally start writing another idea or possible solution method.  However, I noticed was missing in his table were checks in the two rows that had to do with “inviting others” into his thought process and also making comments on others’ solutions and ideas.  It made me wonder how much time in class he spends listening to other students talking or if he is just listening to his own ideas in his own mind.  I am working on trying to get him to collaborate more – with his creative mind it would behoove him to start interacting more with others.

Outgoing student who is unaware of his effect on others

This table belongs to a student who really has overestimated his contributions to class.  He definitely spends a lot of time talking (and hence I spend a lot of time managing his unrelated talking unfortunately) and thinks that talking – any kind of talking – is useful and contributing to class discussion.  One of the things I have talked to him about is the idea of active listening and how important not speaking can be.  He has not yet caught onto the idea of being respectful while listening, and still is considering his next talking move while others are trying to make their points.  It will be a difficult discussion, but a necessary one for this young man to grow in important ways.  At least I now have this chart to refer to when I have that discussion.

So was this exercise everything I had hoped?  Not really – but it definitely had some great highlights.  Class discussion was very exciting and interesting while students were aware and deliberate knowing the different types of ways in which they could contribute.  Knowing that they had to hand this table in to me in 5 days was putting the onus on them to show that they had or had not fulfilled what I had observed of them in class.  I do believe they learned a lot about what they were capable of.  I do believe I would do it again.

PBL & James and the Giant Peach: Try looking at it a different way

2 Comments

James Henry Trotter: “When I had a problem, my mum and dad would tell me to look at it another way.” (Roald Dahl)

I’ve always thought that PBL fostered creative problem solving as opposed to memorization of pneumonic devices.  One of my students today proved me right when I gave a “quick quiz” on the use of the idea of tangent.  We had discussed tangent in class for only two days and in two ways – one as a slope of a line with a given angle and from that idea we discussed how it could be interpreted as the ratio of the sides of a right triangle (if you put a right triangle under the line).

Of course, during this conversation some student who had studies the ever popular SOHCAHTOA before mentioned this in class and told everyone that they had just memorized this and that’s how they knew it.  I said that’s fine but I’d like them to try to think about the context of the problems and see if this helps make any sense of it for them.

So today on the quiz one student was attempting this problem – very basic, very procedural, not at all something that I would call atypical of a textbook-like problem on tangent.

A bird is sitting on top of the Main School Building and looks down at the end of the baseball field with an angle of depression of 4 degrees.  If the MSB is 87 feet tall, how far away is the end of the baseball field?

So the student attempts to create a ratio with the sides of the triangle and even sets it up correctly.  However, because she does the algebra incorrectly, she gets an answer that is extremely small 8.037 x 10^-4.  In fact, during the quiz, she calls me over and asks what it means, she doesn’t remember scientific notation and starts getting all anxious because we didn’t do anything like this in the problems in the previous two days?  How can the answer be that small?  I said well, you better go back and think of something else.

In most classes, a student in this situation might stress out, try to do the problem over again with the limited perspective of “TOA” or of just viewing the right triangle in one way.  However, because this students had also learned other students’ perspectives of tangent as slope of a line what this girl did at this point was to see it from a different way.  Interestingly, this is what she did.  In an alternative, albeit confusing way of writing the equation of the x-axis, she wrote y=0x to represent the ground.  Then she found the tangent of 4 degrees and used that as the slope of a line.  She put the bird at the point (0,87)

She writes the equation y= – (tan4)x + 87 and explains that this is the equation of a line that makes a 4 degree angle with the x axis and has a y-intercept of 87.  Then she realizes that if she finds the intersection of that line and the x-axis, she would find how far the building is from the baseball field.  This is what she does and uses her graphing calculator to get the right answer.

When she hands in this quiz to me, I half expected that tiny little answer as her distance to the baseball field.  But what I got was an amazingly inventive solution and a correct answer.  With a problem that didn’t make sense, she looked at it a different way and ended up getting the right answer.  It was amazing what changing your perspective could do and this was great evidence that even under pressure, the habits of creativity and connection were paying off.