Category: Articles

Reducing Cognitive Load in PBL

2 Comments

One of the things that I have been thinking about for a very long time is the idea of those who oppose PBL.  Namely those who prescribe to behaviorist and cognitive scientist theories of learning, which I know a great deal about because of my doctoral work.  So many teachers, parents and others have asked me about this over the past 25 years that you’d think I would have an answer.  I know I have thoughts but I do want to do more research in this area.

I do not pay lip-service to the ideas of cognitive load theory for sure and definitely respect those who follow these ideas.  I do think there is a place for thinking about this theory in PBL, but not an argument for why NOT to do it.  At its heart though, I believe the learning outcomes that are important in the different types of theory (CLT vs. constructivist learning for example) is what ends up differentiating them and also the way the knowledge is constructed.  I do believe in the importance of reducing the Cognitive Load for students so that their long-term memory can be triggered and practiced.

So I do believe there is a place for this in PBL – it just hasn’t been discussed a great deal.  There is always this us vs. them notion that one is right and the other is wrong – it comes from very strong belief systems and I totally understand where they are coming from.  However, if PBL is done well in a scaffolded, structured way, I believe that you can both reduce cognitive load and also ask students to think creatively.

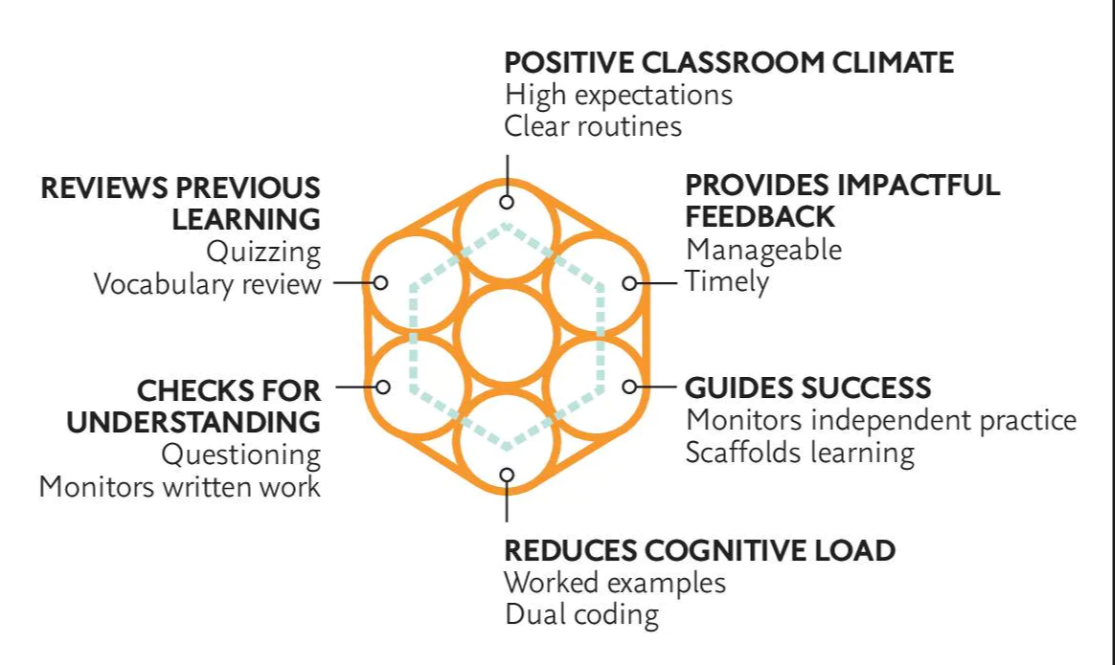
Here is an image I saw from an article in the Guardian recently entitled Teachers: Your Guide to Learning Strategies that Really Work by Carl Hendrick. This graphic is describing the six ways to make your classroom best-ready for learning.

 

Positive Class room climate

 

When I was looking at this, the first thing I thought of is “This is my PBL classroom.”  However, I could tell there would have to be some discussion of the “reducing cognitive load” part.  All the other aspects, I believe you can find in some other blogpost of mine somewhere.  In a PBL classroom, the way that students get timely feedback is in so many ways (see my rubrics, journals, etc.).  The nightly homework is the scaffolding of learning and monitoring of independent practice – again when done well.  I won’t go through every one of these, but would love your takes (in the comments below) on each of them.

So then, how can we talk about reducing cognitive load in PBL – where is lecture and worked problems that the teacher does?  I would argue that the cognitive load is reduced by the scaffolding of the problems in the curriculum.  In other words, by triggering students’ prior knowledge the cognitive load is reduced in such a way that they are remembering something they have learned from the past, and then being asked to look at something new.  The “something new” goes through many phases of problems – concrete, multiple representations, all the way through to abstract – in order to slightly build up the cognitive load.  Again, this is all if it is done well and very deliberately with the idea of not to overload students’ thinking but to help to build the schemas that are needed for constructing knowledge both individually and socially.

The problems are worked by the students, yes – I will give you that.  But it is the teacher’s responsibility to make sure that the steps are correct, students get feedback on their thoughts and ideas, that on the board at the end of the discussion is a correct solution and so much more.  What this type of teaching does, in my view is both reduce cognitive load to a point, yet also allow students to gain agency and ownership of the material through their prior knowledge and experience.

Something else that Mr. Hendrick says in his article is:

“Getting students to a place where they can work independently is a hugely desired outcome, but perhaps not the best vehicle to get there. Providing worked examples and scaffolding in the short-term is a vital part of enabling students to succeed in the long-term.”

And I would ask, what does students’ success mean in this framework?  Some studies have shown that worked examples are beneficial in only some cases for student learning.  Others have shown that students that are taught with worked examples out-perform those who received individual instruction.  I could go on and on with the studies contradicting each other.  But what if they weren’t in contradiction?  What if there was a way that they could work together – both reducing cognitive load and also giving students agency and voice in the classroom?  Allowing students the freedom to become independent problem solvers but also scaffolding the learning in such a way that their cognition was not overloaded?  Maybe I’m an optimist, but I do believe there is a way to do both.

I’ve looked at life from both sides now…

4 Comments

This past July, I spent a few days at the MAA Mathfest in Chicago for the first time. The main reason I went was because the Academy of Inquiry-Based Learning was having a Conference within the Mathfest with the theme of “Diversity in IBL.”  IBL is generally what college faculty call the type of teaching and learning that many of us at the secondary level has been calling PBL for years.  I was so interested to hear many mathematics professors talking about the struggles of writing curriculum, dealing with facilitating discussion, using writing – all of the same parts of this type of teaching that we may have been talking about for so any years.  I highly suggest that we could benefit from talking to each other.  If you would like to get involved with this movement, Stan Yoshinobu, the Director of the Academy of Inquiry-Based Learning, has put forth some challenges for his community.  Check them out.

One of the most interesting talks that I attended was by a professor from Denison University, Lew Ludwig, titled, “Applying Cognitive Psychology to the Mathematics Classroom.”  As a devout social constructivist, I generally like going to talks where I can learn more about other views of education.  Seeing both sides definitely helps me understand many of the views of my colleagues and see if evidence supports my own perspective. Ludwig had published a review of another article that was titled, “Inexpensive techniques to improve education:  Applying cognitive psychology to enhance educational practice”(Roediger and Pye, 2012).

Basically, the presentation summarized three simple techniques that cognitive psychology had evidence helped student learning. The three techniques were called

  1. The distribution and interleaving of material and practice during learning.
  2. Frequent assessment of learning (test-enhanced learning, continual assessment)
  3. Explanatory questioning (elaborative interrogation and self explanation; having students ask themselves questions and provide answers or to explain to themselves why certain points are true).

In the original article, the authors write:

“Repetition of information improves learning and memory. No
surprise there. However, how information is repeated determines
the amount of improvement. If information is repeated back to back
(massed or blocked presentation), it is often learned quickly but
not very securely (i.e., the knowledge fades fast). If information is
repeated in a distributed fashion or spaced over time, it is learned
more slowly but is retained for much longer”

When this was reported, I was first in shock.  I couldn’t believe I was hearing something in a presentation about Cognitive Psychology that was actually supported by the definition of PBL that I use.  The curriculum I use takes the idea of looking at topics and teaching them over a longer time span, but distributed among other topics.  I have called this decompartmentalization of topics, which helps students see the connectedness of mathematics.

The second idea, consistent assessment, is based on the concept that testing is not really a great measure of how much a student has learned, but it actually solidifies the learning that has occurred.  So three groups of students were given different ways of learning by reading a passage of information. The first group read a passage four times. The second group read the passage three times and had test.  The third group read the passage once and was tested three times.  Their performances on tests on the information in the passage 5 minutes later and then one week later.

Diagram of retention testing research
from Roediger & Pye (2012) p.245

So if we connect the idea that testing is not the best method of seeing how much students have learned and the fact that consistent assessment actually helps students retain their knowledge, what I do in my PBL classrooms, is not only “test” but do all sort of forms of assessment (writing, oral assessment, hand-in homework with feedback, labs, quizzes, problem sets, self-assessment, etc.) alternately throughout the term.  There is probably not a week where students are not assessed in at least 2 ways. I feel that this has led students to have good retention of material and the assessments are strong measurements of their learning.

The last one was the one I was most excited to hear about – explanatory questioning.  This seemed to give students so much more responsibility for their own learning than traditional cognitive psychology as I had understood it.  The authors of this study claim that explanatory questioning can be broken in to two areas:

Elaborative Interrogation – students generating plausible explanations to statements while they are studying and learning.  This speaks directly to the idea of mathematical discussion and how students generate explanations when they ask themselves “why?”

Self-Explanation – students monitoring their learning and describing, either aloud or silently some features of their learning.  This idea can be found all over the PBL classroom but in mine, it’s generally found most in metacognitive journaling where students use self-explanation the most.

“Obviously, the elaborative interrogation and self explanation are related because both strategies encourage or even require students to be active learners, explaining the information to themselves (perhaps rephrasing in language they understand better) or asking themselves why the information is true.”

I honestly couldn’t believe what I was reading – this is an article on educational methods based on cognitive psychology that is suggesting that we require students to be active learners and “explain the infomation to themselves”?  This is lunacy.  I have been teaching for 25 years where students have been complaining to their parents that they have had to explain things to themselves – who would’ve known that I was applying cognitive psychology?

My guess is that these ideas are only enhanced by the social aspect of the classroom and other constructivist ideas – clearly the constructivitst classroom in enhanced by or agrees with some of these cognitive psychology methods as well.  Listening to both sides of the theories is actually helpful and I’m seriously going to continue doing this! Although I never thought that there might be strong connections between cognitive psychology theories and PBL, I do know that it’s life’s illusions I recall and I “really don’t know life, at all.”

Why PBL Works for Introverts

My school year is underway and as September just flew by, I have been completely overwhelmed by work – of course.  I am undertaking a new assessment method with a colleague of “feedback first and then grades” (blogpost to come when I give back the first set next week) but for now I wanted to comment on an article I just read this morning entitled “When Schools Overlook Introverts” that was posted on the Atlantic’s website.  This is a very thoughtfully written piece by Michael Godsey that is discussing how so much education is based on the idea of social constructivism which might be hard on those of us who are built to work best in “quieter, low-key environments.” This implies that the environments of collaboration and working with others are always loud, chaotic and multi-faceted.

And you know, sometimes it is.  Classrooms where kids are all at the board or working with technology can be messy.  Everyone’s talking at once, kids are calling me over and asking questions out loud (often the same questions 5 times in a row) and they are seeing themselves as the center of attention.  Once they understand, they move on and help their partner move on.  In my classroom, they take pictures with their iPads, record work in Notability or use GeoGebra to get a different perspective – either algebraic or geometric.  This can be quite chaotic.

However, most of the time in the PBL classroom.  everyone is required to sit quietly and listen to one student describe their thought process.  They need to learn to sit patiently while another student works though confusion and misunderstanding and ask questions of the presenter.  An introvert has a great deal of time of quiet to themselves being inside their head while the presenter is discussing his or her own grappling with a problem from the night before and the introvert can sit there and think, “Huh, that’s not what I did.  Should I say something and comment, or just accept that as the right answer?” The introvert grapples with different demons in the PBL classroom if they are a strong mathematics student in many ways because they might feel confident in the material but not confident that people care about their ideas.  Who knows?  It depends on their personality.

The introvert also has the opportunity to write journal entries for me and also to write bi-weekly learning reflections about what his or her learning successes were for the week.  This year I have a student with a speech impediment who was upfront with me about it at the beginning of the year.  This student has quickly become one of my best communicators because he realized how much I value what he has to say and that I would be patient and so would the rest of the class.  If he can’t say what he needs to say at the moment he wants to in class, he will always have an opportunity each week to do it.

I am very clear on my classroom contribution Assessment Rubrics that the grade does not depend on quantity of contribution, but quality.  Introverts should contribute because they have something important to add, an excellent question to make a clarifying point or something that will add depth to the conversation – never just to add to their grade. They can look at what they need to improve on by using my Student Analysis of Contribution which I will be doing next week – it’s about that time of the term.

I believe that although PBL strives to allow for all voices to be heard (both extrovert and introvert) it is the teacher that makes or breaks the classroom culture.  We need to be continually checking and rechecking the barometer of communication and tone of the class to be sure to all students are feeling heard. So that as Godsey says at the end of his article, the kids can learn with others and not by the “hell of other people.”

 

Why Can’t We All Just Get Along?: Some Inquiry Math Classes are not Content-less

Maybe it’s just how I am, or maybe I’m just always worried about what people are going to say about me, but I am hesitant to criticize other teachers publicly in the blogosphere. I’ve always felt this camaraderie with others once I’ve learned they were a teacher even if we are very different from each other – different disciplines, different pedagogical styles, different countries – there are still fundamental commonalities that even public and private school teachers have.

I just finished reading a KQED blogpost entitled “Do You Have the Personality to be an Inquiry-Based Teacher?” that sort of summarizes the theoretical qualities that the author feels a teacher who would teach with IBL would need to exhibit in order to successfully run a classroom. It’s kind of interesting – I’m not sure I agree with it, but respect the author for putting his ideas out there. I’ve been an inquiry/problem-based teacher for almost 20 years and I don’t think I exhibit all of the qualities listed, so I’m not sure it’s quite true.

Anyway, that’s not the point – at the end of the blogpost there are about 11 comments from people who are educators and many of them are quite negative and even degrading to the author:

“I earned a Ph.D. in Educational Psychology, but phrases like this one still baffle me: “…the divide between a transmission model and an inquiry model…” ”

“First, we need to make sure that we have at least a rudimentary understanding of the language in which we will be teaching. Second, we need to make sure we can write.”

“That is what’s wrong with you teachers.You want to do it your way.”

“Some of us have been doing this for decades, where were you?”

Whoa, Whoa, Whoa…cowboys…hold your horses. This guy is just writing an essay about something he believes in. What kind of role model are we being for our students if this is how we are reacting to something we don’t agree with? What happened to civil communication? I totally agree that people are allowed to comment and voice their opinions on someone else’s opinion, but there has to be a way to do it with respect and decency.

So I am going to try to model what I would like to see as a response to something I actually do disagree with. Here is a blogpost by a very respectable Professor in Canada, who I have to be totally honest, I do not know at all. I tried to learn as much as possible about him before writing a response to his blogpost in order not to make any assumptions about him (and not make a fool of myself in doing so), so I may be wrong about some of this information because I garnered it from different websites. It seems he is a research mathematician who is currently studying to get a teaching degree, but who lectures for mathematics courses at the college level. I cannot ascertain if he has any experience teaching at lower levels (like elementary or secondary). From his blogpost it does seem like he takes pride in the amount of background research he does, which again is very respectable and I appreciate in bloggers. He seems to care a lot about student learning and from his opinions on his blog he seems to lean towards being a behaviorist and cognitive theorist in terms of learning theories.

His latest blogpost is titled “The Content-less Curriculum” and it is a critique of the movement towards 21st Century skills being a part of the mathematics classroom. It does sound like Prof. Penfound is implying that with the inclusion of “soft skills” of collaboration, critiquing others work, problem solving,communication, etc. (i.e. the MPS for the CCSS) there must be a loss of mathematical content. In fact, he says that

“there must be a trade-off for the inclusion of “soft skills” activities into an already packed curriculum. So what gets removed from the curriculum then? Content knowledge.”

I would respectfully, but wholeheartedly disagree with this. By teaching with the PBL curriculum that I use, I have all of the college prep geometry curriculum I desire and I also concurrently am assessing and teaching the skills of problem solving and the so-called “soft skills” that he is implying are an add-on. I still give quick quizzes to make sure that students are up on their basic skills that are so important for basic problem solving (or else they wouldn’t be able to do the open-ended problems they are given).  The mathematics that students leave my courses having experienced is rich and leaves an impression on the way they think.

Making blanket statements about teachers implying that we all make choices that are not based in research or good practice is just not true. I actually invite you Prof. Penfound to come visit my classes and see my IBL/PBL classes in practice and let me know what you think of your opinions of the rigor of the mathematics that is discussed. Although we are most likely at different ends of the spectrum in terms of learning theories, I do believe that students have different needs and try to work with kids’ learning needs individually. However, I do believe as @danieldmccabe does that there are going to be new outcomes required of our ever-growing diverse body of graduates in the near future (or even present). I also have to say that I have thought rather thoroughly about the implementation of a teaching program which includes “soft skills” and even wrote a dissertation on it.

It is possible to balance content and practice skills and it is what I and many other classroom practitioners strive for. I do not deny that there are some practitioners out there that are confused about what problem-based and project-based learning outcomes should be especially with regard to secondary mathematics, but that is a subject for another blogpost.  The balance between content and practice skills we should strive for does not mean that one is more important or less important and in fact they both need to be assessed with the ultimate goal being to create independent problem solvers. From my experience this does not necessarily happen in a classroom where the educator does not take into consideration the so-called “soft skills.” But that statement is, of course, based on my 25 years of anecdotal classroom experience.

 

What does “making students metacognitive” mean? – answering “why should someone learn?” in Math

So I recently tweeted a nice article that I read that discussed “12 Questions to Help Students See Themselves as Thinkers” in the classroom (not specifically the math classroom

 

and appropriately, Anna Blinstein tweeted in response:

 

So I thought I needed to respond in a post that spoke to this question. First of all, I should state the caveat that even when I am in a more “standard” classroom (i.e. not a PBL classroom) – which happened to me last year – I try as much as possible to keep my pedagogy consistent with my values of PBL which include

1) valuing student voice
2) connecting the curriculum
3) dissolving the authoritative hierarchy of the classroom
4) creating ownership of the material for students

I find that helping students to be metacognitive helps with all of this. An important aside her is also Muller’s definition of 21st century learning* which is much more than that 20th century learning and education that often comes with direct instruction in the mathematics classroom (not always).I think it’s important to note that the more fluid concept of knowledge that is ubiquitous with technology today and is no longer static in textbooks or delivered by teachers.  Students can go find out how to do anything (procedurally) nowadays, but it is the understanding of it that is more important and the true mathematical learning and sense making.

Anyway, I think I would write way too much if I responded to every one of the questions, but how would I use these questions in my direct instruction class that I taught last year?  What I tried to do was introduce a topic with some problems (and then we would do some practice with problems from the textbook so I could keep up with where my colleague was in the material).  Well, this course was Algebra II, which often referred to prior knowledge that always reminded students of something they had studied before.  I let them use computers to look things up on the internet and use the technology at hand, GeoGebra, Graphing Calculators, each other to ask questions about the functions we were studying.  They could look up topics like domain, range, asymptotes (why would there be an asymptote on a rational function)…but then the bigger questions like “what am I curious about?” had more to do with how did those asymptotes occur, what made vertical vs. horizontal asymptotes and then I would have them do journal entries about them (see my blogposts on metacognitive journaling – journaling and resilience, using journal writing, page on metacognitive journaling).

The more “big picture” questions like “Why learn?” and “What does one *do* with knowledge?” I find easier to deal with because the students ask those.  I think that all teachers find their own ways to deal with them, but I enjoy doing is asking students about a tough question they are dealing with in their life – I use the example of whether or not I should continue working when I had my two kids.  Was keeping my job worth it financially over the cost of daycare? and of course I had to weight my emotional state when I wasn’t working – this is why I enjoy learning and what I do with my knowledge.  When kids see that there’s more to do with functions than just points on a grid, it becomes so much clearer for them – but you know that!

What I really like about Dr. Muller’s list is that he lays out some nice deliberate ways in which we as math teachers can get students to think more clearly and reflectively about mathematics as a purposeful process as opposed to a just procedures that they can learn by just watching a Kahn Academy video.

 

*”Learning – here defined as the overall effect of incrementally acquiring, synthesizing, and applying information – changes beliefs. Awareness leads to thoughts, thoughts lead to emotions, and emotions lead to behavior. Learning, therefore, results in both personal and social change through self-knowledge and healthy interdependence.” Muller http://tutoringtoexcellence.blogspot.com/2014/08/helping-students-see-themselves-as.html

Looking for the Teacher of Grit

I’m in the middle of working on organizing my courses for the Exeter conference in about a week and something I’m really struggling with is trying to articulate to teachers how they can impart to their students this idea of grit in the PBL classroom.  So I started doing a little research online (besides looking through all of the books I have read on the subject).  I took Angela Duckworth’s Grit Test at her lab’s website (got a 3.63 grit score- grittier than 60% of other U.S. citizen’s my age…hmmm).  Then I started reading some blog posts of other PBL teachers and writers like here on the MAA’s blog which is trying to encourage math students to tinker with problems or here which is more of an all-purpose index of resources to teaching grit. There was this wonderful video of a teacher in NH who created a neat grit curriculum for her 5th grade class (with Angela Duckworth too)

John Larmer of the Buck Institute wrote a really nice blog entry on how project-based learning fosters grit in students. I even found a nice video of Po Bronson, author of Nurture Shock (the book about how parents have failed kids because we don’t let them fail).  This is a short video of how Mr. Bronson believes we should be allowing kids to fail these days.

He says (in so many words) that if kids grow up without learning how to fail, they will become risk-averse.  This is what I am finding in my classroom at times.  The risk-averse kid combined with the fixed mindset kid, combined with the “I-have-to-get-into-college-and-make-my-parents-happy” kid makes the PBL classroom very difficult when you are trying to get them to take risks and be creative.  Add that to the classroom culture that they have been used to for the first 9 years of their education in the U.S. and sadly, it makes for a tough place to foster the teaching of grit.

In fact, on my most recent course evaluations I asked students what they found most challenging about the class and the two pieces that tied for first place were journal writing and

“having to be vulnerable and make mistakes in front of my peers.”

I so want to change that and I always thought that I created a classroom atmosphere where students were comfortable.  I did all of these things that the professionals are suggesting on these websites:

1. modeling risk-taking and making mistakes myself
2. talking about growth mindset regularly
3. ask them to write about positive experiences when they are proud of themselves
4. using class contribution feedback forms (self-report and analysis of class contribution sheets)
5. using strategies where students think of a wrong way before we talk about the correct solution method together.

But somehow, even at the end of the year, their fear of being wrong in front of each other (and me, some commented) is still predominantly what they say challenged them.  So I would say to Po Bronson, where is the teacher of Grit?  What is the secret?  How do I make it so?  Is there a time when it’s too late for some kids?  Most of what I’ve seen on the internet is teaching grit to elementary school children – does the fact that I am teaching high school kids make it any harder?

I finally found this great Prezi created by a teacher named Kristen Goulet which, I know, is geared towards elementary school kids, but I think I could find a way to direct it towards older students.  The idea of having them ask themselves whether their self-talk is “because of me” or “because of other” and whether it is “permanent (i.e. fixed mindset)” or “temporary (i.e. growth mindset)” definitely would help them realize how much of the way the deal with adversity is flexible.  It also helps with seeing how to have a more realistic and optimistic view of a certain situation (and is kind of hard to argue with).

So, I’m still in search for the best practices to teach grit (and apparently so is Angela Duckworth – she admits this in her TED talk), but now I know that it is way more complex than just following a certain number of steps – it has so much more to do with a student’s socio-emotional state of mind. Vicki Zakrzewski’s article “What’s wrong with Grit?” is probably the closest I got to agreeing with someone’s assessment of grit and how to teach it.  I know that I am really good at letting kids know what is important to me and doing that modeling that is important as well.  Undoing what has happened to them before they got to me is a tall order, but I’m not going to stop trying.

Does journaling in PBL promote resilience?

So I just read a great blogpost by Kevin Washburn of Clerestory Learning entitled “Teaching Resilience: Reflection” and it immediately made me think of the Metacognitive Journaling that I have students do in my classes.  I never really thought of it the way that Washburn was describing the reflection and the conseuences of reflection, but it’s pretty clear that if his theory is right, that a by-product of journaling could easily be resilience.

Initially, Washburn talks about the process of reflection – right out of Dewey in a way – but he narrows it down to the steps in the process (but does mention the word metacognition – thinking about thinking).  He defines reflection as “the ability to monitor one’s own thinking” which is what I tell my students the goal of writing in the journal is.  Hopefully, by the end of the year, they will have realized the way they look at problems and how they’ve had those “lightbulb” or “ah-ha” moments enough after writing about them, that when they come across a new problem, the process of being aware of their own problem solving is much more natural.

Washburn’s three steps are as follows:

1. Asking yourself “what am I thinking now?”
2.  What can I tell myself to redirect my thinking?
3.  What can I do differently?

Most students in the beginning of the year, can easily do the first step – it begins very simply as them just redoing their work (usually the correct way), which can, unfortunately, just be them rewriting their notes from class.  However, this has to be a place to start for them.  This is where teacher feedback is key.  I spend most of my time writing comments like ,”I want to hear what *you* did initially” or “is this what your first thoughts were?”  It’s really hard for students to believe that you want a record of what they did wrong.

But somewhere during the year, kids grow in their understanding of WHY their initial idea didn’t work.  This seems to be the most important part of the reflection.  That gained insight gives them not only deeper understanding, but a sense of ownership and responsibility for their own learning that can’t be had with just seeing how many points they got off from the problem on an assessment.

I’ve written about this a few times (see other blog entries) and have seen kids grow in their understanding during the 18 years that I’ve been using journals in my classroom.  However, what Washburn helped me see is how this skill of recognizing how their initial erroneous thinking has actually made them a stronger, more confident thinker.  This is an amazing gift.  As Washburn says,

“In life and in the classroom, the one doing the thinking is doing the learning. When thinking ceases and self-defeating messages crescendo, we can guide students to healthier states of mind and, in the process, equip them to make such cognitive turns on their own.”

So  great!

Does PBL teach Resilience?

I just read a great blogpost by a business writer, Gwen Moran, entitled, “SIx Habits of Resilient People.” When I think of people that I admire in my life for their resilience there was usually some circumstance in their life that led them to learn the quality of resilience because they had to. Even the examples that the author uses in this blogpost – being diagnosed with breast cancer, almost being murdered by a mugger, the inability to find a job – these tragedies that people have had to deal with can be turned into positive experiences by seeing them as ways in which we can learn and grow and find strength within ourselves.

But wouldn’t it be great if it didn’t take a negative experience like that to teach us how to be resilient? What if the small things that we did every day slowly taught us resilience instead of one huge experience that we had no choice but to face? Having to deal with small, undesirable circumstances on a daily basis, with the help and support of a caring learning community would be much more preferable, in my opinion, than surviving a mugging. (Not that one is more valuable than the other). But I just wonder – and I’m truly ruminating here, I have no idea – if it is possible to simulate the same type of learning experience on a slower, deeper scale by asking students to learn in a way that they might not like, that might make them uncomfortable, that asks more of them, on a regular basis.

I think you know what I’m getting at. Does PBL actually teach resilience (while also teaching so much more)? In my experience teaching with PBL the feedback I’ve received from students has been overwhelmingly positive in the end. But initially the comments are like this:

“This is so much harder.”
“Why don’t you just tell us what we need to know.”
“I need more practice of the same problems.”
“This type of learning just doesn’t work for me.”

Having students face learning in an uncomfortable atmosphere and face what is hard and unknown is difficult. Thinking for themselves and working together to find answers to problems that they pose as well as their peers pose is very different and unfamiliar. But does it teach the habits that Gwen Moran claim create resilient people? Let’s see. She claims that resilient people….

1.Build relationships – I think I can speak to this one with some expertise and say that at least if the PBL classroom is run with a relational pedagogy then it is very true that PBL teaches to build relationship. My dissertation research concluded nothing less. In discussing and sharing your ideas, it is almost impossible not to – you need relational trust and authority in order to share your knowledge with your classmates and teacher and this will only grow the more the system works for each student.

2. Reframe past hurts. – If we assume that real-life “hurts” are analogous to classroom mistakes, then I would say most definitely. PBL teaches you to reframe your mistakes. PBL is a constant cycle of attempting a problem->observing the flaw in your solution ->trying something else and starting all over again. This process of “reframing” the original method is the means by which students learn the the PBL classroom.

3. Accept failure – This may be the #1 thing that PBL teaches. I am constantly telling my students about how great it is to be wrong and make mistakes. You cannot have success without failing in this class. In fact, it is an essential part of learning. However, students in the US have been conditioned not to fail, so that reconditioning takes a very long time and is a difficult process.

4. Have multiple identities – In a traditional classroom, certain students fulfill  certain roles – there’s the class clown, the teacher’s pet, the “Hermione Grainger” who is constantly answering the teacher’s questions, etc. But what I’ve found happens in the PBL classroom is that even the student who finds him/herself always answering questions, will also find him/herself learning something from the person s/he thought didn’t know anything the next day. Those roles get broken down because the authority that once belonged only to certain people in the room has been dissolved and the assumption is that all voices have authority. All ideas are heard and discussed. PBL definitely teaches a student to have multiple identities while also teaching them a lot about themselves, and possibly humility, if done right.

5. Practice forgiveness – This might take some reinterpreting in terms of learning, but I do believe there are lessons of forgiveness in the PBL classroom. Students who expect themselves to learn everything the first time and when they don’t, feel stupid, need to forgive themselves and realize that learning is an ongoing process. Learning takes time and maybe needs more than one experience with a topic to see what the deeper meanings and understandings really are. Since PBL is not just a repetitive, rote teaching method, students need to learn how to be patient and forgiving of their own weaknesses as a learner and take time to see themselves as big picture learners.

6. Have a sense of purpose – This habit is about “big picture” purpose and looking at a plan. From the research that I did, I also found that PBL brings together many topics in mathematics, allowing students to see the “big picture” connections between topics much better than traditional teaching does. The decompartmentalization that occurs (as opposed to compartmentalizing topics into chapters in a textbook) is confusing at first because they are not used to it, but eventually students see how topics thread together. Just the other day in my geometry class we were doing a problem where they were asking to find as many points as possible that were 3 units away from (5,4) on the coordinate plane. A student in the class asked, “is this how we are going to get into circles?” The whole class was like “Oh my gosh, it is, isn’t it?” Bam, sense of purpose.

All in all, I feel that PBL meets Moran’s criteria of “resilience characteristics” in ways in which it allows students to practice these habits on a regular basis.  So not only does PBL help students learn collaboration, communication and creativity, but perhaps they will see the benefits over time in learning how to move forward from a setback – just a little.

 

Minimizing Shame in the PBL Classroom…and maybe Daring Greatly?

I recently read a blogpost by one of my favorite authors, Brene Brown, of TED talk fame, and the author of a great book about vulnerability called Daring Greatly.  In her blogpost Brene wrote about some reactions to a comment she made on Oprah Winfrey’s Super Soul Sunday show where she talked about shame in schools about which she received a great deal of criticism in the blogosphere and on twitter.

I kept reading as I was shocked that anyone would be offended by anything that Brene Brown could say – especially teachers.  She has always been extremely inspiring and very supportive of teachers – as a teacher herself, her book, Daring Greatly, has a whole chapter on how schools can support a community to come together around vulnerability and become closer and foster creativity and innovation in this way.

However, she talks about the research that she has done about learning and teaching.  She says,

“As a researcher, I do believe that shame is present in every school and in every classroom. As long as people are hardwired for connection, the fear of disconnection (aka shame) will always be a reality. ..Based on my work, I do believe that shame is still one of the most popular classroom management tools.”

Think about it.  When you talk to adults about their memories of school, and specifically math classrooms, many people will tell stories of being embarrassed or humiliated about getting something wrong, about feeling less than adequate or unworthy of being in the class they were in.  Even if the teacher was not doing anything deliberate, if a student has the courage to answer a teacher initiated question and get it wrong, the response that is given can make or break their self-worth that day.

I’ve been giving this a lot of thought in the context of the PBL Classroom – How are we supposed to be teaching students how to take risks and not be afraid to be wrong and make mistakes in their learning if they have this fear of shame that is so deeply entrenched in our culture?  Especially in mathematics classrooms, how are we supposed to undo so many negative experiences that may have affected a student’s ability to allow themselves to be vulnerable and learn in this way?

PBL relies on the fact that a student is willing and able to make connections and conjecture regularly – numerous times in a class and on their own during “homework” time.  Being wrong and uncertain is really the norm and not the anomaly in this classroom.  As October rolls around and I hear more from students (and parents) about the discomfort they are feeling, I really do understand how different this is for everyone.  However, I do think we need to rely on the fact that students can be resilient and strong when pushed to try new things and to learn in a way that is good for them.  It is just that resilience that will make them better leaders, learners and more creative in the work force later on in life.

In talking to some students recently, I asked them where they thought they would learn more, in a classroom where it was laid out for them what they had to do or where they had to make choices about methods and sometimes it would be unclear.  I could tell that one girl was really struggling with that question.  She knew that it would be easier in the other classroom, but also knew that she would learn more and wanted to stay where her learning would be more effective.

What can I do to help this process go more smoothly?  Make sure that they know that I am working hard NOT to use shame as a classroom management tool.  That I am sincerely interested in the mistakes that they are making and how it is helping their learning.  I want them to grow from their errors and misconceptions and find ways to use those to their advantage.  I want to add to their self-worth not only as a math student, but as a problem solver in every way.

As Brene Brown says:

“I don’t believe shame-free exists but I do believe shame-resilience exists and that there are teachers creating worthiness-validating, daring classrooms every single today.”

I can be truly aware of the language that I use and the questions that I ask in order to make sure that everyone’s voice is heard and that my students know that I want to hear their ideas.  It’s really the only way to get them to Dare Greatly!

PS – Check out the wonderful quote by Teddy Roosevelt that I use in my PBL classes about Daring Greatly that Brene Brown used for the title of her book.