On an assessment students did for me today I gave this question:

An aging father left a triangular plot of land to his two children. When the children saw how the land was to be divided in two parts (Triangle ADC and Triangle BDC), one child felt that the division of the land was not fair, while the other was fine with it. What do you think and why? Support your justification with mathematical evidence.

 So this student had a hard time with this question. Since there was no height given and the bases were different, she was unable to think about how to compare the areas. She was however able to say that it would be a fair split if the areas were the same. So since I am doing this work this year with giving feedback first and then grades (see past blogpost “Why teachers don’t give feedback before grades and why they should”) I wrote this feedback on the problem set:  I am trying to get her to remember a problem we did in class where there was a similar problem we did with an acute triangle and obtuse triangle that shared the same height:

The area of the shaded triangle is 15. Find the area of the unshaded triangle.

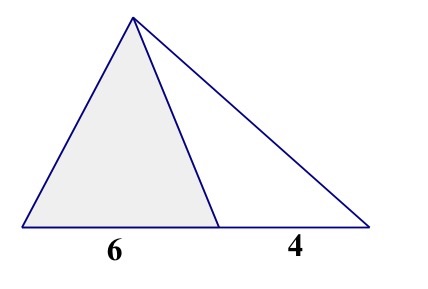
This idea of where the height of obtuse triangles are is a really tough one for some geometry students. But more than that the idea of sharing a height and what effect that has on the area is also difficult.

We will see tomorrow if this student is able to take my feedback and see what whether the division of the land is fair.

By the way, here’s a response that another student had:

Just in case you can’t read it:

“Because the height is the same, it’s the ratio of the bases that would determine which child would get the most land. I think the division of land was not fair, because the heights are the same so therefore the bases are determining the area of the plot. If x=5 then child one would get A=20, child 2 would get 12.5 and that makes the original plot of land 37.5. This means child 2 has a third of the land (12.5:25) (part:part) and half of child 1’s) Even without x=5, the child 2 would only get a third of the land.”

We’ll see what happens!